Fonctions logarithmes - T S
Classe:
Terminale
I Définition et propriétés
I.1 Définition
On appelle fonction logarithmique népérien la fonction notée $\ln$ qui est définie sur $]0\;;\ +\infty[$ et qui vérifie $(\ln x)'=\dfrac{1}{x}\ $ et $\ \ln 1=0$
$$\begin{array}{rcl}\ln\ :\ ]0\;;\ +\infty[&\longrightarrow&\mathbb{R}\\ x&\longmapsto&\ln x \end{array}$$
Remarques
Soit $u$ une fonction définie sur un intervalle $I$ de $\mathbb{R}$ alors :
$\centerdot\ \ln u$ existe si $u>0$
$\centerdot\ \ln|u|$ existe si $u\neq 0$
$\centerdot\ \ln u^{2}$ existe si $u\neq 0$
Exercice d'application
Déterminer les ensembles de définition des fonctions suivantes :
$f_{1}(x)=\ln 2x\;,\quad f_{2}(x)=\ln(4x+3)\;,\quad f_{3}(x)=\ln|x|$
$f_{4}(x)=\ln\left|\dfrac{x+3}{x-2}\right|\;,\quad f_{5}(x)=\ln(x^{2}-4x+3)$
$f_{6}(x)=\ln(9-x^{2})+\ln(x^{2}+x)$
Résolution
$\begin{array}{rcrcl} f_{1}(x)\;\exists&\Leftrightarrow&2x&>&0\\ \\&\Leftrightarrow&x&>&0 \end{array}$
Donc, $x\in\;]0\;;\ +\infty[$, d'où $D_{f_{1}}=]0\;;\ +\infty[$
$\begin{array}{rcrcl} f_{2}(x)\;\exists&\Leftrightarrow&4x+3&>&0\\ \\&\Leftrightarrow&x&>&-\dfrac{3}{4} \end{array}$
Ainsi, $D_{f_{2}}=\left]-\dfrac{3}{4}\;;\ +\infty\right[$
$\begin{array}{rcl} f_{3}(x)\;\exists&\Leftrightarrow&x\neq 0\end{array}$
Donc, $D_{f_{3}}=\mathbb{R}\setminus\left\{0\right\}$
$\begin{array}{rcrcl} f_{4}(x)\;\exists&\Leftrightarrow&\dfrac{x+3}{x-2}&\neq&0\\ \\&\Leftrightarrow&(x+3)&\neq&0\\ \\&\Leftrightarrow&x&\neq&-3\end{array}$
Or, on sait que $\dfrac{x+3}{x-2}$ existe si, et seulement si, $x\neq 2$
Par suite, $D_{f_{4}}=\mathbb{R}\setminus\left\{-3\;;\ 2\right\}$
$\begin{array}{rcl} f_{5}(x)\;\exists&\Leftrightarrow&x^{2}-4x+3>0\\ \\&\Leftrightarrow&x\in\;]-\infty\;;\ 1[\cup]3\;;\ +\infty[\end{array}$
D'où, $D_{f_{5}}=]-\infty\;;\ 1[\cup]3\;;\ +\infty[$
$\begin{array}{rcl} f_{6}(x)\;\exists&\Leftrightarrow&\left\lbrace\begin{array}{rcl} 9-x^{2}&>&0\\x^{2}+x&>&0\end{array}\right.\\ \\&\Leftrightarrow&\left\lbrace\begin{array}{lcl} x\in\;]-3\;;\ 3[\\ x\in\;]-\infty\;;\ -1[\cup]0\;;\ +\infty[\end{array}\right.\\ \\&\Leftrightarrow&x\in\;]-3\;;\ -1[\cup]0\;;\ 3[\end{array}$
Ainsi, $D_{f_{6}}=]-3\;;\ -1[\cup]0\;;\ 3[$
I.2 Propriétés
$\centerdot\ \ (\ln x)'=\dfrac{1}{x}\;,\quad(\ln u)'=u'\times\dfrac{1}{u}=\dfrac{u'}{u}\;,\quad(\ln|u|)'=\dfrac{u'}{u}$ avec $u$ une fonction dérivable et strictement positive sur un intervalle $I$ de $\mathbb{R}.$
$\centerdot\ \ $ Soit $f(x)=\ln x$, on a :
$\begin{array}{rcl} f\ :\ ]0\;;\ +\infty[&\longrightarrow&\mathbb{R}\\ x&\longmapsto&\ln x \end{array}$ et $f'(x)=\dfrac{1}{x}>0$ donc, $f$ est strictement croissante.
Tableau de variation
$$\begin{array}{|c|lcr|}\hline x&0&&+\infty\\ \hline f'(x)&||&+&\\ \hline &||&&\\f&||&\nearrow&\\&||&&\\ \hline \end{array}$$
De plus :
$-\ $ si $0<x\leq 1$ alors, $\ln x\leq 0$
$-\ $ si $x> 1$ alors, $\ln x> 0$
$\centerdot\ \ a>0$ et soit $g$ la fonction définie par $g(x)=\ln ax.$
On a : $D_{g}=]0\;;\ +\infty[$ et $g$ est dérivable sur $]0\;;\ +\infty[.$
Soit $g'(x)=\dfrac{a}{ax}=\dfrac{1}{x}=f'(x)$ donc, $g(x)=f(x)+c\quad\forall\;x>0$
Par suite, $g(1)=f(1)+c$ ce qui entraîne que $c=\ln a$
D'où : $\boxed{\ln ax=\ln x+\ln a}$
$\centerdot\ \ $ On a : $a\times\dfrac{1}{a}=1$ et soit $f(x)=\ln x$ une fonction continue et strictement croissante sur $]0\;;\ +\infty[$ donc bijective.
D'où : $\ln\left(a\times\dfrac{1}{a}\right)=\ln 1\ \Rightarrow\ \ln a+\ln\dfrac{1}{a}=0$
Par conséquent, $\boxed{\ln\dfrac{1}{a}=-\ln a\;,\quad a>0}$
$\centerdot\ \ a>0\;,\ b>0$ alors on a :
$\ln\left(\dfrac{a}{b}\right)=\ln\left(a\times\dfrac{1}{b}\right)=\ln a+\ln\dfrac{1}{b}$
Donc, $\boxed{\ln\left(\dfrac{a}{b}\right)=\ln a-\ln b}$
$\centerdot\ \ a>0\;,\ \ln a^{p}=p\ln a\quad p\in\mathbb{Z}$
Démontrons par récurrence
$\centerdot\ \ p>0$
Pour $p=1$ on a $\ln a=\ln a$ et $1\ln a=\ln a$ donc, la proposition est vérifiée.
Supposons que $\ln a^{n}=n\ln a$ et montrons que $\ln a^{n+1}=(n+1)\ln a.$
On a :
$\begin{array}{rcl}\ln a^{n+1}&=&\ln (a^{n}\times a)\\ \\&=&\ln a^{n}+\ln a\\ \\&=&n\ln a+\ln a\\ \\&=&(n+1)\ln a\end{array}$
Donc, $\forall\;n\in\mathbb{N}\;,\ \ln a^{n}=n\ln a$
$\centerdot\ \ p\in\mathbb{Z}\setminus\mathbb{N}\ \Rightarrow\ p=-n$ avec $n\in\mathbb{N}$
On a :
$\begin{array}{rcl}\ln a^{p}&=&\ln a^{-n}\\ \\&=&\ln\left(\dfrac{1}{a^{n}}\right)\\ \\&=&-\ln a^{n}\\ \\&=&-n\ln a\\ \\&=&p\ln a\end{array}$
D'où : $\boxed{\forall\;p\in\mathbb{Z}\;,\ \ln a^{p}=p\ln a}$
$\centerdot\ \ \lim\limits_{x\rightarrow +\infty}\ln x$
On sait que $\lim\limits_{x\rightarrow +\infty}f(x)=+\infty$ se traduit par :
$\forall\;A>0\;,\ \exists\;B>0\ \text{ tel que si } x\geq B\ \text{ alors }f(x)\geq A$
Donc, $\forall\;A=n\ln 2\;,\ \exists\;B>0\ \text{ tel que si } x\geq B\ \text{ alors }f(x)\geq n\ln 2$
En posant $B=2^{n}$ on obtient : $x\geq 2^{n}\ \Rightarrow\ \ln x\geq 2^{n}$
D'où : $\boxed{\lim\limits_{x\rightarrow +\infty}\ln x=+\infty}$
$\centerdot\ \ \lim\limits_{x\rightarrow 0^{+}}\ln x$
On a :
$\begin{array}{rcl}\lim\limits_{x\rightarrow 0^{+}}\ln x&=&\lim\limits_{X\rightarrow +\infty}\ln\dfrac{1}{X}\quad\text{avec }X=\dfrac{1}{x}\\ \\&=&\lim\limits_{X\rightarrow +\infty}(-\ln X)\\ \\&=&-\infty\end{array}$
Donc, $\boxed{\lim_{x\rightarrow 0^{+}}\ln x=-\infty}$
$\centerdot\ \ f(x)=\ln x$ est une fonction continue et strictement croissante de $]0\;;\ +\infty[$ vers $\mathbb{R}$ donc c'est une bijection.
Ainsi, $1\in\mathbb{R}$ alors, il existe un unique $x_{0}\in\;]0\;;\ +\infty[$ tel que $f(x_{0})=1$
D'où : $\boxed{x_{0}=\mathrm{e}\simeq 2.72}$
$\centerdot\ \ a\geq b$ si, et seulement si, $\ln a\geq \ln b$
$\centerdot\ \ a>0$ alors on a :
$\begin{array}{rcl} a=(\sqrt{a})^{2}&\Rightarrow&\ln a=\ln(\sqrt{a})^{2}\\ \\&\Rightarrow&\ln a=2\ln\sqrt{a}\\ \\&\Rightarrow&\ln\sqrt{a}=\dfrac{1}{2}\ln a \end{array}$
D'où, $\boxed{\ln\sqrt{a}=\dfrac{1}{2}\ln a}$
Remarque :
$p\ln\mathrm{e}=\ln\mathrm{e}^{p}$
Exercice d'application
Donner les valeurs de :
$\ln 9\;,\quad\ln 6\;,\quad\ln\dfrac{16}{49}\;,\quad\ln\dfrac{5\sqrt{5}}{8}$
sachant que $\ln 2\simeq 0.69\;,\quad\ln 3\simeq 1.09\;,\quad\ln 5\simeq 1.69\;,\quad\ln 7\simeq 1.94$
Résolution
$\begin{array}{rcl}\ln 9&=&\ln 3^{2}\\ \\&=&2\ln 3\\ \\&\simeq&2\times 1.09\\ \\&\simeq&2.18\end{array}$
Donc, $\ln 9\simeq 2.18$
$\begin{array}{rcl}\ln 6&=&\ln(2\times 3)\\ \\&=&\ln 2+\ln 3\\ \\&\simeq&0.69+1.09\\ \\&\simeq&1.78\end{array}$
Donc, $\ln 6\simeq 1.78$
$\begin{array}{rcl}\ln\dfrac{16}{49}&=&\ln\left(\dfrac{4}{7}\right)^{2}\\ \\&=&2\ln\dfrac{4}{7}\\ \\&=&2(\ln 4-\ln 7)\\ \\&\simeq&2(2\times 0.69-1.94)\\ \\&\simeq&-1.12\end{array}$
Donc, $\ln\dfrac{16}{49}\simeq -1.12$
$\begin{array}{rcl}\ln\dfrac{5\sqrt{5}}{8}&=&\ln 5\sqrt{5}-\ln 8\\ \\&=&\ln 5+\ln \sqrt{5}-\ln(2\sqrt{2})^{2}\\ \\&=&\ln 5+\dfrac{1}{2}\ln 5-2\ln 2\sqrt{2}\\ \\&=&\ln 5+\dfrac{1}{2}\ln 5-2\left(\ln 2+\dfrac{1}{2}\ln 2\right)\\ \\&\simeq&1.69+\dfrac{1}{2}\times 1.69-2\left(0.69+\dfrac{1}{2}\times 0.69\right)\\ \\&\simeq&2.535-2.07\\ \\&\simeq&0.465\end{array}$
Donc, $\ln\dfrac{5\sqrt{5}}{8}\simeq 0.465$
II Équations - Inéquations
Exemple
Résoudre dans $\mathbb{R}$
1) $\ln(2x-3)=-1$
2) $\ln(3x+5)-\ln(x-2)=1$
3) $\ln^{2}x-4\ln x+3\geq 0$
Résolution
1)
$\begin{array}{rcl} \ln(2x-3)=-1\ \text{ existe}&\Leftrightarrow&2x-3>0\\ \\&\Leftrightarrow&x>\dfrac{3}{2}\end{array}$
D'où, $D_{E}=\left]\dfrac{3}{2}\;;\ +\infty\right[$
Par suite, on a :
$\begin{array}{rcl} \ln(2x-3)=-1&\Rightarrow&\ln(2x-3)=-\ln\mathrm{e}\\ \\&\Rightarrow&\ln(2x-3)=\ln\dfrac{1}{\mathrm{e}}\\ \\&\Rightarrow&2x-3=\dfrac{1}{\mathrm{e}}\\ \\&\Rightarrow&x=\dfrac{1}{2\mathrm{e}}+\dfrac{3}{2}\end{array}$
Comme $\left(\dfrac{1}{2\mathrm{e}}+\dfrac{3}{2}\right)\in D_{E}$ alors, $S=\left\{\dfrac{1}{2\mathrm{e}}+\dfrac{3}{2}\right\}$
2)
$\begin{array}{rcl}\ln(3x+5)-\ln(x-2)=1\ \text{ existe}&\Leftrightarrow&\left\lbrace\begin{array}{rcl} 3x+5&>&0\\x-2&>&0\end{array}\right.\\ \\&\Leftrightarrow&\left\lbrace\begin{array}{lcl} x&>&-\dfrac{5}{3}\\ x&>&2\end{array}\right.\end{array}$
Ainsi, $D_{E}=]2\;;\ +\infty[$
Par conséquent,
$\begin{array}{rcl}\ln(3x+5)-\ln(x-2)=1&\Rightarrow&\ln\left(\dfrac{3x+5}{x-2}\right)=\ln\mathrm{e}\\ \\&\Rightarrow&\dfrac{3x+5}{x-2}=\mathrm{e}\\ \\&\Rightarrow&3x+5=\mathrm{e}x-2\mathrm{e}\\ \\&\Rightarrow&x=\dfrac{5+2\mathrm{e}}{\mathrm{e}-3}\end{array}$
Or, $\dfrac{5+2\mathrm{e}}{\mathrm{e}-3}\notin D_{E}$ donc, $S=\emptyset$
3) $\ln^{2}x-4\ln x+3\geq 0$ existe si, et seulement si, $x>0.$
D'où : $D_{E}=]0\;;\ +\infty[$
Pour résoudre l'inéquation $\ln^{2}x-4\ln x+3\geq 0$ on procède par changement de variable en posant $X=\ln x.$
Ainsi, l'inéquation devient $X^{2}-4X+3\geq 0$
Soit $X_{1}=1$ et $X_{2}=3$ solutions de l'équation $X^{2}-4X+3=0$
Alors, $(X-1)(X-3)=(\ln x-1)(\ln x-3)$
Par suite,
$\begin{array}{rcrcl}\ln x-1\geq 0&\Leftrightarrow&\ln x&\geq&1\\ \\&\Leftrightarrow&\ln x&\geq&\ln\mathrm{e}\\ \\&\Leftrightarrow&x&\geq&\mathrm{e}\end{array}$
$\begin{array}{rcrcl}\ln x-3\geq 0&\Leftrightarrow&\ln x&\geq&3\\ \\&\Leftrightarrow&\ln x&\geq&\ln\mathrm{e}^{3}\\ \\&\Leftrightarrow&x&\geq&\mathrm{e}^{3}\end{array}$
Par conséquent, en utilisant le tableau de signes suivant
$$\begin{array}{|c|lcccccr|}\hline x&0&&\mathrm{e}&&\mathrm{e}^{3}&&+\infty\\ \hline\ln x-1&||&-&0&+&|&+&\\ \hline\ln x-3&||&-&|&-&0&+&\\ \hline\ln^{2}x-4\ln x+3&||&+&0&-&0&+&\\ \hline\end{array}$$
On obtient : $S=]0\;;\ \mathrm{e}]\cup[\mathrm{e}^{3}\;;\ +\infty[$
III Courbe et compléments
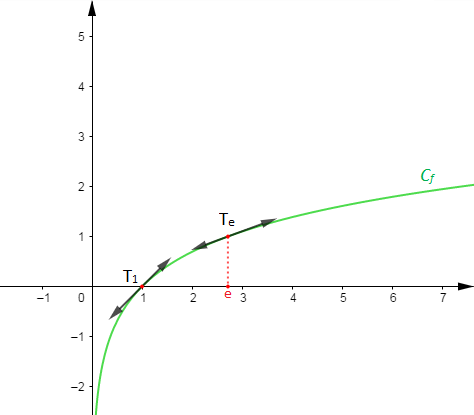
Soit $f$ la fonction définie par $f(x)=\ln x$ de courbe représentative $C_{f}$ alors, $D_{f}=]0\;;\ +\infty[.$
On a : $\lim\limits_{x\rightarrow 0^{+}}f(x)=-\infty$ donc, la droite d'équation $x=0$ est une asymptote verticale à $C_{f}.$
Aussi, $\lim\limits_{x\rightarrow +\infty}f(x)=+\infty$ donc, calculons $\lim_{x\rightarrow +\infty}\dfrac{f(x)}{x}.$
Pour cela, considérons la fonction $\varphi$ définie sur $]0\;;\ +\infty[$ par : $$\varphi(x)=\ln x-\sqrt{x}$$
Sa fonction dérivée $\varphi'$ est donnée par : $\varphi'(x)=\dfrac{1}{x}-\dfrac{1}{2\sqrt{x}}=\dfrac{2-\sqrt{x}}{2x}$
Alors on a :
$\begin{array}{rcrcl}\varphi'(x)\geq 0&\Leftrightarrow&2-\sqrt{x}&\geq&0\\ \\&\Leftrightarrow&-\sqrt{x}&\geq&-2\\ \\&\Leftrightarrow&x&\leq&4\end{array}$
Donc, $\varphi$ est croissante sur $]0\;;\ 4]$ et décroissante sur $[4\;;\ +\infty[.$
De plus, $\varphi(4)=\ln 4-2=2\ln 2-2\simeq -0.62<0$
$$\begin{array}{|c|lcccr|}\hline x&0&&4&&+\infty\\ \hline\varphi'(x)&||&+&0&-&\\ \hline&||&&-0.62&&\\ \varphi&||&\nearrow&|&\searrow&\\&-\infty&&|&&-\infty\\ \hline\end{array}$$
Par suite, nous constatons que :
$\forall\;x\in\;]0\;;\ +\infty[\;,\ \varphi(x)\leq 0$ c'est-à-dire $\ln x\leq\sqrt{x}.$
Par conséquent, $\forall\;x\geq 1\;,\ 0\leq\ln x\leq\sqrt{x}.$
D'où : $$0\leq\dfrac{\ln x}{x}\leq\dfrac{\sqrt{x}}{x}=\dfrac{1}{\sqrt{x}}$$
Or, $\lim\limits_{x\rightarrow +\infty}\dfrac{1}{\sqrt{x}}=0$ donc, en appliquant le théorème des gendarmes on obtient :
$\boxed{\lim_{x\rightarrow +\infty}\dfrac{\ln x}{x}=0}$
Ainsi, $C_{f}$ admet une branche parabolique de direction $(x'Ox).$
Par ailleurs, $f'(x)=\dfrac{1}{x}>0$
Tableau de variation
$$\begin{array}{|c|lcr|}\hline x&0&&+\infty\\ \hline f'(x)&||&+&\\ \hline&||&&+\infty\\ f&||&\nearrow&\\&-\infty&&\\ \hline\end{array}$$
Soit $T_{1}\ $ et $\ T_{\mathrm{e}}$ les tangentes à $C_{f}$ respectivement aux points d'abscisses $1\ $ et $\ \mathrm{e}$ alors on a :
$\begin{array}{rcl} T_{1}\ :\ y&=&f'(1)(x-1)+f(1)\\ \\&=&x-1\end{array}$
Donc, $\boxed{T_{1}\ :\ y=x-1}$
$\begin{array}{rcl} T_{\mathrm{e}}\ :\ y&=&f'(\mathrm{e})(x-\mathrm{e})+f(\mathrm{e})\\ \\&=&\dfrac{1}{\mathrm{e}}(x-\mathrm{e})+1\\ \\&=&\dfrac{1}{\mathrm{e}}x\end{array}$
D'où : $\boxed{T_{\mathrm{e}}\ :\ y=\dfrac{1}{\mathrm{e}}x}$
Représentation graphique
Autres limites
$\centerdot\ \ \lim\limits_{x\rightarrow 0^{+}}x\ln x$
On a :
$\begin{array}{rcl}\lim\limits_{x\rightarrow 0^{+}}x\ln x&=&\lim\limits_{X\rightarrow +\infty}\dfrac{1}{X}\ln\dfrac{1}{X}\quad\text{avec }X=\dfrac{1}{x}\\ \\&=&\lim\limits_{X\rightarrow +\infty}-\dfrac{\ln X}{X}\\ \\&=&0\end{array}$
Donc, $\boxed{\lim_{x\rightarrow 0^{+}}x\ln x=0}$
$\centerdot\ \ \lim\limits_{x\rightarrow 0^{+}}\dfrac{\ln(1+x)}{x}$
Soit $\varphi(x)=\ln(1+x)$ la fonction dérivable sur $]-1\;;\ +\infty[$ et $\varphi'(x)=\dfrac{1}{1+x}$ sa fonction dérivée.
On a :
$\begin{array}{rcl}\lim\limits_{x\rightarrow 0}\dfrac{\ln(1+x)}{x}&=&\lim\limits_{x\rightarrow 0}\dfrac{\varphi(x)-\varphi(0)}{x-0}\\ \\&=&\varphi'(0)\\ \\&=&1\end{array}$
D'où : $\boxed{\lim_{x\rightarrow 0}\dfrac{\ln(1+x)}{x}=1}$
$\centerdot\ \ \lim\limits_{x\rightarrow 1}\dfrac{\ln x}{x-1}$
Soit $f(x)=\ln x$ donc,
$\begin{array}{rcl}\lim\limits_{x\rightarrow 1}\dfrac{\ln x}{x-1}&=&\lim\limits_{x\rightarrow 1}\dfrac{f(x)-f(1)}{x-1}\\ \\&=&f'(1)\\ \\&=&1\end{array}$
D'où : $\boxed{\lim_{x\rightarrow 1}\dfrac{\ln x}{x-1}=1}$
Exercice d'application
Calculer les limites suivantes
1) $\lim\limits_{x\rightarrow 0}\dfrac{\ln(1+4x)}{3x}$
2) $\lim\limits_{x\rightarrow 0^{+}}x^{n}\ln x\;,\quad n\geq 2$
3) $\lim\limits_{x\rightarrow 0^{+}}x\ln^{n}x\;,\quad n\geq 1$
Résolution
1)
$\begin{array}{rcl}\lim\limits_{x\rightarrow 0}\dfrac{\ln(1+4x)}{3x}&=&\lim\limits_{x\rightarrow 0}\dfrac{\ln(1+4x)}{4x}\times\dfrac{4}{3}\\ \\&=&\lim\limits_{X\rightarrow 0}\dfrac{\ln(1+X)}{X}\times\dfrac{4}{3}\quad\text{avec }X=4x\\ \\&=&\dfrac{4}{3}\quad\text{car }\lim\limits_{X\rightarrow 0}\dfrac{\ln(1+X)}{X}=1\end{array}$
2)
$\begin{array}{rcl}\lim\limits_{x\rightarrow 0^{+}}x^{n}\ln x&=&\lim\limits_{x\rightarrow 0^{+}}x^{n-1}\times x\ln x\\ \\&=&0\end{array}$
3)
$\begin{array}{rcl}\lim\limits_{x\rightarrow 0^{+}}x\ln^{n} x&=&\lim\limits_{X\rightarrow 0^{+}}X^{n}\ln^{n} X^{n}\quad\text{avec }X^{n}=x\\ \\&=&\lim\limits_{X\rightarrow 0^{+}}X^{n}(n\ln X)^{n}\\ \\&=&\lim\limits_{X\rightarrow 0^{+}}n^{n}(X\ln X)^{n}\\ \\&=&0\end{array}$
IV Fonctions logarithmes de base $a>0\;,\ a\neq 1$
IV.1 Définition
On appelle fonction logarithme de base $a$ la fonction notée : $$\log_{a}(x)=\dfrac{\ln x}{\ln a}$$
Exemple
$-\ \ $ fonction logarithme décimal notée : $\log_{10}(x)=\dfrac{\ln x}{\ln 10}$
$-\ \ $ fonction logarithme népérien notée : $\log_{\mathrm{e}}(x)=\ln x$
IV.2 Étude
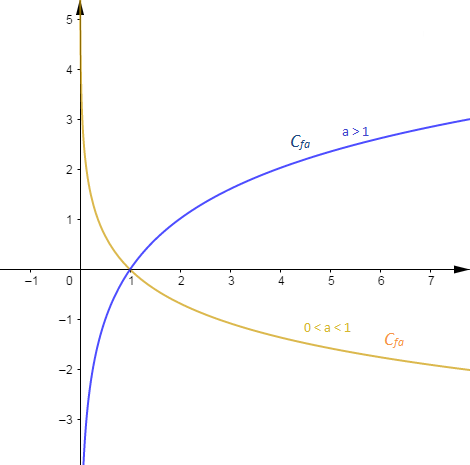
Soit $f_{a}$ la fonction définie par $f_{a}(x)=\log_{a}(x)=\dfrac{\ln x}{\ln a}$ de courbe représentative $C_{f_{a}}.$
Alors, $D_{f_{a}}=]0\;;\ +\infty[$ et on a :
$\begin{array}{rcl}\lim\limits_{x\rightarrow 0^{+}}f_{a}(x)&=&\lim\limits_{x\rightarrow 0^{+}}\dfrac{\ln x}{\ln a}\\ \\&=&\left\lbrace\begin{array}{rcl} +\infty&\text{si}&0<a<1\\-\infty&\text{si}&a>1\end{array}\right.\end{array}$
Donc, la droite d'équation $x=0$ est une asymptote verticale à $C_{f_{a}}.$
$\begin{array}{rcl}\lim\limits_{x\rightarrow +\infty}f_{a}(x)&=&\lim\limits_{x\rightarrow +\infty}\dfrac{\ln x}{\ln a}\\ \\&=&\left\lbrace\begin{array}{rcl} -\infty&\text{si}&0<a<1\\+\infty&\text{si}&a>1\end{array}\right.\end{array}$
Calculons alors, $\lim\limits_{x\rightarrow +\infty}\dfrac{f_{a}(x)}{x}.$ On a :
$\begin{array}{rcl}\lim\limits_{x\rightarrow +\infty}\dfrac{f_{a}(x)}{x}&=&\lim\limits_{x\rightarrow +\infty}\dfrac{\ln x}{x\ln a}\\\\&=&0\end{array}$
Ainsi, $C_{f_{a}}$ admet une branche parabolique de direction $(x'Ox).$
Soit $f'_{a}$ la fonction dérivée de $f_{a}$ alors, $$f'_{a}(x)=\left(\dfrac{\ln x}{\ln a}\right)'=\dfrac{1}{x\ln a}$$
Par suite, $f'_{a}(x)>0$ si, et seulement si, $a>1.$
Par conséquent, $f_{a}$ est croissante pour $a>1$ et décroissante si $0<a<1.$
Tableau de variation
$$a>1\qquad\qquad\qquad\quad 0<a<1$$
$$\begin{array}{|c|lcr|}\hline x&0&&+\infty\\ \hline f_{a}'(x)&||&+&\\ \hline&||&&+\infty\\ f_{a}&||&\nearrow&\\&-\infty&&\\ \hline\end{array}\qquad\begin{array}{|c|lcr|}\hline x&0&&+\infty\\ \hline f_{a}'(x)&||&-&\\ \hline&+\infty&&\\ f_{a}&||&\searrow&\\&||&&-\infty\\ \hline\end{array}$$
Illustration graphique
Auteur:
Diny Faye & Seyni Ndiaye
Ajouter un commentaire