Corrigé Bac Maths S2 1er groupe 2014
Exercice 1
1. Soit z un nombre complexe non nul donné.
- L'écriture algébrique de z est de la forme : z=a+ib, avec a sa partie réelle et b sa partie imaginaire.
- L'écriture exponentielle de z est de la forme : z=reiθ, avec r son module et θ un de ses arguments.
- L'écriture trigonométrique de z est de la forme : z=r(cosθ+isinθ), avec r son module et θ un de ses arguments.
2. Soient K(z0), M(z) et M′(z′) et soit r la rotation de centre K(z0) qui transforme M(z) en M′(z′) on a : {KM′=KM(→KM, →KM′)=θ [2π](1)
Ce qui est équivalent à {|z′−z0|=|z−z0|arg(z′−z0z−z0)≡θ [2π](2)
D'où z′=eiθz+z0(1−eiθ)
B) Soit z0=1−i√3
1. Écriture trigonométrique de z0.
On a |z0|=√1+3=2,
Soit θ un argument de z0 alors cosθ=12 et sinθ=−√32 ce qui donne θ=−π3 [2π] d'où z0=2(cosπ3−isinπ3)
2. Calculons z40.
z40=[2(cosπ3−isinπ3)]4=16(cos4π3−isin4π3)=16(−12+i√32)
D'où z40=−8+i8√3
3. Résolvons l'équation z4=1
z4=1 implique |z|4=1 et 4argz=0 [2π] ce qui donne |z|=1 et argz=kπ2,k∈Z
d'où l'ensemble des solutions S de l'équation z4=1 est S={−1; 1; i; −i}
4. Déduisons-en les solutions de (E) : z4=−8+8i√3.
z4=−8+8i√3 est équivalent à z4−8+8i√3=1
ce qui est équivalent, d'après B) 2), à (z1−i√3)4=1
Ce qui donne d'après B) 3) les solutions suivantes :
- Sous forme algébrique : z0=1−i√3, z1=−1+i√3, z2=√3+i, z3=−√3−i
- Sous forme trigonométrique : z0=2(cosπ3−isinπ3), z1=2(cos2π3+isin2π3), z2=2(cosπ6+isinπ6), z3=2(cos7π6+isin7π6)
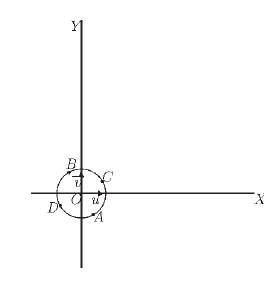
5. 

6. Soit r la rotation de centre O et d'angle π2
D'après A) 2) si M′(z′) est l'image de M(z) par r alors z′=zeiπ2
7. Soient les points A, B, C et D d'affixes respectives zA=z0, zB=z1, zC=z2 et zD=z3.
Vérifions que r(A)=C.
zAeiπ2=2e−iπ3eiπ2=2eiπ6=zC
d'où r(A)=C.
Vérifions que r(C)=B.
zCeiπ2=2eiπ6eiπ2=2ei4π6=2ei2π3=zB
d'où r(C)=B.
Vérifions que r(B)=D.
zBeiπ2=2ei2π3eiπ2=2ei7π6=zD
d'où r(B)=D.
8. r(A)=C implique |zA|=|zC|,
r(C)=B implique |zC|=|zB|,
r(B)=D implique |zB|=|zD|
D'où |zA|=|zC|=|zB|=|zD|=2
Ce qui est équivalent à OA=OB=OC=OD=2
D'où les points A, B, C et D sont situés sur le même cercle de centre O et de rayon 2.
Exercice 2
Une boîte contient 8 cubes indiscernables au toucher : R1, R2, R2, R2, V1, V1, V2, J2.
R représente la couleur rouge, V la couleur verte, J la couleur jaune, 1 et 2
les numéros des couleurs.
A) Soit (Ω, P(Ω), p) un espace probabilisé. Soient A et B deux événements de cet espace probabilisé.
A et B sont indépendants si et seulement si p(A∩B)=p(A)×p(B)
B) On choisit successivement et sans remise 2 cubes de la boîte.
1. Soient les événements A : "Obtenir des cubes de couleurs différentes" et
B : "Obtenir au plus un cube portant le numéro 2"
a) p(A)=A14×A14+A13×A15+A11×A17A28=1928
b) p(¯B)=A25A28=514 d'où p(B)=914
c) Calculons p(A∩B) et comparons-le avec p(A)×p(B)
A∩B, l'événement : "Obtenir des cubes de couleurs différentes avec au plus un portant le numéro 2".
Les différentes possibilités sont :
(R1, V1), (R1, V1), (V1, R1), (V1, R1)
(R2, V1), (V1, R2), (R2, V1), (V1, R2), (R2, V1), (V1, R2)
(R2, V1), (V1, R2), (R2, V1), (V1, R2), (R2, V1), (V1, R2), (R1, J2)
(J2, R1), (V1, J2), (J2, V1), (V1, J2), (J2, V1), (R1, V2), (V2, R1)
(R2, V1), (V1, R2), (R2, V1), (V1, R2), (R2, V1), (V1, R2)
(R2, V1), (V1, R2), (R2, V1), (V1, R2), (R2, V1), (V1, R2), (R1, J2)
(J2, R1), (V1, J2), (J2, V1), (V1, J2), (J2, V1), (R1, V2), (V2, R1)
Donc p(A∩B)=37
Ou encore p(A∩B)=C12×A22+C13×C12×A22+A22+C12×A22+A22A28=37
Or p(A)×p(B)=914×1928 qui est différent de 3 d'où A et B ne sont pas indépendants.
2. Les valeurs prises par X :
X={0, 1, 2}
a) La loi de probabilité de X :
X=ai012p(X=ai)31447314
b) Espérance mathématique E(X) de X :
E(X)=∑mj=1xjp(X=xj),
E(X)=47+2×314=1
c) Variance V (X) de X :
V(X)=∑mj=1x2jp(X=xj)−(E(X))2,
V(X)=47+4×314−1=37
C) Tirage simultané de 3 cubes de la boîte :
a) p(C)=C33×C23×C15C58=27
b) pn=p(Dn)=1−(57)n
c) (pn)n∈N est strictement croissante et limn→+∞pn=1
Problème
PARTIE A
1. a) g(x) existe si =≠1 avec x>0
Dg=]0; 1[∪]1; +∞[
b) limx→0+g(x)=0; limx→+∞g(x)=−∞
limx→1−g(x)=limx→1−xx−1−ln|x−1|=limx→1−x−(x−1)ln(1−x)x−1=−∞
limx→1+g(x)=limx→1+xx−1−ln|x−1|=limx→1+x−(x−1)ln(x−1)x−1=+∞
2) x↦ xx−1 et x↦ ln|x−1| sont dérivables pour tout x≠1 d'où g est dérivable sur Dg et g′(x)=−1(x−1)2−1x−1=−x(x−1)2
Sur Dg, g′(x)<0.
Tableau de variations de g :
x01+∞g′(x)−−0+∞g↘↘−∞−∞
3. Sur ]1; +∞[, g est dérivable et strictement décroissante donc elle réalise
une bijection de ]1; +∞[ vers ]−∞; +∞[.
Or 0∈R il existe donc α∈]1; +∞[ tel que g(α)=0.
Montrons que 4<α<5.
g(4)≃0.23 et g(5)≃−0.14
g(4)×g(5)<0 donc 4<α<5.
4. Sur ]1; α[ g(x)>0 et sur ]α; +∞[ g(x)<0
PARTIE B
Soit
f(x)={ln|x−1|x,si x>0−6exe2x+3ex+2,si x≤0(3)
1. a) x↦ ln|x−1|x est définie si x≠0 et x≠1,
d'où elle est définie sur ]0; 1[∪]1; +∞[.
−6exe2x+3ex+2 est définie pour tout x∈R, d'où elle est définie sur ]−∞; 0].
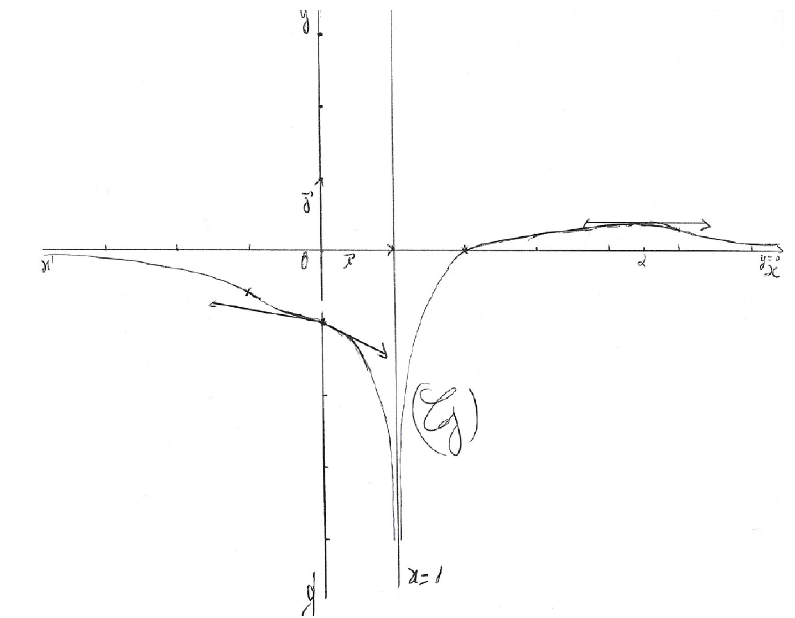
Donc Df=]−∞; 0]∪]0; 1[∪]1; +∞[=R∖{1}
limx→−∞f(x)=limx→−∞−6exe2x+3ex+2=0; limx→+∞f(x)=limx→+∞ln|x−1|x=0
limx→1−f(x)=limx→1−ln(1−x)x=−∞; limx→1+f(x)=limx→1+ln(x−1)x=−∞
b) La droite d'équation x=1 est une asymptote verticale à la courbe
(Cf) de f et la droite d'équation y=0 est une asymptote horizontale à la courbe (Cf) de f aux voisinages de −∞ et +∞.
2. a) limx→0−f(x)=limx→0−−6exe2x+3ex+2=−1; limx→0+f(x)=limx→0+ln(1−x)x=−1
on a aussi f(0)=−6e0e0+3e0+2=−1
limx→0−f(x)=limx→0+f(x)=f(0)
Donc f est continue en 0.
b) On admet que limx→0+ln(1−x)+xx2=−12.
Et on a limx→0−f(x)−f(0)x−0=−6exe2x+3ex+2+1x=limx→0−e2x−3ex+2x(e2x+3ex+2)=limx→0−e2x−3ex+2xe2x+3ex+2
Or limx→0−e2x−3ex+2x=e2×0−3e0=−1
d'où limx→0−f(x)−f(0)x−0=−16.
f′d(0)=−12 et f′g(0)=−16, f′d(0)≠f′g(0) d'où (Cf) admet deux demi-tangentes au point d'abscisse 0.
3. a) f(α)=ln(α−1)α or g(α)=0 nous donne αα−1=ln(α−1) d'où f(α)=αα−1×1α=1α−1.
b) Sur ]0; 1[∪]1; +∞[, f′(x)=1x−1x−ln|x−1|x2=xx−1−ln|x−1|x2=g(x)x2
Sur ]−∞; 0[, f′(x)=−6ex(3e2x+6ex+2)(e2x+3ex+2)2
Sur ]0; 1[∪]1; +∞[, f′(x) a le même signe que g(x),
Sur ]−∞; 0[, f′(x)<0.
Dressons le tableau de variations de la fonction f.
x−∞01α+∞f′(x)−−16||−12−+0−01α−1f(x)↘−1↗↘↘−∞−∞0
4. Traçons la courbe (Cf) de f dans un repère orthonormé (0; →i, →j) d'unité graphique 2cm.
5. a) −6xx2+3x+2=ax+2+bx+1 nous donne a=−12 et b=6,
d'où −6xx2+3x+2=−12x+2+6x+1.
b) D'après a) −6exe2x+3ex+2=−12ex+2+6ex+1=−12ex(1+2e−x)+6ex(1+e−x)
D'où −6exe2x+3ex+2=−12e−x1+2e−x+6e−x1+e−x
c) Soit A l'aire du domaine délimité par l'axe des abscisses, la courbe,
les droites d'équations x=−ln2 et x=0.
A=∫0−ln2−f(x)dx×u.a=∫0−ln2−−6exe2x+3ex+2dx×u.a
=∫0−ln212e−x1+2e−x−6e−x1+e−xdx=[−6ln(1+2e−x)+6ln(1+e−x)]0−ln2×4cm2
d'où A=24ln109cm2

Commentaires
Anonyme (non vérifié)
lun, 06/27/2022 - 08:09
Permalien
Très bien
Anonyme (non vérifié)
lun, 06/27/2022 - 08:09
Permalien
Très bien
Anonyme (non vérifié)
lun, 06/27/2022 - 08:10
Permalien
Waw c’est bien
ABDEL ABDOU MAJANI (non vérifié)
mar, 08/23/2022 - 20:34
Permalien
MATHEMATIQUE
ABDEL ABDOU MAJANI (non vérifié)
mar, 08/23/2022 - 20:34
Permalien
MATHEMATIQUE
Ajouter un commentaire