Solution des exercices : Énergie cinétique - 1er s
Classe:
Première
Exercice 1
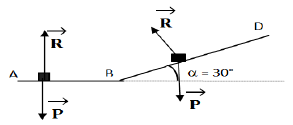
1. Exprimons, puis calculons l'énergie cinétique de l'autoporteur en $A.$
$\begin{array}{rcl} E_{C_{A}} &=&\dfrac{1}{2}mV_{A}^{2}\\ &=&\dfrac{1}{2}\times 600\cdot 10^{-3}\times 6^{2} \\\Rightarrow E_{C_{A}} &=&10.8J \end{array}$
2. Inventaire des forces extérieures agissant sur l'autoporteur au cours de la phase $AB.$
Les forces extérieures sont : $\overrightarrow{P}$ et $\overrightarrow{R}$
3. a) Définition d'un système pseudo-isolé ;
Un système pseudo-isolé est un système soumis à des forces qui se compensent.
b) L'autoporteur est pseudo-isolé au cours de la phase $AB$, car, pendant cette phase les forces se compensent.
Par contre, pendant la phase $BD$, les forces ne se compensent plus, et le système n'est plus pseudo-isolé
c) Déduction de la vitesse du centre d'inertie du mobile en $B$
Le théorème de l'énergie cinétique s'écrit :
$\begin{array}{rcl} \Delta BC &=& \sum\,W\left(\overrightarrow{F}_{extérieurs}\right)\\\Rightarrow\;E_{CB}-E_{CA}&=&W_{AB}\left(\overrightarrow{P}\right)+W_{AB}\left(\overrightarrow{R}\right) \\\Rightarrow\dfrac{1}{2}mV_{B}^{2}-\dfrac{1}{2}mV_{A}^{2}&=&0+0\\\Rightarrow\dfrac{1}{2}mV_{A}^{2}&=&\dfrac{1}{2}mV_{B}^{2}\\\Rightarrow\;V_{B}^{2}&=&V_{A}^{2}\\\Rightarrow\;V_{B}&=&V_{A}\\ \Rightarrow\;V_{B}&=&6m\cdot s^{-1} \end{array}$
4. Calcul du travail du poids de l'autoporteur et le travail de l'action $R$ du plan sur l'autoporteur au cours du déplacement $BC_{1}$
$\begin{array}{rcl} W_{BC_{1}}\left(\overrightarrow{P}\right) &=&\overrightarrow{P}\cdot\overrightarrow{BC_{1}}\\ &=&-mgBC_{1}\sin\alpha\nonumber\\ &=&-600\cdot 10^{-3}\times 10\times 1\times\sin 30^{\circ} \\ \Rightarrow\;W_{BC_{1}}\left(\overrightarrow{P}\right) &=&-3.0J \end{array}$
$W_{BC_{1}}\left(\overrightarrow{R}\right)=\overrightarrow{R}\cdot\overrightarrow{BC_{1}}=0\left(\overrightarrow{R}\perp\overrightarrow{BC_{1}}\right)$
5. Déduction de $V_{c_{1}}$
Le théorème de l'énergie cinétique au solide entre les instants $t_{B}$ et $t_{C_{1}}$ s'écrit :
$\begin{array}{rcl} E_{C_{c_{1}}}-E_{C_{B}}&=&W_{BC_{1}}\left(\overrightarrow{P}\right)+W_{BC_{1}}\left(\overrightarrow{R}\right)\\\Rightarrow\dfrac{1}{2}mV_{C_{1}}^{2}-\dfrac{1}{2}mV_{B}^{2}&=&-mgBC_{1}\sin\alpha+0\\\Rightarrow\;V_{C_{1}}^{2}-V_{B}^{2}&=&-2gBC_{1}\sin\alpha\\\Rightarrow\; V_{C_{1}}^{2}&=&V_{B}^{2}-2gBC_{1}\sin\alpha\\\Rightarrow\; V_{C_{1}} &=&\sqrt{V_{B_{1}}-2gBC_{1}\sin\alpha}\\ \Rightarrow\; V_{C_{1}}&=&\sqrt{6^{2}-2\times 10\times 1\times\sin 30^{\circ}}\\\Rightarrow\;V_{C_{1}} &=&5.1\,m\cdot s^{-1} \end{array}$
6. Déduction de $BC_{2}$ la distance parcourue par le mobile avant de rebrousser chemin en $C_{2}.$
$\begin{array}{rcl} E_{C_{c_{1}}}-E_{C_{B}} &=& W_{BC_{2}}\left(\overrightarrow{P}\right)+W_{BC_{2}}\left(\overrightarrow{R}\right)\\\Rightarrow\;0-\dfrac{1}{2}mV_{B}^{2} &=&-mgBC_{2}\sin\alpha+0 \\\Rightarrow \;-V_{B}^{2} &=& -2gBC_{2}\sin\alpha\\\Rightarrow\; 2gBC_{2}\sin\alpha &=&V_{B}^{2} \\\Rightarrow\; BC_{2} &=&\dfrac{V_{B}^{2}}{2g\sin\alpha}\\\Rightarrow\;BC_{2} &=&\dfrac{6^{2}}{2\times 10\sin 30^{\circ}}\\\Rightarrow \;BC_{2} &=&3.6\,m \end{array}$
Exercice 2
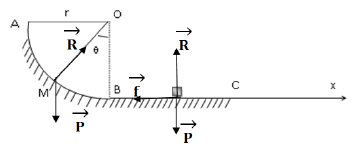
1.1. Bilan des forces qui s'appliquant sur le mobile au point $M$ sont : $\overrightarrow{P}$ et $\overrightarrow{R}.$
1.2. Expression du travail de chacune des forces, au point $M$, en fonction de $m$, $g$, $r$ et $\theta.$
$W_{AM}\left(\overrightarrow{P}\right)=\overrightarrow{P}\cdot\overrightarrow{AM}=mgr\cos\theta$
$W_{AM}\left(\overrightarrow{R}\right)=\overrightarrow{R}\cdot\overrightarrow{AB}=0\quad\text{car}\quad\overrightarrow{R}\perp\overrightarrow{AB}$
1.3. Établissement de l'expression littérale de la vitesse $V_{M}$ du mobile en fonction de $V_{A}$, $g$, $r$ et $\theta.$
Appliquons le théorème de l'énergie cinétique au point $M$ et
$\begin{array}{rcl} E_{C_{M}}-E_{C_{A}} &=& W_{AM}\left(\overrightarrow{P}\right)+W_{AM}\left(\overrightarrow{R}\right)\\\Rightarrow\dfrac{1}{2}mV_{M}^{2} &=&mgr\cos\theta+0\\\Rightarrow\dfrac{1}{2}mV_{M}^{2} &=&\dfrac{1}{2}mV_{A}^{2}+mgr\cos\theta\\\Rightarrow \;V_{M}^{2} &=&V_{A}^{2}+2gr\cos\theta\\\Rightarrow\; V_{M} &=&\sqrt{V_{A}^{2}+2gr\cos\theta} \end{array}$
1.4. Calcul de $V_{M}$ en $B$ $($pour $\theta=0).$
$\begin{array}{rcl} V_{M} &=&\sqrt{V_{A}^{2}+2gr\cos\theta}\ ;\ \text{pour }\theta=0\\\Rightarrow\; V_{M} &=& \sqrt{V_{A}^{2}+2gr\cos 0}\\\Rightarrow \;V_{B} &=&\sqrt{V_{A}^{2}+2gr}\\\Rightarrow \;V_{B} &=&\sqrt{5^{2}+2\times 10\times 1}\\\Rightarrow \;V_{B} &=&6.71\,m\cdot s^{-1} \end{array}$
2. Détermination de l'expression littérale et numérique de $f.$
$\begin{array}{rcl} E_{C_{C}}-E_{C_{B}} &=& W_{BC}\left(\overrightarrow{P}\right)+W_{BC}\left(\overrightarrow{R}\right)+W_{BC}\left(\overrightarrow{f}\right)\\\Rightarrow\dfrac{1}{2}mV_{C}^{2}-\dfrac{1}{2}mV_{B}^{2} &=&0+0-f\times BC\\\Rightarrow \;f &=&\dfrac{m\left(V_{B}^{2}\right)}{2BC}\\\Rightarrow\; f &=&\dfrac{0.1\times\left(6.71^{2}-5\right)}{2\times 1} \\\Rightarrow \;f &=& 1N \end{array}$
Exercice 3 Voiture tremplin

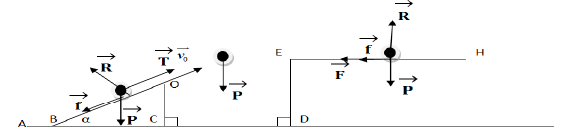
1. Dans le référentiel terrestre supposé galiléen, les forces s'exerçant sur le système {ensemble automobile-pilote} sont :
$-\ $dans la phase $BO$ : son poids $\overrightarrow{P}$, la réaction $\overrightarrow{R}$ du support, les frottements $\overrightarrow{f}$ et la traction $\overrightarrow{T}$ du système.
$-\ $dans la phase $OE$ : son poids $\overrightarrow{P}.$
$-\ $dans la phase $EH$ : son poids $\overrightarrow{P}$, la réaction normale $\overrightarrow{R}$ du support, les frottements $\overrightarrow{f}$ et la force de freinage $\overrightarrow{F}$
2. Si on suppose que le système est soumis à des forces qui ne se compensent pas dans la phase $BO$, alors le système n'est pas pseudo isolé (d'après le principe d'inertie).
Si on suppose qu'il évolue à vitesse constante et à trajectoire rectiligne, alors le système est pseudo isolé (d'après le principe d'inertie).
Dans la phase $OE$, le système n'est soumis qu'à son poids ; il n'est donc pas pseudo isolé.
Dans la phase $EH$, le système freine donc(les forces ne se compensent plus), d'après le principe d'inertie, il n'est pas pseudo isolé.
3. Détermination du travail de chaque force de chacune des phases :
$-\ $Phase $BO$
$\begin{array}{rcl} W_{BO}\left(\overrightarrow{P}\right) &=&-mg\cdot OC\\ &=&-1.00\cdot 10^{3}\times 9.81\times 8.00\\ &=&-78.5\cdot 10^{3}J \end{array}$
$W_{BO}\left(\overrightarrow{R}\right)=0\quad\text{car}\quad\overrightarrow{R}\perp\overrightarrow{BO}$
$\begin{array}{rcl} W_{BO}\left(\overrightarrow{f}\right) &=&\overrightarrow{f}\cdot BO\\ &=&-\dfrac{500\times 8.00}{\sin 15.5^{\circ}}\\\Rightarrow W_{BO}\left(\overrightarrow{f}\right) &=&-15.5\cdot 10^{3}J \end{array}$
$\begin{array}{rcl} W_{BO}\left(\overrightarrow{T}\right) &=& \overrightarrow{T}\cdot\overrightarrow{BO}\\ &=&\dfrac{T\times OC}{\sin\alpha} \end{array}$
$-\ $Phase $OE$
$\begin{array}{rcl} W_{BO}\left(\overrightarrow{P}\right) &=&-mg(ED-OC)\\ &=&-1.00\cdot 10^{3}\times 9.81\times(10.0-8.00)\\ &=&-19.6\cdot 10^{3}J\end{array}$
$-\ $Phase $EH$
$W_{EH}\left(\overrightarrow{P}\right)=0J\ (\overrightarrow{P}\perp\overrightarrow{BH})$
$W_{EH}\left(\overrightarrow{f}\right)=0J\ (\overrightarrow{R}\perp\overrightarrow{EH})$
$\begin{array}{rcl} W_{EH}\left(\overrightarrow{f}\right) &=&-500\times 100\\\Rightarrow\; W_{EH}\left(\overrightarrow{f}\right)&=&-50.0\cdot 10^{3}J \end{array}$
4. D'après le théorème de l'énergie cinétique (dans un référentiel galiléen, la variation d'énergie cinétique d'un système en translation entre deux points est égale à la somme des travaux des forces qui s'exercent sur ce système entre ces deux points.) entre $O$ et $E$, on a :
$$\dfrac{1}{2}mv_{1}^{2}-\dfrac{1}{2}mv_{0}^{2} =W_{OE}\left(\overrightarrow{P}\right)$$
$\begin{array}{rcl} \text{ soit }\ \dfrac{1}{2}mv_{1}^{2}-\dfrac{1}{2}mv_{0}^{2} &=&mg(OC-ED)\\\Rightarrow\; v_{0} &=&\sqrt{v_{1}^{2}+2g(DE-OC)}\\\Rightarrow\; v_{0} &=&\sqrt{24^{2}+2\times 9.81(10.0-8.00)}\\\Rightarrow \;v_{0} &=&24.8\,m\cdot s^{-1} \end{array}$
5. D'après le théorème de l'énergie cinétique entre $E$ et $H$, on a :
$\dfrac{1}{2}mv_{H}^{2}-\dfrac{1}{2}mv_{1}^{2} =W_{EH}\left(\overrightarrow{F}\right)+W_{EH}\left(\overrightarrow{f}\right).$
Or $v_{H}=0\,m\cdot s^{-1}$
d'où il vient : $-\dfrac{1}{2}mv_{1}^{2}=-f\times EH-F\times EH.$
Donc $F=\dfrac{mv_{1}^{2}}{2EH}-f=\dfrac{1.00\cdot 10^{3}\times 24^{2}}{2\times 10.00}-500=23.8\cdot 10^{3}.$
6. La puissance du travail de la force $\overrightarrow{F}$
$\begin{array}{rcl} P &=&\dfrac{W_{EH}\left(\overrightarrow{F}\right)}{t}\\ &=&\dfrac{-F\times EH}{t}\\ &=&\dfrac{-23.8\cdot 10^{3}\times 10.00}{8.00}\\ &=& -29.8\cdot 10^{3}W. \end{array}$
Exercice 4

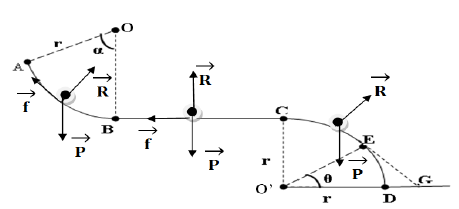
1. Calcul des vitesses $V_{B}$ et $V_{C}$ avec lesquelles le skieur passe en $B$ et en $C.$
$\bullet\ $Système étudié : le skieur
$\bullet\ $Référentiel d'étude : référentiel terrestre supposé galiléen
$\bullet\ $Bilan des forces appliquées : $\overrightarrow{P}$ et $\overrightarrow{R}$
Le théorème de l'énergie cinétique s'écrit :
$-\ $entre $A$ et $B$
$\begin{array}{rcl} E_{c_{B}}-E_{c_{A}}&=&W_{AB}\left(\overrightarrow{P}\right)+W_{AB}\left(\overrightarrow{R}\right)\\\Rightarrow\dfrac{1}{2}mV_{B^{2}}-0&=&mgr(1-\cos\alpha)+0\\\Rightarrow\dfrac{1}{2}mV_{B^{2}}&=&mgr(1-\cos\alpha)\\ \Rightarrow\;V_{B^{2}}&=&2gr(1-\cos\alpha)\\\Rightarrow\;V_{B}&=&\sqrt{2gr(1-\cos\alpha)}\\&=&\sqrt{2\times 10\times 5\left(1-\cos 60^{\circ}\right)}\\\Rightarrow\;V_{B}&=&7.07m\cdot s^{-1} \end{array}$
$-\ $entre $B$ et $C$
$\begin{array}{rcl} E_{c_{C}}-E_{c_{B}}&=&W_{BC}\left(\overrightarrow{P}\right)+W_{BC}\left(\overrightarrow{R}\right)\\\Rightarrow\dfrac{1}{2}mV_{C}^{2}-\dfrac{1}{2}mV_{B}^{2}&=&0+0\\\Rightarrow\;V_{C}^{2}&=&V_{B}^{2}\\\Rightarrow\;V_{C}&=&V_{B}\\\Rightarrow\;V_{C}&=&7.07m\cdot s^{-1} \end{array}$
2.1 Expression de $V_{B}$ en fonction de $m$, $r$, $f$, et $g.$
Le théorème de l'énergie cinétique s'écrit :
$-\ $entre $A$ et $B$
$\begin{array}{rcl} E_{c_{B}}-E_{c_{A}}&=&W_{AB}\left(\overrightarrow{P}\right)+W_{AB}\left(\overrightarrow{R}\right)+W_{AB}\left(\overrightarrow{f}\right)\\\Rightarrow\dfrac{1}{2}mV_{B}^{2}-0&=&mgr(1-\cos\alpha)+0-fr\alpha\text{ avec }\left(\alpha=\dfrac{\pi}{3}\right)\\ \Rightarrow\dfrac{1}{2}mV_{B}^{2}&=&mgr(1-\cos\alpha)-f\times\dfrac{\pi}{3}r\\ \Rightarrow\;V_{B}^{2}&=&2gr(1-\cos\alpha)-2f\times\dfrac{\pi}{3}r\\ \Rightarrow\;V_{B}&=&\sqrt{2gr\left(1-\cos\alpha\right)-2f\times\dfrac{\pi}{3}r} \end{array}$
2.2 Expression de $V_{c}$ en fonction de $m$, $r$, $f$ et $V_{B}$
Le théorème de l'énergie cinétique s'écrit :
$-\ $entre $B$ et $C$
$\begin{array}{rcl} E_{c_{C}}-E_{c_{B}}&=&W_{BC}\left(\overrightarrow{P}\right)+W_{BC}\left(\overrightarrow{R}\right)+W_{BC}\left(\overrightarrow{f}\right)\\ \Rightarrow\dfrac{1}{2}mV_{C}^{2}-\dfrac{1}{2}mV_{B}^{2}&=&0+0-fr\\ \Rightarrow\;V_{C}^{2}&=&2V_{B}^{2}-\dfrac{2fr}{m}\\\Rightarrow\;V_{C}&=&\sqrt{2\left(V_{B}^{2}-\dfrac{fr}{m}\right)} \end{array}$
2.3 Calcul de l'intensité de la force de frottement
$\begin{array}{rcl} V_{c}&=&\sqrt{2\left(V_{B}^{2}-\dfrac{fr}{m}\right)}\\&=&0\\ \Rightarrow\;2\left(V_{B}^{2}-\dfrac{fr}{m}\right)&=&0\\ \Rightarrow\dfrac{fr}{m}&=&V_{B}^{2}\\\Rightarrow\;f&=&\dfrac{mV_{B}^{2}}{r}\\&=&\dfrac{80\times 7.07^{2}}{5}\\\Rightarrow\;f&=&8.0\cdot 10^{2}N \end{array}$
3.1 Expression de la vitesse $V_{E}$ en fonction de $g$, $r$ et $\theta$
Le théorème de l'énergie cinétique s'écrit :
$-\ $entre $C$ et $E$
$\begin{array}{rcl} E_{c_{E}}-E_{c_{C}}&=&W_{CE}\left(\overrightarrow{P}\right)+W_{CE}\left(\overrightarrow{R}\right)\\\Rightarrow\dfrac{1}{2}mV_{E}^{2}-0&=&mgr(1-\sin\theta)\\\Rightarrow\;V_{E}^{2}&=&2gr(1-\sin\theta)\\\Rightarrow\;V_{E}&=&\sqrt{2gr(1-\sin\theta)} \end{array}$
3.2 Calcul de la valeur de l'angle $\theta$
$\begin{array}{rcl} V_{E}^{2}&=&2gr(1-\sin\theta)\\\Rightarrow(1-\sin\theta)&=&\dfrac{V_{E}^{2}}{2gr}\\ \Rightarrow\sin\theta&=&1-\dfrac{V_{E}^{2}}{2gr}\\\Rightarrow\theta&=&\sin^{-1}\left(1-\dfrac{V_{E}^{2}}{2gr}\right)\\\Rightarrow\theta&=&\sin^{-1}\left(1-\dfrac{5.77^{2}}{2\times 10\times 5}\right)\\ \Rightarrow\theta&=& 60^{\circ} \end{array}$
4. vitesse avec laquelle le skireur atterrit sur la piste de réception en un point $G$
$\begin{array}{rcl} V_{E}&=&\sqrt{2gr(1-\sin\theta)}\;,\text{ au point }G\;,\ \theta=0\\\Rightarrow\;V_{E}&=&\sqrt{2gr(1-\sin 0)}\\\Rightarrow\;V_{E}&=&\sqrt{2gr}\\&=&\sqrt{2\times10\times5}\\\Rightarrow\;V_{E}&=&10\,m\cdot s^{-1} \end{array}$

Commentaires
Ilyass elbari (non vérifié)
mer, 01/06/2021 - 22:01
Permalien
Problème
Mbaye Ndiaye (non vérifié)
mer, 02/03/2021 - 17:45
Permalien
Correction des exercices
sunudaara (non vérifié)
ven, 06/18/2021 - 18:40
Permalien
correction
Ndonazi (non vérifié)
mer, 01/31/2024 - 21:40
Permalien
Devenir un scientifique
sunudaara (non vérifié)
ven, 06/18/2021 - 18:40
Permalien
correction
Siby (non vérifié)
mer, 02/03/2021 - 20:48
Permalien
Reussit
BAH Salif (non vérifié)
mer, 02/17/2021 - 13:31
Permalien
correction des exercices 4 et plus
Ahmadou bamba Sagna (non vérifié)
jeu, 11/25/2021 - 14:10
Permalien
Je veux correction de l'exercice 4
Ahmadou bamba Sagna (non vérifié)
jeu, 11/25/2021 - 14:10
Permalien
Je veux correction de l'exercice 4
Babou cissé (non vérifié)
lun, 11/28/2022 - 23:12
Permalien
La correction de l'exercice 8
Papa Ousmane seck (non vérifié)
mar, 12/19/2023 - 20:05
Permalien
Fuiuggv
Babou cissé (non vérifié)
lun, 11/28/2022 - 23:13
Permalien
La correction de l'exercice 8
Doudou fall (non vérifié)
dim, 12/25/2022 - 17:37
Permalien
Éleve scientifique
Xavier (non vérifié)
ven, 12/08/2023 - 15:16
Permalien
École
Niang
mar, 01/02/2024 - 23:08
Permalien
Voir les exercices
Abdoulaye seck (non vérifié)
ven, 12/23/2022 - 20:59
Permalien
J’ai pas compris l’exercice 6
BAH Salif (non vérifié)
mer, 02/17/2021 - 13:30
Permalien
correction des exercices 4 et plus
BAH Salif (non vérifié)
mer, 02/17/2021 - 13:33
Permalien
correction des exercices 4 et plus
BAH Salif (non vérifié)
mer, 02/17/2021 - 13:34
Permalien
correction des exercices 4 et plus
BAH Salif (non vérifié)
mer, 02/17/2021 - 13:38
Permalien
correction des exercices 4 et plus
Mamadou ba (non vérifié)
dim, 02/28/2021 - 21:04
Permalien
L exo4
Choukaih (non vérifié)
mar, 03/09/2021 - 17:21
Permalien
Etudier
Haroun (non vérifié)
jeu, 05/19/2022 - 23:39
Permalien
Obtenir ces exercices corrigés
Mouhamed seck (non vérifié)
mer, 12/21/2022 - 21:15
Permalien
Correction alcane
Mously (non vérifié)
ven, 12/22/2023 - 20:03
Permalien
Exercice 6
Mouhammad (non vérifié)
jeu, 01/07/2021 - 07:54
Permalien
Eleve
Mr mbaye (non vérifié)
dim, 01/17/2021 - 13:24
Permalien
Pc
SEKONGO (non vérifié)
mer, 01/20/2021 - 15:42
Permalien
Atteindre le supérieur
Gora (non vérifié)
ven, 01/22/2021 - 13:40
Permalien
La correction n’est pas
Aliou Mamadou seck (non vérifié)
ven, 02/12/2021 - 11:59
Permalien
Élève du lycée de Richard toll
Millogo kalo (non vérifié)
mer, 03/10/2021 - 02:22
Permalien
Je veux télécharger les corrigés
Mouhamed (non vérifié)
ven, 03/12/2021 - 17:36
Permalien
étudier
Mouhamed (non vérifié)
ven, 03/12/2021 - 17:36
Permalien
Étudier
KOUMAKH SARR (non vérifié)
mar, 01/10/2023 - 14:23
Permalien
Travailler
KOUMAKH SARR (non vérifié)
mar, 01/10/2023 - 14:23
Permalien
Travailler
KOUMAKH SARR (non vérifié)
mar, 01/10/2023 - 14:23
Permalien
Travailler
Adamou haboubacar (non vérifié)
dim, 03/14/2021 - 21:52
Permalien
Exercice 4
Diagne (non vérifié)
lun, 04/12/2021 - 09:34
Permalien
reussite
Diagne (non vérifié)
lun, 04/12/2021 - 09:34
Permalien
reussite
ken (non vérifié)
lun, 07/19/2021 - 15:44
Permalien
eleve
Abeti (non vérifié)
sam, 10/16/2021 - 18:19
Permalien
Demande de téléchargement des corrigés
Issa Baldé (non vérifié)
mar, 03/22/2022 - 00:00
Permalien
Demande de telechargement des corrigés
Idriss (non vérifié)
jeu, 12/02/2021 - 11:56
Permalien
Commentaire
Mbayang (non vérifié)
dim, 12/05/2021 - 17:14
Permalien
Aide exo 1 6 eme question
Youssouf Ali Ab... (non vérifié)
lun, 01/24/2022 - 21:00
Permalien
Traité des exercices en physique et chimie
Youssouf Ali Ab... (non vérifié)
lun, 01/24/2022 - 21:00
Permalien
Traité des exercices en physique et chimie
Timan (non vérifié)
dim, 01/08/2023 - 09:18
Permalien
Les exercices corrigés
abi (non vérifié)
lun, 02/14/2022 - 19:14
Permalien
recherche
André Bampoky (non vérifié)
jeu, 03/03/2022 - 23:12
Permalien
Étudié
André Bampoky (non vérifié)
jeu, 03/03/2022 - 23:12
Permalien
Étudié
Pages
Ajouter un commentaire