Calcul intégral - T S
Classe:
Terminale
I Primitive
I.1 Définition
Soit I un intervalle de R et f une fonction continue sur I. On dit que F est une primitive de f sur I notée ∫f si, F est dérivable sur I et ∀x∈I, F′(x)=f(x)
Exemple
Soit f et F deux fonctions définies par :
f(x)=x2,F(x)={13x3six≤013x3−1six>0
F est-elle une primitive de f sur :
]−∞, 0] ?,[0, +∞[ ?,]0, +∞[ ?,R ?
Résolution
limx→0−F(x)−F(0)x−0=limx→0−13x3x=limx→0−13x2 =0
Donc, F est dérivable à gauche de 0.
limx→0+F(x)−F(0)x−0=limx→0+13x3−1x=limx→0+13x2−1x=−∞
Donc, F n'est pas dérivable à droite de 0.
F est dérivable sur ]−∞, 0] et F′(x)=x2=f(x), donc F est une primitive de f sur ]−∞, 0].
F n'est pas dérivable sur [0, +∞[, donc F n'est pas une primitive de f sur [0, +∞[.
F est dérivable sur ]0, +∞[ et F′(x)=f(x), donc F est une primitive de f sur ]0, +∞[.
F non dérivable sur R, donc F n'est pas une primitive de f sur R.
Théorème 1
Toute fonction continue sur I y admet une primitive.
Théorème 2
Si F est une primitive de f sur I, alors toute fonction G définie par G(x)=F(x)+c;c une constante est aussi une primitive de f sur I.
Donc deux primitives quelconques d'une fonction diffèrent d'une constante et il existe une unique primitive qui prend une valeur y en x0.
Théorème 3
Soit f une fonction dérivable sur I de dérivée f′ continue sur I et g une fonction continue sur I de primitive G définie sur un intervalle J contenant f(I), alors (g∘f)f′ a pour primitive sur I, G∘f
I.2 Opération sur les primitives
Si F est une primitive de f et G une primitive de g, alors
αF+βG est une primitive de αf+βg;α, β∈R
Tableau de quelques primitives
u et v sont deux fonctions continues et c une constante.
fFa∈Rax+c1x; x>0ln|x|+cexexsinx−cosx+ccosxsinx+c1√x; x>02√x1x2; x>0−1xfFxnxn+1n+1+c1cos2x=1+tan2xtanxu′unun+1n+1u′uln|u|u′eueuu′u2−1u
fFu′cosusinuu′sinu−cosuu′cos2u=1+tan2utanuu′√u2√uu′v+v′uuvλf; λ∈RλFu′v−uv′v2uv
Théorème
Si u et v sont dérivables sur I de dérivées u′ et v′ continues sur I, alors ∫u′v=uv−∫uv′
En effet, (uv)′=u′v+v′u⇒u′v=(uv)′−uv′⇒∫u′v=∫(uv)′−∫uv′⇒∫u′v=uv−∫uv′
Exemple
Déterminer les primitives de :
f(x)=xcosx,g(x)=xex,h(x)=lnx
Résolution
Posons u′=cosx ⇒ u=sinx et v=x ⇒ v′=1 donc, ∫f(x)=F(x)=xsinx−∫sinx=xsinx+cosx
On pose : u′=ex ⇒ u=ex et v=x ⇒ v′=1 alors, ∫g(x)=G(x)=xex−∫ex=xex−ex
Posons : u=x ⇒ u′=1 et v=lnx ⇒ v′=1x alors, ∫h(x)=H(x)=xlnx−∫1=xlnx−x
II Définition et propriétés
II.1 Définition
Soit I un intervalle de R, a, b∈I et f une fonction continue sur I. On appelle intégrale de a à b de f le réel noté ∫baf(x)dx=[F(x)]ba=F(b)−F(a)
où F est une primitive quelconque de f sur [a, b] ou [b, a]
Remarques
⋅ Le choix de la primitive F n'influe pas le résultat de l'intégrale. En effet, si F et G sont deux primitives d'une même fonction f sur I, alors elles différent d'une constante. Les quantités F(b)−F(a) et G(b)−G(a) sont donc égales. Soit G(x)=F(x)+c, alors ∫baf(x)dx=[G(x)]ba=G(b)−G(a)=F(b)+c−(F(a)+c)=F(b)−F(a)
⋅ Soit I un intervalle et x0∈I, Pour tout réel x de I, nous avons : ∫xx0f(t)dt=[F(t)]xx0=F(x)−F(x0) La fonction F définie sur I par F(x)=∫xx0f(t)dt est donc la primitive de f sur I qui s'annule en x0.
⋅ La lettre t ( ou x) choisie pour la variable est une variable "muette" ; elle peut être notée par toute autre lettre. Ce qui signifie que : ∫baf(x)dx=∫baf(t)dt=∫baf(u)du=F(b)−F(a)
Exemple
Calculer I=∫541x2−9dx,J=∫10xx+1dx,K=∫e1lnxxdx
Résolution
Soit I=∫541x2−9dx.
On a : 1x2−9=1(x−3)(x+3)=16(x−3)−16(x+3), alors I=∫541x2−9dx=∫54(16(x−3)−16(x+3))dx=[16ln|x−3|−16ln|x+3|]54=16ln2−16ln8−(16ln1−16ln7)=16ln2−16ln23+16ln7=16ln2−36ln2+16ln7=−13ln2+16ln7
Donc, I=∫541x2−9dx=−13ln2+16ln7
J=∫10xx+1dx. Or, xx+1=1−1x+1 donc, J=∫10xx+1dx=∫10(1−1x+1)dx=[x−ln|x+1|]10=1−ln2
Donc J=∫10xx+1dx=1−ln2
K=∫e1lnxxdx=[12ln2|x|]e1=12ln2e−0=12
Donc, K=∫e1lnxxdx=12
II.2 Propriétés
Soit I un intervalle de R, a, b, c∈I, f et g deux fonctions continues sur I, α, β∈R, alors
⋅ ∫aaf(x)dx=0
⋅ ∫baf(x)dx=−∫abf(x)dx
⋅ Relation de Chasles ∫baf(x)dx=∫caf(x)dx+∫bcf(x)dx
⋅ Linéarité de l'intégration ∫ba(αf+βg)(x)dx=α∫baf(x)dx+β∫bag(x)dx
⋅ Si f est paire, alors ∫a−af(x)dx=2∫a0f(x)dx
⋅ Si f est impaire, alors ∫a−af(x)dx=0
⋅ Si f est périodique de période T, alors ∫a+Taf(x)dx=∫T0f(x)dx
⋅ Positivité de l'intégration : Si a≤b et f≥0 sur [a, b], alors ∫baf(x)dx≥0
⋅ Conservation de l'ordre de l'intégration : Si a≤b et f≤g sur [a, b], alors ∫baf(x)dx≤∫bag(x)dx
⋅ |∫baf(x)dx|≤∫ba|f(x)|dx
⋅ Inégalité de la moyenne : La fonction f étant continue sur [a, b], alors il existe deux réels m et M tels que, pour tout réel x∈[a, b] on ait m≤f(x)≤M et donc : m(b−a)≤∫baf(x)dx≤M(b−a)
En effet m≤f(x)≤M⇒∫bamdx≤∫baf(x)dx≤∫baMdx⇒m(b−a)≤∫baf(x)dx≤M(b−a)
Théorème de la moyenne
Pour toute fonction f définie et continue sur l'intervalle [a, b], d'après le théorème des valeurs intermédiaires, il existe au moins un réel c∈[a, b] tel que
f(c)=1b−a∫baf(x)dx Le réel f(c) est appelé valeur moyenne de f sur [a, b].
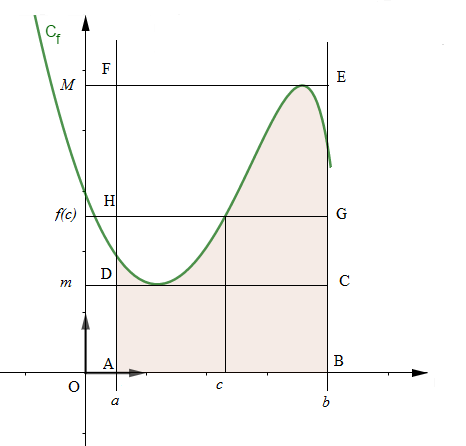
Interprétations géométriques
Le plan est muni d'un repère orthogonal (O; →i, →j). Soit f une fonction continue et positive de courbe représentative (Cf), alors
⋅ L'encadrement m(b−a)≤∫baf(x)dx≤M(b−a) signifie que l'aire du domaine coloré est minorée par l'aire du rectangle ABCD, et majorée par celle du rectangle ABEF.
⋅ L'égalité f(c)=1b−a∫baf(x)dx signifie que l'aire du domaine coloré est égale à celle du rectangle ABGH.
Exercice d'application
Soit la suite (un) définie par un=∫e1x(lnx)ndx Montrer que un est décroissante.
Résolution
un+1−un=∫e1x(lnx)n+1dx−∫e1x(lnx)ndx=∫e1(x(lnx)n+1−x(lnx)n)dx=∫e1x(lnx)n(lnx−1)dx
Or, x≤e ⇒ lnx≤1, donc lnx−1≤0. De plus x(lnx)n>0.
Alors, x(lnx)n(lnx−1)<0 et donc ∫e1x(lnx)n(lnx−1)dx<0 d'où un+1−un<0 ce qui signifie que (un) est décroissante.
III Méthodes d'intégration
III.1 A l'aide du tableau de primitives
Exemple
Calculer I=∫10x√1−x2dx,J=∫π60sin2xcos3xdx
Posons u=1−x2 ⇒ u′=−2x, donc x√1−x2=−12u′u1/2.
Or, u′u1/2 a pour primitive 23u3/2 donc −12(23u3/2)=−13u3/2 est primitive de −12u′u1/2.
Donc, I=∫10x√1−x2dx=[−13(1−x2)√1−x2]10=13
On a : sin2xcos3x=12[sin5x+sin(−x)] donc, J=∫π60sin2xcos3xdx=∫π60(12sin5x−12sinx)dx=[−110cos5x+12cosx]π60=√320+√34+110−12=6√320−410
Donc, J=∫π60sin2xcos3xdx=3√310−25
III.2 A l'aide de la linéarisation
Exemple
Calculer I=∫π0sin5xdx
On a sin5x=(eix−e−ix2i)5,
On a sin5x=(eix−e−ix2i)5,
après développement (chapitre - nombres complexes) on obtient sin5x=116(sin5x−5sin3x+10sinx),
donc, I=∫π0sin5xdx=∫π0116(sin5x−5sin3x+10sinx)dx=[−180cos5x+548cos3x−58cosx]π0=180−548+58+180−548+58=140−524+54
III.3 Par changement de variables
Théorème
Si g est continue sur [a, b] et de dérivée g′ continue sur [a, b].
Si f est continue sur [α, β] avec α=g(a) et β=g(b), alors ∫baf∘g(x)g′(x)dx=∫βαf(u)du
Exemple
Calculer A=∫π40tanxdx,B=∫π0sinxcos4xdx
Résolution
Soit A=∫π40tanxdx=∫π40sinxcosxdx
Posons : u=cosx ⇒ du=−sinxdx, alors u′=dudx ⇒ du=u′dx et donc A=∫π40tanxdx=∫√221−duu=[−ln|u|]√2/21=−ln1√2=ln√2 = 12ln2
Posons : u=cosx ⇒ du=−sinxdx, alors u′=dudx ⇒ du=u′dx et donc A=∫π40tanxdx=∫√221−duu=[−ln|u|]√2/21=−ln1√2=ln√2 = 12ln2
Soit B=∫π0sinxcos4xdx
Posons : u=cosx ⇒ du=−sinxdx,
alors, B=∫π0sinxcos4xdx=∫−11−u4du=∫1−1u4du=2∫10u4du car g(u)=u4 est paire=2[15u5]10=25
Posons : u=cosx ⇒ du=−sinxdx,
alors, B=∫π0sinxcos4xdx=∫−11−u4du=∫1−1u4du=2∫10u4du car g(u)=u4 est paire=2[15u5]10=25
Remarque
∫baαf(αt+β)dt=∫αb+βαa+βf(u)du
u=αt+β ⇒ du=αdt
III.4 Intégration par parties
Si u et v sont dérivables sur I de dérivées u′ et v′ continues sur I et a, b∈I, alors ∫bau′(x)v(x)dx=[u(x)v(x)]ba−∫bau(x)v′(x)dx
Exemple
Calculer les intégrales suivantes A=∫π0xcosxdx,B=∫e1xlnxdx
Résolution
Posons : u′=cosx ⇒ u=sinx et v=x ⇒ v′=1, donc A=∫π0xcosxdx=[xsinx]π0−∫π0sinxdx=0+[cosx]π0=−2
On pose u′=x ⇒ u=x22 et v=lnx ⇒ v′=1x,
alors, B=∫e1xlnxdx=[x22lnx]e1−∫e1x2dx=e22−[x24]e1=e22−e24+14=e2+14
IV Calcul approché d'une intégrale
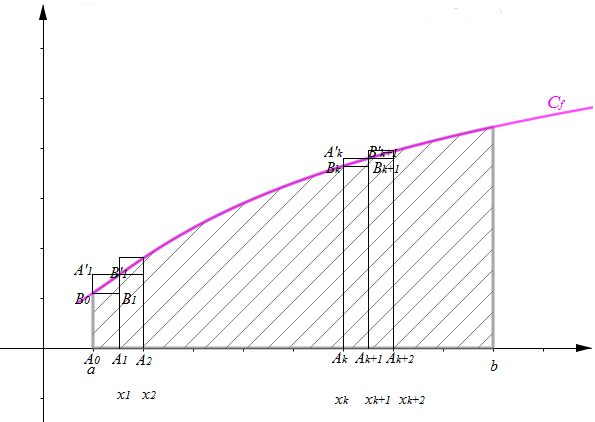
Méthode des rectangles
Soit I=[a, b] un intervalle (a<b) de R, f une fonction continue strictement croissante et positive sur I.
∫baf(x)dx est la surface du plan ou l'aire du domaine comprise entre la courbe Cf, l'axe (x′Ox) et les droites d'équations x=a et x=b.
On subdivise [a, b] en n segments de même longueur b−an, on a donc :
x0=a, x1=a+b−an, x2=a+2(b−a)n,…, xk=a+k(b−a)n et xn=a+n(b−a)n=b.
On a ainsi un découpage régulier de l'intervalle I car ∀k∈[0, (n−1)], xk+1−xk=b−an.
Soit sn la somme des aires des rectangles AkAk+1Bk+1Bk et Sn la somme des aires des rectangles AkAk+1B′k+1A′k,0≤k≤n−1.
L'aire du domaine sous la courbe est donc encadrée par deux suites adjacentes d'aires de rectangles associés à une subdivision de l'intervalle [a, b],
on a alors sn≤∫baf(x)dx≤Sn avec : sn=f(a)(b−an)+f(a+b−an)×b−an+…+f(a+(n−1)(b−a)n)×b−an=b−ann−1∑k=0f(a+k(b−a)n) donc sn=b−ann−1∑k=0f(a+k(b−a)n)
Sn=f(a+b−an)×b−an+f(a+2(b−a)n)×b−an+…+f(a+(n−1)(b−a)n)×b−an+f(b)×b−an=b−ann∑k=1f(a+k(b−a)n) donc, Sn=b−ann∑k=1f(a+k(b−a)n)
Autre méthode
Sur [xk, xk+1], f est croissante alors, f(xk)≤f(x)≤f(xk+1) et donc ∫xk+1xkf(xk)dx≤∫xk+1xkf(x)dx≤∫xk+1xkf(xk+1)dxf(xk)(xk+1−xk)≤∫xk+1xkf(x)dx≤f(xk+1)(xk+1−xk)b−anf(a+k(b−a)n)≤∫xk+1xkf(x)dx≤b−anf(a+(k+1)(b−a)n)b−ann−1∑k=0f(a+k(b−a)n)≤n−1∑k=0∫xk+1xkf(x)dx≤b−ann−1∑k=0f(a+(k+1)(b−a)n)b−ann−1∑k=0f(a+k(b−a)n)≤∫baf(x)dx≤b−ann∑k=1f(a+k(b−a)n)
Ainsi, sn≤∫baf(x)dx≤Sn
Sn+sn2 est une valeur approchée de ∫baf(x)dx à Sn−sn2 près.
Sn−sn2=(f(b)−f(a))(b−an)
Donc en subdivisant de plus en plus finement l'intervalle [a, b], les suites sn et Sn convergent vers un même nombre, ce nombre est l'aire sous la courbe Cf.
limn→+∞sn=limn→+∞Sn=∫baf(x)dx
V Fonction définie par une intégrale
⋅ Soit I un intervalle de r et a, b∈R, l'intégrale ∫baf(x)dx existe si f est continue sur [a, b].
⋅ Soit f une fonction continue sur I, pour tous a, x∈I la fonction F définie par F(x)=∫xaf(t)dt est une fonction définie par une intégrale.
⋅ f est continue sur I, alors f admet une primitive G sur I, donc F(x)=[G(t)]ax=G(x)−G(a). Ainsi, F′(x)=G′(x)=f(x)
Exemple
Soit F la fonction définie par F(x)=∫x01√1+t2dt
On a 1√1+t2 continue sur R, donc F est définie sur R et ∀−x∈R, F(−x)=∫−x01√1+t2dt=−∫x01√1+u2du=−F(x) après changement de variables u=−t ⇒ du=−dt, donc F est impaire.
1√1+t2 est continue sur R, donc admet une primitive F1 et donc
F(x)=F1(x)−F1(0) ⇒ F′(x)=F′1(x)=1√1+x2>0, donc F est strictement croissante. On a :
1+t2≥t2⇒1√1+t2≤1t2⇒0≤∫x01√1+t2dt≤∫x01t2dt⇒0≤F(x)≤[−1t]x0⇒0≤F(x)≤−1x+1
Ainsi, 0≤limx→+∞F(x)≤1
⋅ f continue sur R, u(x) et v(x) dérivable sur R, alors
F(x)=∫v(x)u(x)f(t)dt existe si, et seulement, u(x) et v(x) existent et appartiennent au domaine de continuité de f.
f continue sur R donc admet une primitive F1, alors F(x)=[F1(t)]v(x)u(x)=F1(v(x))−F1(u(x))
F1 dérivable sur R, alors F1(u(x)) est dérivable sur R, idem pour F1(v(x)), d'où F dérivable sur R.
Donc F′(x)=v′(x)F′1(v(x))−u′(x)F′1(u(x))=v′(x)f(v)−u′(x)f(u)
VI Calcul d'aire et de volume
VI.1 Calcul d'aires
Soit (O; →i, →j) un repère orthogonal, ||→i||=mcm, ||→j||=ncm.
L'unité d'aire est l'aire du quadrilatère construit sur les vecteurs de bases du repères. 1 unité d'aire (u.a)=m×ncm2
⋅ Soit f≥0 sur [a, b].
L'aire A de la partie du plan comprise entre Cf, l'axe des abscisses et les droites d'équation x=a et x=b est égale à : A=∫baf(x)dxu.a
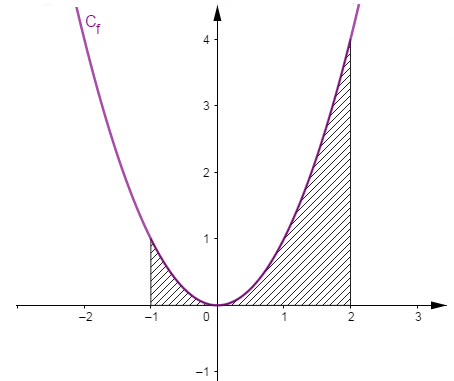
Exemple
Soit (O; →i, →j) un repère orthogonal, ||→i||=||→j||=1cm. On considère la parabole Cf représentant la fonction f définie sur R par f(x)=x2.
Calculer l'aire A en cm2 du domaine délimité par Cf, l'axe des abscisses et les droites d'équation x=−1 et x=2
Résolution
On a 1u.a=1cm2
A=∫2−1f(x)dx=∫2−1x2dx=[x33]2−1=83+13=3cm2
⋅ Soit f≥0 sur [a, b].
L'aire de la partie du plan comprise entre Cf, l'axe des abscisses et les droites d'équation x=a et x=b est égale à : ∫ba−f(x)dxu.a
Exemple
Soit f la fonction définie sur R par f(x)=−x2.
Calculer l'aire A du domaine délimité par Cf, l'axe des abscisses et les droites d'équation x=−1 et x=2
Résolution
A=∫2−1−f(x)dx=∫2−1x2dx=[x33]2−1=83+13=3cm2

⋅ Soit f et g deux fonctions continues sur [a, b].
L'aire de la partie du plan comprise entre Cf, Cg et les droites d'équation x=a et x=b est égale à : ∫ba|f(x)−g(x)|dxu.a
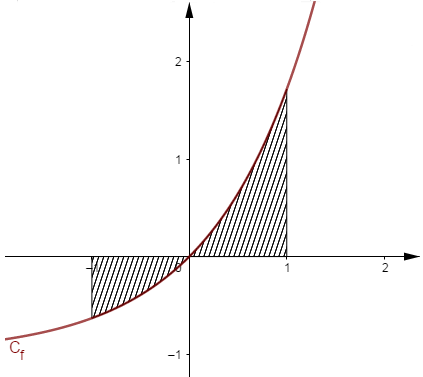
Exemple : cas particulier d'une fonction changeant de signe
Soit f et g les fonctions définies sur R par f(x)=ex−1 et g(x)=0
Calculer l'aire A du domaine délimité par Cf, Cg et les droites d'équation x=−1 et x=1
Résolution
A=∫1−1|f(x)−g(x)|dx=∫1−1|ex−1|dx=∫0−1−(ex−1)dx+∫10ex−1dx=−[ex−x]0−1+[ex−x]10=−[1−1e−1]+[e−1−1]=1e+e−2
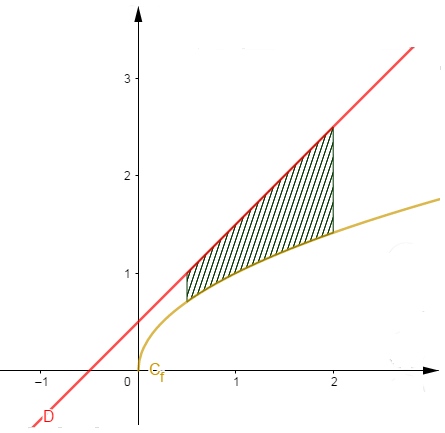
⋅ Soit f une fonction continue sur [a, b] et D une droite d'équation αx+β.
L'aire de la partie du plan comprise entre Cf, D et les droites d'équation x=a et x=b est égale à : ∫ba|f(x)−(αx+β)|dxu.a
Exemple
Soit f la fonction définie sur R+ par f(x)=√x et D : x+12
Calculer l'aire A du domaine délimité par Cf, D et les droites d'équation x=12 et x=2
A=∫21/2|f(x)−(αx+β)|dx=∫21/2(x+12−√x)dx=[x22+12x−x3/232]21/2=(2+1−4√23)−(18+14−√26)=3−38−7√26
VI.2 Calcul de volume
Soit (O\;;\ \vec{i}\;,\ \vec{j}\;,\ \vec{k}) un repère orthogonal.
L'unité de volume est le volume du parallélépipède construit sur les vecteurs de bases du repères.
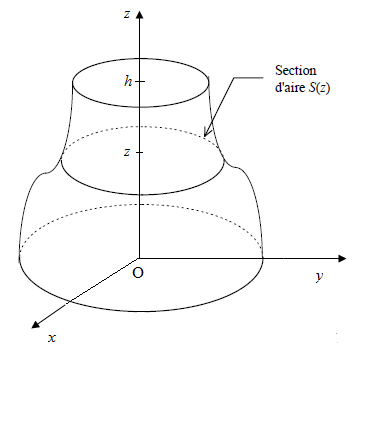
Soit S un solide de hauteur h. Un plan \mathcal{P} parallèle au plan (x'Ox) coupe S suivant une figure plane de surface S(z). Le volume \mathcal{V} de S est égal à \mathcal{V}=\int_{0}^{h}S(z)\mathrm{d}z\;u.v
1\;u.v=\text{une unité de volume}
Exemples
a) Cylindre (R\;,\ h)
S(z)=\pi R^{2} \begin{eqnarray} \mathcal{V}&=&\int_{0}^{h}S(z)\mathrm{d}z\nonumber \\ \\&=&\int_{0}^{h}\pi R^{2}\mathrm{d}z\nonumber \\ \\&=&[\pi R^{2}]_{0}^{h}\nonumber \\ \\&=&\pi R^{2}h\nonumber \end{eqnarray}

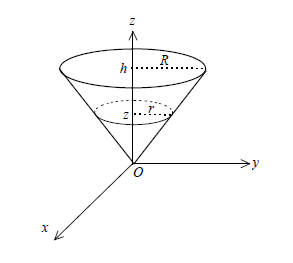
b) Cône (R\;,\ h)
S(z)=\pi r^{2}, d'après Thalès \dfrac{r}{R}=\dfrac{z}{h}, donc S(z)=\pi\dfrac{R^{2}}{h^{2}}z^{2}.
Donc \begin{eqnarray} \mathcal{V}&=&\int_{0}^{h}S(z)\mathrm{d}z\nonumber \\ \\&=&\int_{0}^{h}\pi\dfrac{R^{2}}{h^{2}}z^{2}\mathrm{d}z\nonumber \\ \\&=&\pi\dfrac{R^{2}}{h^{2}}\int_{0}^{h}z^{2}\mathrm{d}z\nonumber \\ \\&=&\pi\dfrac{R^{2}}{h^{2}}\left[\dfrac{z^{3}}{3}\right]_{0}^{h}\nonumber \\ \\&=&\pi\dfrac{R^{2}h}{3}\nonumber \end{eqnarray}
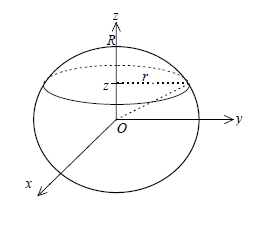
c) Sphère de rayon R
S(z)=\pi r^{2} or r^{2}+z^{2}=R^{2}\ \Rightarrow\ r^{2}=R^{2}-z^{2}, donc S(z)=\pi(R^{2}-z^{2}).
Alors \begin{eqnarray} \mathcal{V}&=&\int_{-R}^{R}S(z)\mathrm{d}z\nonumber \\ \\&=&\int_{-R}^{R}\pi(R^{2}-z^{2})\mathrm{d}z\nonumber \\ \\&=&2\pi\int_{0}^{R}(R^{2}-z^{2})\mathrm{d}z\nonumber \\ \\&=&2\pi\left[R^{2}z-\dfrac{z^{3}}{3}\right]_{0}^{R}\nonumber \\ \\&=&2\pi\left(R^{3}-\dfrac{R^{3}}{3}\right)\nonumber \\ \\&=&\dfrac{4}{3}\pi R^{3}\nonumber \end{eqnarray}
Remarque
Soit f une fonction continue sur [a\;,\ b], alors le volume engendré par la rotation de C_{f}\;,\ x=a et x=b autour de l'axe des abscisses est égal à \mathcal{V}=\int_{a}^{b}\pi(f(x))^{2}\mathrm{d}x
Auteur:
Diny Faye & Seyni Ndiaye

Commentaires
Anonyme (non vérifié)
sam, 07/27/2019 - 13:51
Permalien
Merci à vous pour tout ce que
M Pouye (non vérifié)
lun, 01/11/2021 - 07:22
Permalien
C'est très intéressant, merci
Anonyme (non vérifié)
lun, 04/17/2023 - 18:20
Permalien
ghferuipguitg-ioiuug tefg"o
Ajouter un commentaire