Fonction Ln - TL
Classe:
Terminale
1. Définition
Nous admettrons qu'il existe une seule fonction F ayant les trois propriétés suivantes :
∙ Elle n'est définie que pour x>0, soit sur ]0 ; +∞[ ;
∙ Elle s'annule pour x=1, soit F(1)=0.
∙ Elle admet pour fonction dérivée F′ telle que F′(x)=1x∀x>0.
Cette fonction est appelée fonction logarithme népérien (notée en abrégé ln).
2. Propriétés fondamentales
1. ∀a>0, ∀b>0, lnab=lna+lnb.
2. ∀a>0, ∀b>0, lnab=lna−lnb
3. ∀n∈Z, ∀a>0, lnan=nlna
cas particulier :
∀a>0, ln1a=−lna.
4. ∀a>0, =ln√a=12lna.
∙ Limites importantes
limx⟶0+lnx=−∞
limx⟶+∞lnx=+∞
limx⟶0+xlnx=0
limx⟶+∞lnxx
limx⟶0+ln(1+x)x=1.
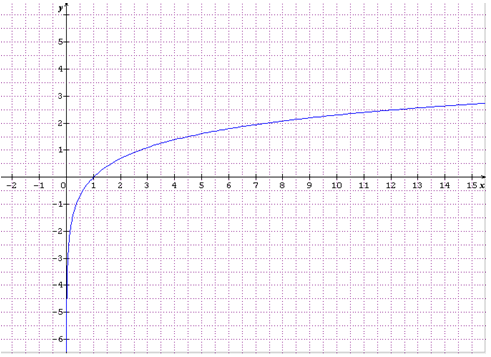
4. Représentation graphique
Par définition, la fonction ln est dérivable sur R+∗ et on a :
(lnx)′=1x∀x>0
Tableau de variation
Tableau
Tableau de valeurs
x0.10.30.5123lnx−2.303−1.204−0.69300.6931.099
x456789lnx1.3861.6091.7921.9462.0792.197
Exercice d'application :
Donner les équations des tangentes à C, courbe représentative de ln aux points d'abscisse respectives 1 et e
Propriété admise
La fonction la réalise une bijection de ]0 ; +∞[ vers R.
Cela signifie que pour tout réel λ l'équation f(x)=λ admet une solution et une seule.
En particulier, il existe un nombre et un seul, noté e tel que : lne=1.
e≈2.7182818…
Une autre conséquence de cette propriété est qu'on a les équivalences suivantes :
1. ∀a>0, ∀b>0,lna=lnb⇔a=b
2. ∀a>0, ∀b>0,lna>lnb⇔a>b
3. ∀a>0, ∀b>0,lna<lnb⇔a<b
4. (lnx)<0⇔(0<x<1)
5. (lnx)=0⇔(x=1)
6. (lnx)>0⇔(x>1).
7. ∀m∈Z, lnx=m⇔(x=em) car lnem=mlne=m

Commentaires
Anonyme (non vérifié)
ven, 04/26/2024 - 12:43
Permalien
J’apprécie énormément
Ajouter un commentaire