Repérage dans le plan 3e
Classe:
Troisième
I. repérage orthonormal
Soient →i et →j deux vecteurs du plan de direction respective (x′x) et (y′y) non parallèles et O leur point d'intersection.
On appelle repère cartésien, le triplet (O; →i, →j).
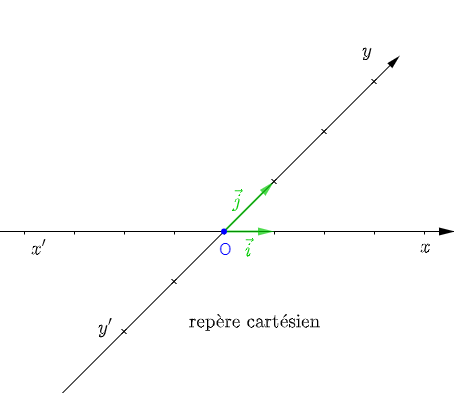
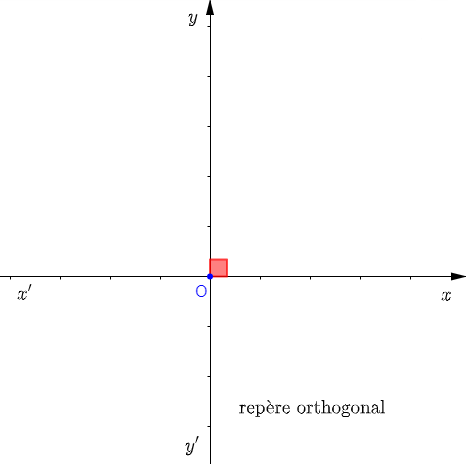
Si (x′x)⊥(y′y) alors, le repère cartésien sera le repère orthogonal.
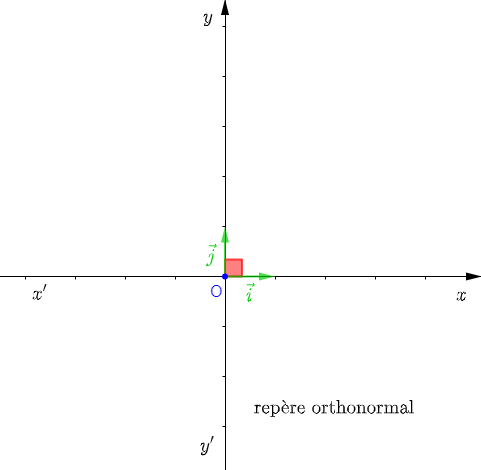
Si, pour le repère orthogonal, on a ℓ→i=ℓ→j alors, le repère sera dit repère orthonormal.
Le point O est appelé origine du repère et les axes (x′x) et (y′y)sont respectivement appelés axe des abscisses et axe des ordonnées.
Remarque :
En posant →i=→OI et →j=→OJ, le repère orthonormal sera noté (O; I, J)
II. Coordonnées d'un point dans un repère orthonormal
Soient (O; I, J) un repère orthonormal et M un point du plan.
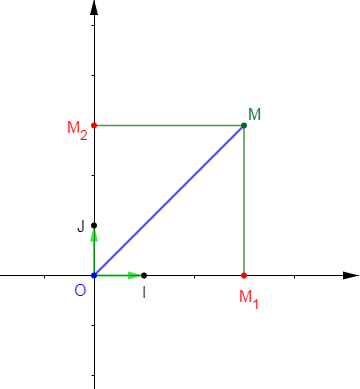
On a : OM1MM2 un parallélogramme alors, →OM=→OM1+→OM2.
Or, les vecteurs →OM1 et →OM2 sont respectivement colinéaires aux vecteurs →i et →j.
Donc, il existe deux réels x et y tels que →OM1=x.→i et →OM2=y.→j.
Ainsi, →OM=x.→i+y.→j. D'où le couple (x; y) est appelé les coordonnées du point M dans le repère orthonormal (O; →i, →j).
On notera M(xy) ou M(x; y)
Si M a pour coordonnées (xy) dans le repère orthonormal (O; →i, →j) alors, →OM=x.→i+y.→j.
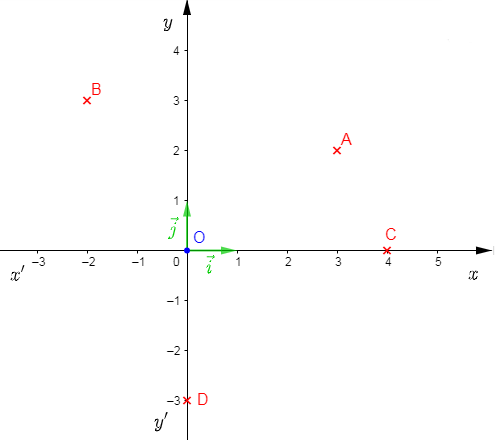
Exemple :
On considère un repère orthonormal (O; →i, →j) et on donne :
→OA=3→i+2→j; →OB=−2→i+3→j; →OC=4→i et →OD=−3→j.
Trouvons les coordonnées des points A, B, C et D et plaçons les dans le repère orthonormal.
On a : →OA=3→i+2→j alors, A(32)
→OB=−2→i+3→j alors, B(−2; 3)
→OC=4→i alors, C(40)
→OD=−3→j alors, D(0; −3)
Les réels x et y sont appelés respectivement abscisse et ordonnée du point M dans le repère orthonormal (O; →i, →j).
⋅ Le point O a pour coordonnées (00), on notera O(00)
⋅ Si un point a pour abscisse nul, il se trouvera alors dans l'axe des ordonnées.
⋅ Si un point a pour ordonnée nulle, il se trouvera alors dans l'axe des abscisses.
III. Coordonnées d'un vecteur dans un repère orthonormal
III.1 Coordonnées d'un vecteur représenté par un bipoint.
Soient (O; →i, →j) un repère orthonormal, A et B deux points de ce repère.

On a : →OA=xA→i+yA→j et →OB=xB→i+yB→j
et comme →AB=(xB→i+yB→j)−(xA→i+yA→j)
alors, →AB=xB→i+yB→j−xA→i−yA→j.
Ainsi, →AB=(xB−xA)→i+(yB−yA)→j.
Si A(xAyA) et B(xByB) dans un repère orthonormal alors, →AB(xB−xAyB−yA)
Exemple : on considère un repère orthonormal (O; →i, →j) et on donne :
→OA=2→i+3→j; →OB=−3→i+2→j et →OC=−→i−4→j.
Calculons les coordonnées des vecteurs →AB, →AC et →BC
On a : A(23), B(−32) et C(−1−4)
alors, →AB(−3−22−3)=(−5−1), →AC(−1−2−4−3)=(−3−7)
et →BC(−1−(−3)−4−2)=(2−6)
III.2 Coordonnées d'un vecteur somme
Soient →u(xy) et →v(x′y′) deux vecteurs dans un repère orthonormal (O; →i, →j).
On aura : →u=x.→i+y.→j et →v=x′.→i+y′.→j
Alors, →u+→v=(x.→i+y.→j)+(x′.→i+y′.→j).
Donc, →u+→v=x.→i+y.→j+x′.→i+y′.→j.
Ainsi, →u+→v=(x+x′).→i+(y+y′).→j.
Si →u(xy) et →v(x′y′) dans un repère orthonormal alors, →u+→v(x+x′y+y′)
III.3 Coordonnées d'un vecteur égal à un autre
Soient →u(xy) et →v(x′y′) deux vecteurs dans un repère orthonormal (O; →i, →j).
On se propose de trouver la condition nécessaire et suffisante pour que les vecteurs →u et →v soient égaux.
On a : →u=x.→i+y.→j et →v=x′.→i+y′.→j
or, →u=→v
alors, x.→i+y.→j=x′.→i+y′.→j.
Donc, x=x′ et y=y′
Si →u(xy) et →v(x′y′) dans un repère orthonormal et →u=→v alors, x=x′ et y=y′ et réciproquement.
III.4 Coordonnées d'un vecteur opposé à un autre
Soient →u(xy) et →v(x′y′) deux vecteurs dans un repère orthonormal (O; →i, →j).
On se propose de trouver la condition nécessaire et suffisante pour que les vecteurs →u et →v soient des vecteurs opposés.
On a : →u=x.→i+y.→j et →v=x′.→i+y′.→j
or, →u+→v=→0
alors, x.→i+y.→j+x′.→i+y′.→j=→0.
Donc, (x+x′).→i+(y+y′).→j=0.→i+0.→j
Ainsi, x+x′=0 et y+y′0
d'où, x=−x′ et y=−y′
Si →u(xy) et →v(x′y′) dans un repère orthonormal et →u+→v=→0 alors, x=−x′ et y=−y′ et réciproquement.
III.5 Coordonnées d'un vecteur multiplié par un réel
Soient →u(xy) et →v(x′y′) deux vecteurs dans un repère orthonormal (O; →i, →j) et k∈R tel que →v=k.→u.
On a : →u=x.→i+y.→j et →v=x′.→i+y′.→j
or, →v=k.→u.
alors, x′.→i+y′.→j=k(x.→i+y.→j).
Donc, x′.→i+y′.→j=(kx).→i+(ky).→j.
D'où, x′=kx et y′=ky
Si →u(xy) dans un repère orthonormal et k∈R alors, k→u(kxky)
III.6 Condition de colinéarité de deux vecteurs
Soient →u(xy) et →v(x′y′) deux vecteurs colinéaires dans un repère orthonormal (O; →i, →j).
Alors on a : →v=k.→u avec k∈R.
Donc, x′=kx et y′=ky, par suite x′y′=kxky.
Par conséquent, x′y′=xy.
Ainsi, xy′=x′y ; d'où, xy′−x′y=0
Si →u(xy) et →v(x′y′) dans un repère orthonormal et →u, →v colinéaires alors, xy′−x′y=0.
Réciproque : Si →u(xy) et →v(x′y′) deux vecteurs dans un repère orthonormal et xy′−x′y=0 alors, →u et →u colinéaires.
III.7 Applications
⋅ Coordonnées du milieu d'un segment
Soient A(xAyA) et B(xByB) dans un repère orthonormal (O; →i, →j) et I(xIyI) milieu de [AB].
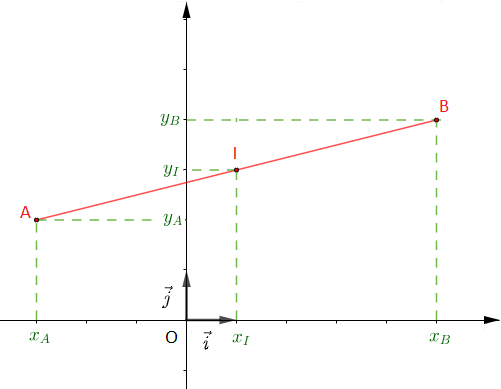
On se propose de déterminer les coordonnées du point I en fonction des coordonnées des points A et B.
On a : I milieu de [AB] alors, →AI=→IB.
Or, →AI(xI−xAyI−yA) et →IB(xB−xIyB−yI)
Donc, xI−xA=xB−xI et yI−yA=yB−yI.
Ainsi, 2xI=xA+xB et 2yI=yA+yB
D'où, xI=xA+xB2 et yI=yA+yB2.
Si A(xAyA) et B(xByB) dans un repère orthonormal (O; →i, →j) et I(xIyI) milieu de [AB] alors, I(xA+xB2yA+yB2)
Exemple : on considère un repère orthonormal (O; →i, →j) et on donne A(−12) et B(54).
On a : I milieu de [AB] alors, I((−1)+522+42) ; donc, I(23).

On considère un repère orthonormal (O; →i, →j) et on donne A(−12), B(34) et C(5y).
Déterminons la valeur de y pour que les points A, B et C soient alignés.
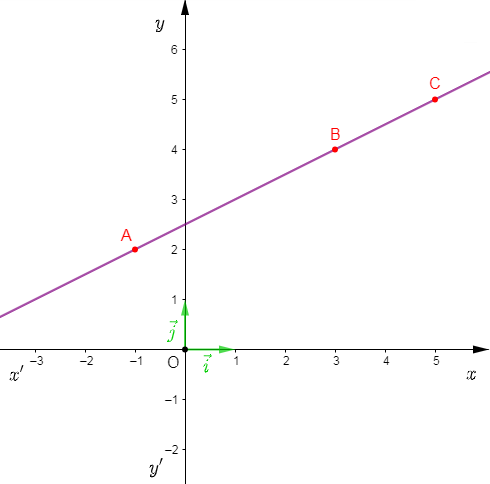
1er méthode :
On a : A, B et C alignés alors, →AC=k.→AB.
Or, →AC(6y−2); →AB(42) et k.→AB(4k2k)
Donc, 6=2k et y−2=2k
Ainsi, k=32 et y−2=2(32) ; d'où, y=5.
2em méthode :
On a : A, B et C alignés alors, →AB et →AB colinéaires.
Or, →AB(42) et →AC(6y−2)
Donc, 4(y−2)−2(6)=0
Ainsi, 4y−8−12=0
D'où, y=5
⋅ Détermination du quatrième point d'un parallélogramme
On considère un repère orthonormal (O; →i, →j) et on donne A(35), B(−22) et C(−43).
Déterminons les coordonnées du point D telles que ABCD soit un parallélogramme.
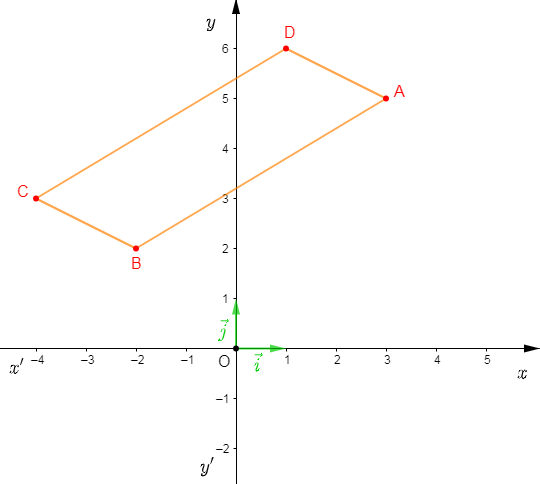
On a : ABCD parallélogramme alors, →CD=→BA.
Or, →CD(xD+4yD−3) et →BA(53)
Donc, xD+4=5 et yD=6
D'où, D(16).
⋅ Détermination des coordonnées du centre de gravité d'un triangle
On considère un repère orthonormal (O; →i, →j) et on donne A(−33), B(63) et C(36).
Déterminons les coordonnées du point G centre de gravité du triangle ABC.
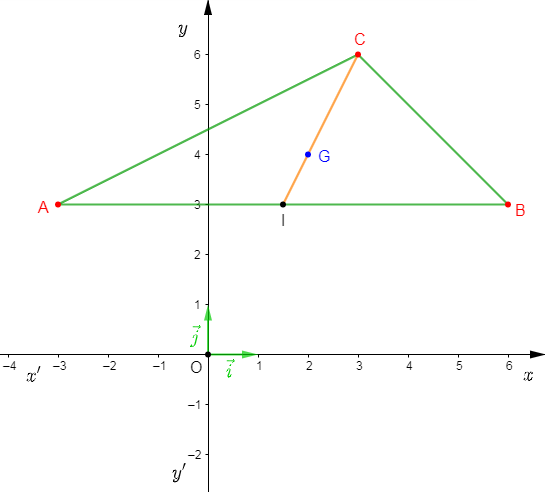
1er méthode :
On a : G centre de gravité de ABC alors, →GA+→GB+→GC=→0.
Or, →GA(−3−xG3−yG); →GB(6−xG3−yG) et →GC(3−xG6−yG)
Donc, (→GA+→GB+→GC)(6−3xG12−3yG) et →0(00)
Par suite, 6−3xG=0 et 12−3yG=0
Ainsi, xG=2 et yG=4
D'où, G(24)
2em méthode :
On a : G centre de gravité de ABC et I milieu de [AB].
Alors, →IC=3→IG
Or, I(323), →IC(323), →IG(xG−32yG−3) et 3→IG(3xG−923yG−9)
Donc, 32=3xG−92 et 3=3yG−9
Ainsi, xG=2 et yG=4
D'où, G(24)
IV. Distance de deux points dans un repère orthonormal
Soient A(xAyA) et B(xByB) deux points dans un repère orthonormal (O; →i, →j)
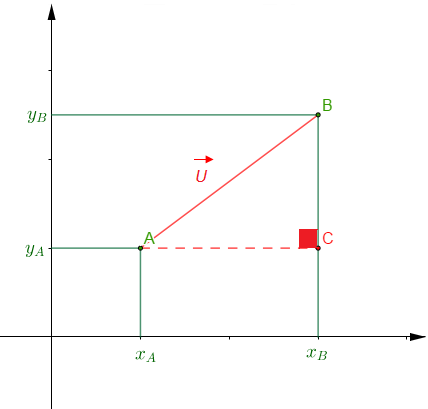
On a : ABC un triangle rectangle en C. D'après le théorème de Pythagore, on aura : AB=√AC2+CB2
Or, AC2=(xB−xA)2 et CB2=(yB−yA)2
Donc, AB=√(xB−xA)2+(yB−yA)2
Si A(xAyA) et B(xByB) sont deux points dans un repère orthonormal alors, AB=√(xB−xA)2+(yB−yA)2.
En posant →AB=→u(xy) alors, xB−xA=x et yB−yA=y
Donc, L→u=AB=√x2+y2
Si →AB(xy) dans un repère orthonormal alors, AB=√x2+y2.
Applications :
⋅ Nature d'un triangle
On considère un repère orthonormal (O; →i, →j) et on donne A(1−2), B(21) et C(50).
Déterminons la nature exacte du triangle ABC
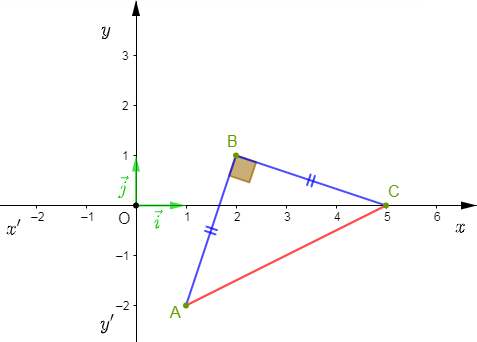
On a : →AB(13) alors, AB=√12+32=√10
→AC(42) alors, AC=√42+22=2√5
et →BC(3−1) alors, BC=√32+(−1)2=√10
Donc, AB=BC. Ainsi, ABC est un triangle isocèle en B.
De plus on a AC2=(2√5)2=20, AB2=(√10)2=10 et BC2=(√10)2=10.
Par suite, AC2=AB2+BC2. D'après la réciproque du théorème de Pythagore ABC est un triangle rectangle en B.
D'où, ABC est rectangle et isocèle en B.
⋅ Condition d'orthogonalité de deux vecteurs dans un repère orthonormal
Soient →u(xy) et →v(x′y′) deux vecteurs dans un repère orthonormal (O; →i, →j) tels que →u⊥→v
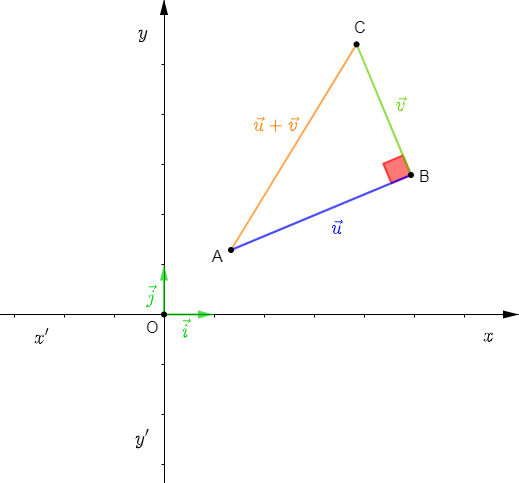
On a : ABC triangle rectangle en B. D'après le théorème de Pythagore on aura : AC2=AB2+BC2.
Or, →AC=→u+→v(x+x′y+y′) alors, AC2=(x+x′)2+(y+y′)2
→AB=→u(xy) alors, AB2=x2+y2
et →BC=→v(x′y′) alors, BC2=x′2+y′2
Donc, x2+2xx′+x′2+y2+2yy′+y′2=x2+y2+x′2+y′2
Ainsi, 2xx′+2yy′=0
D'où, xx′+yy′=0
Si →u(xy) et →v(x′y′) sont deux vecteurs dans un repère orthonormal et →u⊥→v alors, x.x′+y.y′=0
Réciproque :
Si →u(xy) et →v(x′y′) sont deux vecteurs dans un repère orthonormal tels que x.x′+y.y′=0 alors, →u⊥→v
Exemple : on considère un repère orthonormal (O; →i, →j) et on donne A(12), B(21) et C(50).
Démontrons que (AB)⊥(BC).
On a : (AB)⊥(BC) si, et seulement si, →AB⊥→BC.
Or, →AB(13) et →BC(3−1) alors, (1)(3)+(3)(−1)=3−3=0.
Donc, →AB⊥→BC.
D'où, (AB)⊥(BC).
V. Équation générale d'une droite
V.1 Droite passant par deux points dans un repère orthonormal
Soient A(−12) et B(21) deux points dans un repère orthonormal (O; →i, →j).
Déterminer l'équation de la droite (AB).
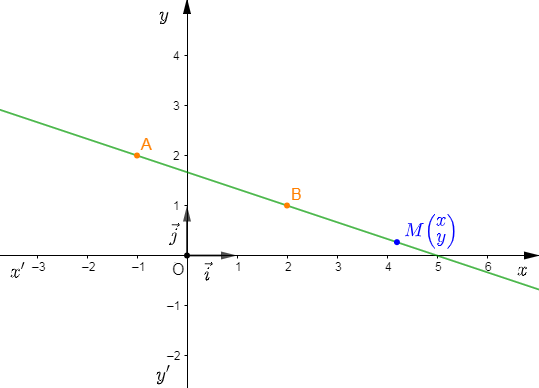
Soit M(xy)∈(AB) alors, →AM et →AB sont colinéaires.
Or, →AM(x+1y−2) et →AB(3−1)
Donc, −1(x+1)−3(y−2)=0
Ainsi, −x−1−3y+5=0
D'où, (AB): −x−3y+5=0 ou encore (AB): x+3y−5=0
V.2 Droite définie par un vecteur et un point : vecteur directeur
Soient →u(23) un vecteur et A(3−2) un point dans un repère orthonormal (O; →i, →j).
Déterminons l'équation de la droite (Δ) passant par A et de direction →u.
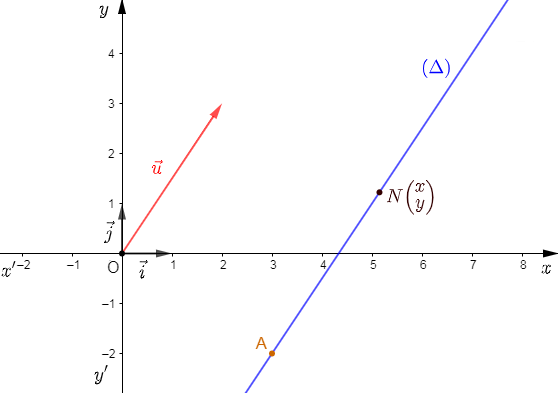
Soit N(xy)∈(Δ) alors, →AN et →u sont colinéaires.
Or, →AN(x−3y+2) et →u(23)
Donc, 3(x−3)−2(y+2)=0
Ainsi, 3x−9−2y−4=0
D'où, (Δ): 3x−2y−13=0
Le vecteur →u de coordonnées (23) est appelé un vecteur directeur de la droite (Δ) d'équation 3x−2y−13=0.
Et l'équation 3x−2y−13=0 est une équation générale de (Δ).
Théorème :
Si (Δ): ax+by+c=0 alors, →u(−ba) est un vecteur directeur de (Δ)
Exemple : trouvons un vecteur directeur de chacune des deux droites suivantes : (D): 4x−3y+5=0 et (Δ): 7x+5y−13=0.
On a : →u(34) est un vecteur directeur de (D) et →v(−57) celui de (Δ)
Applications :
⋅ Droite passant par un point et parallèle à une droite donnée
Soient (D): 4x−3y+1=0 et A(12) un point dans un repère orthonormal (O; →i, →j).
Déterminons l'équation de la droite (Δ) passant par le point A et parallèle à la droite (D).
Soit M(xy)∈(Δ)
On a :(D): 4x−3y+1=0 et alors, →u(34) un vecteur directeur.
Donc, →AM et →u sont colinéaires.
Or, →AM(x−1y−2) et →u(34)
Par suite, 4(x−1)−3(y−2)=0
Ainsi, 4x−4−3y+6=0
D'où, (Δ): 4x−3y+2=0
⋅ Droite passant par un point et perpendiculaire à une droite donnée
Soit (D): 3x+2y−5=0 une droite données et A(−2−3) un point dans un repère orthonormal (O; →i, →j).
Déterminons l'équation de la droite (Δ) passant par le point A et perpendiculaire à la droite (D).
Soit M(xy)∈(Δ)
On a : (D): 3x+2y−5=0 et alors, →u(−23) un vecteur directeur.
Et comme (Δ)⊥D donc, →AM⊥→u.
Or, →AM(x+2y+3) et →u(−23)
Par suite, −2(x+2)+3(y+3)=0
Ainsi, −2x−4+3y+9=0
D'où, (Δ): −2x+3y+5=0
⋅ Équation d'une droite passant par un point et parallèle à l'axe des abscisses (xx′): y=0
Soit A(ab) un point dans un repère orthonormal (O; →i, →j).
Déterminons l'équation de la droite (Δ) passant par le point A et parallèle à l'axe des abscisses.

Soit M(xy)∈(Δ)
On a : →i(10) un vecteur directeur de (xx′) et comme (Δ)∥(xx′) alors, →AM et →i sont colinéaires.
Or, →AM(x−ay−b) et →i(10) donc, 0(x−a)−1(y−b)=0.
Par suite, −y+b=0.
D'où, (Δ): y=b.
Si A(ab)∈(Δ) un point dans un repère orthonormal et (Δ)∥(xx′) alors, (Δ): y=b
⋅ Équation d'une droite passant par un point et parallèle à l'axe des ordonnées (yy′): x=0
Soit A(ab) un point dans un repère orthonormal (O; →i, →j).
Déterminons l'équation de la droite (Δ) passant par le point A et parallèle à l'axe des ordonnés.
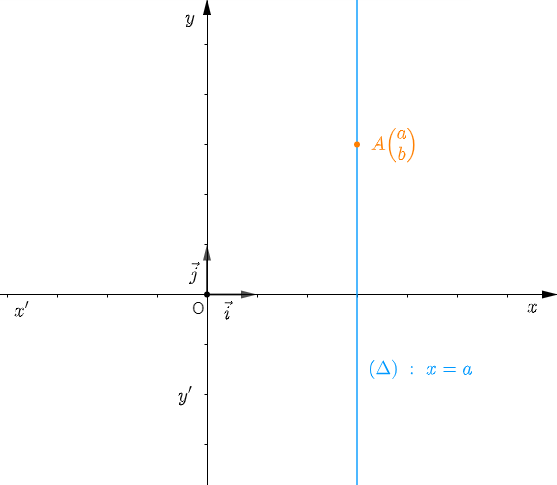
Soit M(xy)∈(Δ)
On a : →j(01) un vecteur directeur de (yy′) et comme (Δ)∥(yy′) alors, →AM et →j sont colinéaires.
Or, →AM(x−ay−b) et →j(01) donc, 1(x−a)−0(y−b)=0.
Ainsi, x−a=0.
D'où, (Δ): x=a.
Si A(ab)∈(Δ) un point dans un repère orthonormal et (Δ)∥(yy′) alors, (Δ): x=a.
VI. Équation réduite d'une droite
VI.1 Exemples et Définitions
Soit (Δ): 3x−2y+6=0
On peut écrire alors (Δ): y=32x+3.
L'équation écrite sous la forme y=32x+3 est appelée équation réduite de la droite (Δ) de coefficient directeur 32 et d'ordonnée à l'origine +3.
Ce qui signifie que la droite (Δ) passe par le point de coordonnées (0; 3).
De manière générale, l'équation réduite d'une droite (Δ) s'écrit sous la forme y=ax+b où a∈R et b∈R.
Le réel a est appelé le coefficient directeur (pente) de la droite (Δ) et le réel b son ordonnée à l'origine ; c'est à dire la droite passe par un point de coordonnées (0; b).
VI.2 Relation entre coefficient directeur et les coordonnées du vecteur directeur d'une droite
Soit (Δ): 3x−2y+6=0
On a : →u(23) un vecteur directeur de (Δ) et (Δ): y=32x+3.
Ainsi, le coefficient directeur d'une droite est égal au rapport de l'ordonnée du vecteur directeur par son abscisse.
Si →u(mn) un vecteur directeur de (Δ): y=ax+b avec m≠0 alors, a=nm
Exemple 1 : trouvons le coefficient directeur d'une droite (Δ) de vecteur directeur →u(−36)
Soit (Δ): y=ax+b et →u(−36) un vecteur directeur de (Δ) alors, a=6−3=−2
Exemple 2 : trouvons un vecteur directeur de la droite (Δ) d'équation y=45x−6
On a : →u(54) un vecteur directeur de (Δ).
VI.3 Propriétés
⋅ Condition de parallélisme de deux droites
Soient (D): y=ax+b et (Δ): y=a′x+b′ deux droites dans un repère orthonormal telles que (D)∥(Δ).
On a : →u(1a) un vecteur directeur de (D), →v(1a′) un vecteur directeur de (Δ) et (D)∥(Δ)
alors, →u et →v sont colinéaires.
Donc, 1(a′)−1(a)=0
Ainsi, a′−a=0. D'où a′=a
Si (D): y=ax+b et (Δ): y=a′x+b′ deux droites dans un repère orthonormal et (D)∥(Δ) alors, a=a′
Réciproque :
Si (D): y=ax+b et (Δ): y=a′x+b′ deux droites dans un repère orthonormal et a=a′ alors, (D)∥(Δ)
⋅ Condition d'orthogonalité de deux vecteurs
Soient (D): y=ax+b et (Δ): y=a′x+b′ deux droites dans un repère orthonormal telles que (D)⊥(Δ).
On a : →u(1a) un vecteur directeur de (D) et →v(1a′) un vecteur directeur de (Δ).
Et comme (D)⊥(Δ) alors, →u⊥→v.
Donc, (1)(1)+(a)(a′)=0
Ainsi, 1+a.a′=0. D'où, aa′=−1
Si (D): y=ax+b et (Δ): y=a′x+b′ deux droites dans un repère orthonormal et (D)⊥(Δ) alors, aa′=−1
Réciproque :
Si (D): y=ax+b et (Δ): y=a′x+b′ deux droites dans un repère orthonormal et aa′=−1 alors, (D)⊥(Δ)
Applications :
⋅ Droite passant par deux points dans un repère orthonormal
Soit A(−12) et B(21) deux points dans un repère orthonormal (O; →i, →j).
Déterminons l'équation de la droite (AB)
Soit (AB): y=ax+b. On a →AB(3−1) un vecteur directeur de (AB) alors, a=−13
Donc, (AB): y=−13x+b et A(−12)∈(AB)
Par suite, 2=−13(−1)+b
Ainsi, b=2−13=53
D'où, (AB): y=−13x+53
Auteur:
Abdoulaye Ba

Commentaires
Mory (non vérifié)
mer, 11/27/2019 - 19:26
Permalien
Contribution
Anonyme (non vérifié)
dim, 08/16/2020 - 18:33
Permalien
J'aurais voulu savoir comment
Niang (non vérifié)
dim, 08/16/2020 - 18:34
Permalien
J'aurais voulu savoir comment
yahya (non vérifié)
mer, 04/22/2020 - 23:24
Permalien
pc
Anonyme (non vérifié)
sam, 08/07/2021 - 18:23
Permalien
Vrmt ça m'a beaucoup aide
Ibrahima Diouf (non vérifié)
mar, 11/02/2021 - 23:24
Permalien
Bien
Anonyme (non vérifié)
mar, 05/07/2024 - 20:29
Permalien
c'est cool
Anonyme (non vérifié)
mer, 09/11/2024 - 13:54
Permalien
merci
Ajouter un commentaire