Fonctions scalaires et vectorielles de Leibniz - T S1
I Barycentre
Soient (Ai, αi)1≤i≤n, n points pondérés ; αi∈R, Ai∈ l'espace E. On appelle barycentre de (Ai, αi) l'unique point G vérifiant :
α1→GA1+α2→GA2+…+αn→GAn=→0 avec ∑ni=1αi≠0
I.1 Propriétés
⋅ Propriété caractéristique : G barycentre de (Ai, αi)1≤i≤n, si et seulement si, ∀M∈E, n∑i=1αi→MAi=(n∑i=1αi)→MG
et ∑ni=1αi≠0.
Donc dans le repère orthonormé (O, →i, →j) on a : n∑i=1αi→OAi=(n∑i=1αi)→OG⇒ →OG=1∑ni=1αi(α1→OA1+α2→OA2+…+αn→OAn)
⇒G(1∑ni=1αi(α1x1+α2x2+…+αnxn)1∑ni=1αi(α1y1+α2y2+…+αnyn))
Aussi nous obtenons dans le repère (O; →i, →j, →k) :
zG=α1zA1+α2zA2+…+αnzAnα1+α2+…+αn.
zG=α1zA1+α2zA2+…+αnzAnα1+α2+…+αn.
⋅ G barycentre de (Ai, αi)1≤i≤n ⇔ ∀k∈R∗, G barycentre de (Ai, kαi)1≤i≤n. Le barycentre reste inchangé si on multiplie les coefficients par un même réel k≠0.
⋅ Barycentre partiel :
Soit G barycentre de (Ai, αi)i∈I,I⊂N.
Si G1 est barycentre de (Ai, αi)i∈I′,I′⊂I alors G sera barycentre de
{(G1, (∑αi)i∈I′);(Ai, αi)i∈(I∖I′)}
⋅ Remarques :
Soit G barycentre de (Ai, αi)i∈I,I⊂N.
Si ∀i, jαi=αj, on dira alors que G est isobarycentre de Ai, i∈I.
⋅ L'isobarycentre de deux points est leur milieu.
⋅ L'isobarycentre de trois points non alignés est le centre de gravité du triangle.
I.2 Produit scalaire
Considérons les vecteurs →AB et →AC. Soient les vecteurs →u et →v tels que :
→AB=→u et →AC=→v
On a :
→u⋅→v=||→u||×||→v||×cos(→u, →v)=¯ABׯAH=xx′+yy′
où H est le projeté orthogonal de C sur (AB) et →u et →v de coordonnées respectives (x, y) et (x′, y′) dans le repère orthonormé.
I.2.1 Propriétés
⋅ →u⋅→v=→v⋅→u
⋅ →u⋅(α→v)=(α→u⋅→v)=α(→u⋅→v)
⋅ →u⋅(→v+→w)=→v⋅→u=→u⋅→v+→u⋅→w
⋅ →u≠→0,→v≠→0,→u⋅→v=0 ⇔ →u⊥→v
I.2.2 Relation d'Alkashi

Soit ABC un triangle d'angles ˆA, ˆB et ˆC respectivement opposés aux cotés a, b et c. Nous avons :
a2=b2+c2−2bc.cosˆA
b2=a2+c2−2ac.cosˆB
c2=a2+b2−2ab.cosˆC
sinˆAa=sinˆBb=sinˆCc=2Sabc où S est la surface du triangle (ABC)
I.2.3 Théorème de la médiane
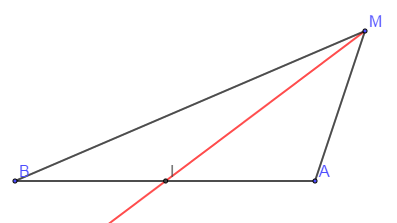
Soient I milieu du segment [AB] et M un point du plan tel que MBA soit un triangle. Alors on a :
→MI=12(→MA+→MB)MA2+MB2=2MI2+AB22
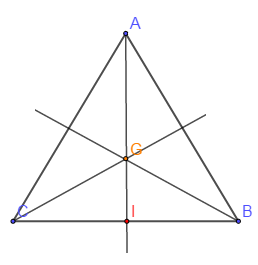
Soit ABC un triangle, G son centre de gravité et I milieu du segment [BC];
→AG=23→AI
→IG=13→IA
II Fonctions vectorielles de leibniz
II.1 Définitions
Soient (Ai)1≤i≤n des points de E, (αi)1≤i≤n∈R. On appelle fonction vectorielle de Leibniz l'application →f définie dans →E (l'ensemble des vecteurs) par :
∀M∈E→f(M)=n∑i=1αi→MAi=α1→MA1+α2→MA2+…+αn→MAn
⋅ Remarque : si G est barycentre de (Ai, αi)1≤i≤n alors →f(G)=→0
II.2 Expression réduite de →f(M)
1er cas :
si ∑αi=0
→f(M)=α1→MA1+α2→MA2+…+αn→MAn=α1(→MO+→OA1)+α2(→MO+→OA2)+…+αn(→MO+→OAn)=α1→MO+α1→OA1+α2→MO+α2→OA2+…+αn→MO+αn→OAn=(α1+α2+…+αn)→MO+α1→OA1+α2→OA2+…+αn→OAn=α1→OA1+α2→OA2+…+αn→OAn=→f(O)
Donc, →f est constante.
2ème cas :
si ∑αi≠0, alors (Ai, αi)1≤i≤n admettent un barycentre; soit G ce barycentre.On a
→f(M)=n∑i=1αi→MAi=(n∑i=1αi)→MG
III Fonctions scalaires de Leibniz
III.1 Définitions
Soient (Ai)1≤i≤n n points, (αi)1≤i≤n∈R. On appelle fonction scalaire de Leibniz l'application définie de E vers R par :
f(M)=n∑i=1αiMA2i
III.2 Expression réduite de f(M)
1er cas :
si ∑αi=0,
f(M)=α1→MA12+α2→MA22+…+αn→MAn2=α1(→MO+→OA1)2+α2(→MO+→OA2)2+…+αn(→MO+→OAn)2=(α1+α2+…+αn)→MO2+α1→OA12+α2→OA22+…+αn→OAn2+2→MO⋅(α1→OA1+α2→OA2+…+αn→OAn)=f(O)+2→MO⋅(α1→OA1+α2→OA2+…+αn→OAn)
Donc, f(M)=f(O)+2→MO⋅(α1→OA1+α2→OA2+…+αn→OAn)
2ème cas :
si ∑αi≠0, alors (Ai, αi)1≤i≤n admettent un barycentre; soit G ce barycentre.On a :
f(M)=α1(→MG+→GA1)2+α2(→MG+→GA2)2+…+αn(→MG+→GAn)2=(∑ni=1αi)→MG2+f(G)+2→MG⋅(α1→GA1+α2→GA2+…+αn→GAn)
Ainsi f(M)=(n∑i=1αi)→MG2+f(G)
Exercice d'application
Soit ABC un triangle tel que AB=AC=5 et BC=6.
1) Calculer →AB⋅→AC
2) Soit G barycentre de (A, 2), (B, 3) et (C, 3)
a) Calculer AG
b) Soit f(M) une fonction scalaire de Leibniz définie par f(M)=2→MB⋅→MC+→MA⋅(→MC+→MB)
Montrer que f(M)=4MG2+f(G)
c) Calculer f(A) et f(G)
d) Déterminer l'ensemble des points M tels que f(M)=f(A).
Résolution
1) →AB⋅→AC=AB×AC×cosˆA=bccosˆA, or d'après Alkashi on a :
a2=b2+c2−2bc.cosˆA ⇒ cosˆA=b2+c2−a22bc et donc
→AB⋅→AC=bcb2+c2−a22bc=b2+c2−a22=25+25−362=7
2) a) G barycentre de (A, 2), (B, 3) et (C, 3), alors
→AG=3→AB+3→AC8⇒ AG2=964(AB2+AC2+2→AB⋅→AC)=964(25+25+2×7)=9
Donc, AG=3
b)
f(M)=2→MB⋅→MC+→MA⋅(→MC+→MB)=2(→MG+→GB)⋅(→MG+→GC)+(→MG+→GA)⋅((→MG+→GC)+(→MG+→GB))=4MG2+→MG⋅(2→MA+3→MB+3→MC)⏟→0+2→GB⋅→GC+→GA⋅(→GC+→GB)=4MG2+2→GB⋅→GC+→GA⋅(→GC+→GB)=4MG2+f(G)
c) f(A)=2→AB⋅→AC=14
On a : f(M)=4MG2+f(G), alors f(G)=f(M)−4MG2 et donc pour M=A on trouve
f(G)=f(A)−4AG2=−22
d)
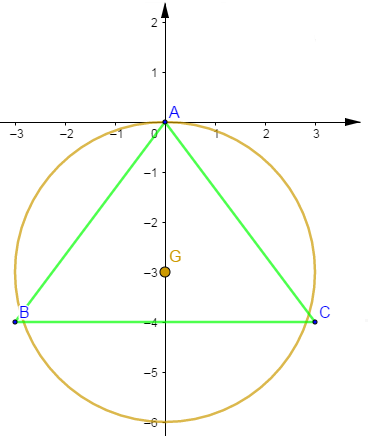
f(M)=f(A)⇔4MG2+f(G)=f(A)⇔4MG2=f(A)−f(G)⇔MG2=f(A)−f(G)4⇔MG=√14+224 = 3
Donc, l'ensemble des points M tels que f(M)=f(A) est le cercle de centre G et de rayon 3.
IV Surfaces et lignes de niveau
- E={M/ →u⋅→MA=k} , →u est un vecteur donné, A un point de E et k∈R.
⋅ Dans le plan E est une droite perpendiculaire à la direction de →u.
⋅ Dans l'espace E est un plan perpendiculaire à la direction de →u
- E={M/ MA=MB}
⋅ Dans le plan E est la médiatrice de [AB].
⋅ Dans l'espace E est le plan médiateur du segment [AB]
- E={M/ AM=k>0}
⋅ Dans le plan E=C(A, k), le cercle de centre A et de rayon k.
⋅ Dans l'espace E=S(A, k), la sphère de centre A et de rayon k
- E={M/ →MA⋅→MB=0}
⋅ Dans le plan E est le cercle de diamètre [AB]
⋅ Dans l'espace E est la sphère de diamètre [AB]
Auteur:
Seyni Ndiaye & Diny Faye

Commentaires
agnideachabi (non vérifié)
ven, 08/03/2018 - 17:30
Permalien
Calcul vectoriel
vianney (non vérifié)
dim, 11/14/2021 - 13:53
Permalien
La notion est bien détaillé j
Anonyme (non vérifié)
sam, 09/24/2022 - 23:26
Permalien
Z
Ajouter un commentaire