Transformations - Isométries du plan - T S1
I Définitions
On appelle transformation du plan toute application bijective du plan dans lui-même.
f est une transformation du plan P si ∀M∈P, ∃ un unique point N tel que f(N)=N.
Exemple :
translation , homothéties, symétries, rotation, affinités
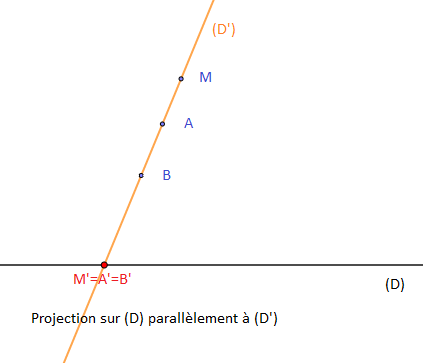
La projection n'est pas une transformation
II Exemples
II.1 Translation
II.1.1 Définitions
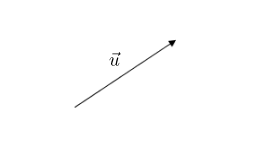
Soit →u un vecteur non nul.
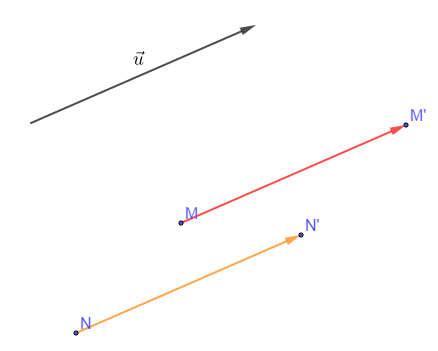
On appelle translation de vecteur →u la transformation du plan notée t→u qui associe, à tout point M de P, le point M′ tel que →MM′=→u.
II.1.2 Ensembles invariants
⋅ Un point M est invariant par une transformation f si f(M)=M. L'ensemble des points invariants est noté InVf.
⋅ Un ensemble E est dit globalement invariant par une transformation f si f(E)=E.
⋅ InVt→u=∅
⋅ Toute droite de direction →u est globalement invariant par t→u
II.1.3 Propriétés
⋅ Propriété caractéristique : soient M et N deux points du plan d'images respectives M′ et N′ par t→u.
Nous avons t→u(M)=M′ si, et seulement si, →MM′=→u
De même t→u(N)=N′ si, et seulement si, →NN′=→u
Ce qui entraine : →MM′=→NN′
D'où, (MM′N′N) est un parallélogramme
⋅ Une transformation f conserve le barycentre si pour G barycentre de (Ai, αi)1≤i≤n alors f(G) est barycentre de (f(Ai), αi)1≤i≤n
Si G est barycentre de (A, α), (B, β), (C, γ) avec α+β+γ≠0 alors, α→GA+β→GB+γ→GC=→0
Ainsi, si t→u(G)=G′, t→u(A)=A′, t→u(B)=B′ et t→u(C)=C′ alors
→G′A′=→GA, →G′B′=→GB et →G′C′=→GC
Et par suite, α→G′A′+β→G′B′+γ→G′C′=→0 avec α+β+γ≠0
Donc, G′ est barycentre de (A′, α), (B′, β), (C′, γ)
La translation conserve le barycentre. Elle conserve aussi le produit scalaire.
⋅ t→u(M)=M′ et t→u(N)=N′ alors →M′N′=→MN
Ce qui donne, ||→M′N′||=||→MN||
Ainsi, la translation du vecteur →u conserve la distance.
Une transformation du plan qui conserve la distance est appelée une isométrie. Donc la translation est une isométrie.
⋅ Soient trois points A , B et C d'images respectives A′ , B′ et C′ par t→u ; →A′B′=→AB et →A′C′=→AC alors on a : (→AB, →AC)=(→A′B′, →A′C′)
Donc, la translation de vecteur →u conserve les angles orientés.
Une transformation du plan qui conserve les distances et la angles orientés est appelée un déplacement.
Donc une translation est un déplacement.
⋅ t→u(M)=M′ et t→u(N)=N′ ⟹→M′N′=→MN
Donc, →M′N′ et →MN sont colinéaires.
D'où, l'image d'une droite est une droite qui lui est parallèle.
En conclusion la translation conserve :
⋅ l'alignement
⋅ le parallélisme
⋅ l'orthogonalité
⋅ le barycentre
⋅ les distances
⋅ les angles orientés
Aussi, l'image d'une figure quelconque est une figure de même nature.
II.1.4 Expression complexe et analytique
Soient →u(ab) ; z→u=a+ib. M(xy) et M′(x′y′) ; t→u(M)=M′.
M d'affixe z noté M(z); z=x+iy et M′ d'affixe z′ noté M′(z′); z′=x′+iy′
On a : →MM′=→u⇔z→MM′=z→u⇔z−z′=z→u⇔z′=z+z→u
z′=z+α est l'expression complexe d'une translation de vecteur →u(α) avec α=z→u
Nous avons : z′=z+z→u ⇒ x′+iy′=x+iy+a+ib=x+a+i(y+b)
Ainsi, {x′=x+ay′=y+b est l'expression analytique d'une translation.
II.1.5 Composée et réciproque
Soit t une translation, M un point du plan d'image M′ par t.
On a : t→0=Id.
En effet, t→0(M)=M′ si et seulement si →MM′=→0. Donc M=M′
Soient deux vecteurs →u et →v non nuls alors t→u∘t→v=t→u+→v
Et donc, t→u∘t→v(M)=t→u[t→v(M)]=t→u(M1)=M′
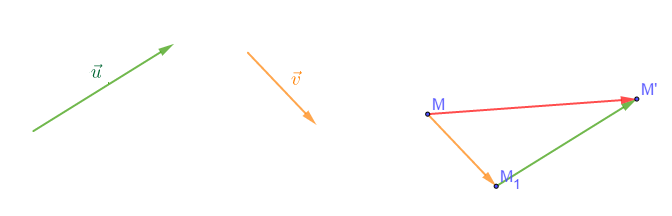
En effet, t→v(M)=M1, t→u(M1)=M′
→MM′=→MM1+→M1M′=→u+→v
Donc, t→u∘t→v=t→u+→v
Soient →u(α) et →v(β) deux vecteurs d'affixes respectifs α et β
On a : t→u : z′=z+α et t→v : z′=z+β
Alors, la composée t→u∘t→v sera définie par : z′=z+β+α avec β+α affixe de →u+→v
t→u une translation de vecteur →u alors on a :
t→u(M)=M′⇔→MM′=→u⇔→M′M=−→u⇔M=t−→u(M′)
Donc, (t→u)−1=t−→u
La réciproque de la translation de vecteur →u est la translation de vecteur −→u.
Exercice d'application
Soit f l'application qui, à tout M(xy), associe le point M′(x′y′) tels que {x′=x−2y′=y+1
1) Déterminer InVf
2) Montrer que f est une translation de vecteur →u dont on donnera les éléments caractéristiques.
3) Déterminer les images de A(12), C(B(03); 5) et D : y−2x+1=0
4) Donner l'expression complexe
Résolution
1) M∈InVf⇔f(M)=M⇔{x′=xy′=y⇔{x=x−2y=y+1impossible
Donc, InVf=∅
2) Soit M′(x′y′) image de M(xy) par f; →MM′(x′−xy′−y), alors f(M)=M′⇒{x′=x−2y′=y+1⇒{x′−x=−2y′−y=1⇒→MM′(x′−xy′−y)=→ω(−21)
Soit →u=→ω, alors f est une translation de vecteur →u=−2→i+→j
3) Soit A′=t→u(A), alors {xA′=xA−2yA′=yA+1⇒{xA′=1−2yA′=2+1⇒A′(−13)
On a : t→u(C)=C′(B′; 5).
Soit B′=t→u(B), alors {xB′=xB−2yB′=yB+1 ⇒ {xB′=−2yB′=4
Donc, C′(B′(−24); 5)
Soit D′=t→u(D) alors, D′//D et donc, D′ : y−2x+c=0
Déterminons la constante c
Soit E(0−1)∈D alors, t→u(E)=E′(x′y′)∈D′.
On a : {xE′=xE−2yE′=yE+1 ⇒ E′(−20)
E′∈D′, alors 4+c=0 ⇒ c=−4. Donc D′ : y−2x−4=0
4) Soit z=x+iy l'affixe de M et z′=x′+iy′ celui de M′, alors z′=x′+iy′=x−2+i(y+1)=x+iy−2+i=z−2+i
Donc, z′=z−2+i est l'expression complexe de cette transformation.
II.2 Homothéties
II.2.1 Définitions
Soit Ω un point du plan , k∈R∗∖{1}. On appelle homothétie de centre Ω et de rapport k la transformation du plan notée h(Ω, k) qui laisse Ω invariant et qui associe à tout point M différent de Ω le point M′ tel que →ΩM′=k→ΩM
h(Ω, k)(M)=M′⇔→ΩM′=k→ΩM
II.2.2 Ensembles invariants
⋅ InVh(Ω, k)={Ω}. Ω est ici le seul point invariant.
Soit M∈ InVh(Ω, k)
Nous avons : h(Ω, k)(M)=M si, et seulement si, →ΩM=k→ΩM
Ce qui donne : →ΩM(1−k)=→0 or 1−k≠0
Et donc, →ΩM=→0. D'où, Ω=M
⋅ Toute droite passant par Ω est globalement invariant par h(Ω, k) car Ω, M et M′ alignés.
II.2.3 Propriétés
⋅ h(Ω, k)(M)=M′⟹Ω,M,M′ alignés.
⋅ h(Ω, k)(M)=M′ alors →ΩM′=k→ΩM
h(Ω, k)(N)=N′ alors, →ΩN′=k→ΩN
Ce qui entraine →M′N′=k→MN
⋅ G barycentre de (Ai, αi)1≤i≤n, alors α1→GA1+α2→GA2+…+αn→GAn=→0.
h(Ω,k)(G)=G′ et h(Ω,k)(Ai)=A′i⇒→G′A′i=k→GAi⇒kα1→GA1+kα2→GA2+…+kαn→GAn=→0⇒α1→G′A′1+α2→G′A′2+…+αn→G′A′n=→0
Ce qui montre que G′ est aussi barycentre de (Ai, αi)1≤i≤n
Donc, il y a conservation du barycentre d'où, l'image d'une droite est une droite qui lui est parallèle car →M′N′ colinéaire à →MN
⋅ →M′N′=k→MN⇒M′N′=|k|.MN
Si |k|≠1 , la distance n'est pas conservée. L'homothétie de rapport |k|≠1 multiplie :
⋅ les distances par |k|
⋅ les aires par k2
⋅ les volumes par k3
⋅ soient h(Ω, k)(A)=A′, h(Ω, k)(B)=B′ et h(Ω, k)(C)=C′ alors on a :
→A′B′=k→AB et →A′C′=k→AC ⇒ (→A′B′, →A′C′)=(k→AB, k→AC)=(→AB, →AC)
Donc, les angles orientés sont conservés.
L'homothétie conserve :
⋅ le barycentre
⋅ les angles orientés
⋅ la perpendiculaire
⋅ le parallélisme
L'image d'une figure quelconque est une figure de même nature.
II.2.4 Expression analytique et complexe
Soient Ω(ab) d'affixe zΩ=a+ib=α , M(xy) d'affixe zM=z=x+iy et M′(x′y′) d'affixe zM′=z′=x′+iy′.
On a : h(Ω, k)(M)=M′⇔→ΩM′=k→ΩM⇔z′−zΩ=k(z−zΩ)⇔z′=kz+(1−k)zΩ
Ainsi, z′=kz+β est l'expression complexe d'une homothétie.
Autrement, z′−zΩ=k(z−zΩ)⇔{x′−a=k(x−a)y′−b=k(y−b)⇔{x′=kx+α1y′=ky+β1 Ce dernier système détermine l'expression analytique d'une homothétie.
Exercice d'application
Soit f l'application définie par {x′=x−2y′=y+1 qui, à tout M(xy), associe le point M′(x′y′)
1) Déterminer InVf
2) Montrer que f est une homothétie dont on donnera les éléments caractéristiques.
3) Déterminer l'expression complexe
4) Déterminer les images de O(00), C(I(13); 5) et D : 2x−y+3=0
Résolution
1) M∈InVf⇔f(M)=M⇔{x′=xy′=y⇔{x=2x−1y=2y+3⇔{x=1y=−3
Donc InVf=Ω(1−3)
2) Soit M′(x′y′) image de M(xy) par f, alors →ΩM′(x′−1y′+3)⇔(2x−1−12y+3+3)⇔(2x−22y+6)⇔(2(x−1)2(y+3))or →ΩM(x−1y+3)⇒ →ΩM′=2→ΩM
Soit →ΩM′=2→ΩM, donc f est une homothétie de centre Ω et de rapport 2. Ainsi, M′=h(Ω, 2)
3) Soit z=x+iy l'affixe de M et z′=x′+iy′ celui de M′, alors
z′=x′+iy′=2x−1+i(2y+3)=2x+2iy−1+3i=2(x+iy)−1+3i=2z−1+3i
z′=x′+iy′=2x−1+i(2y+3)=2x+2iy−1+3i=2(x+iy)−1+3i=2z−1+3i
Donc, z′=2z−1+3i est l'expression complexe de cette transformation.
4) Soit O′=h(Ω, 2)(O), alors zO′=2zO−1+3i=−1+3i
Donc, O′(−13)
On a : h(Ω, 2)(C)=C′(I′; 10).
Soit I′=h(Ω, 2)(I), alors zI′=2zI−1+3i=2(1+3i)−1+3i=2+6i−1+3i=1+9i
Donc, I′(19)
Soit D′=h(Ω, 2)(D), alors D′//D et donc D′ : 2x−y+c=0.
Déterminons la constante c
Soit B(03)∈D, alors B′=h(Ω, 2)(B) ⇒ zB′=2zB−1+3i=2(0+3i)−1+3i=6i−1+3i=−1+9i
Donc, B′(−19)∈D′, alors −2−9+c=0 ⇒ c=11. Donc D′ : 2x−y+11=0.
II.2.5 Composée et réciproque
⋅
h(Ω, k)(M)=M′⇒→ΩM′=k→ΩM⇒→ΩM=1k→ΩM′⇒M=h(Ω, 1k)(M′)
Donc, (h(Ω, k))−1=h(Ω, 1k)
La réciproque d'une homothétie de centre Ω et de rapport k est l'homothétie de centre Ω et de rapport 1k
⋅ Soient h1 et h2 deux homothéties de même centre Ω telles que h1=h(Ω, k) et h2=h(Ω, k′)
⋅ si kk′=1 alors h1∘h2=Id.
⋅ si kk′≠1 alors h1∘h2=h(Ω, kk′)
⋅ h1 et h2 sont de centres différents ; h1=h(Ω1, k1) et h2=h(Ω2, k2).
On a : h1 : z′=k1z+α , h2 : z′=k2z+β
Donc, h1∘h2 : z′=k2(k1z+α)+β=k2k1z+α+β
⇒h1∘h2:k2k1z+a avec a=α+β
⋅ si kk′=1 alors h1∘h2 est une translation.
⋅ si kk′≠1 alors h1∘h2 est une homothétie de rapport k1k2 et de centre le point invariant.
⋅ Remarques : h(Ω, −1)=SΩ, car →ΩM′=−→ΩM ⟹Ω est milieu de [AB]
II.3 Réflexion
II.3.1 Définition
Soit Δ une droite. On appelle réflexion d'axe Δ la transformation du plan qui laisse tout point de Δ invariant et qui associe à M∉Δ le point M′ tel que Δ soit la médiatrice de [AB].

II.3.2 Propriétés
⋅ InVSΔ=(Δ)
⋅ Toute perpendiculaire à Δ est globalement invariant par SΔ
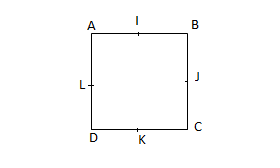
⋅ ABCD un carré ; SBD, SAC, SIK et SJL laissent invariant le carré.
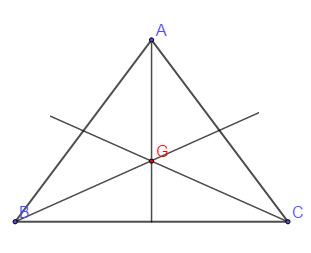
⋅ ABC un triangle équilatérale ; SAG, SBG et SCG laissent invariant le triangle ; G isobarycentre de ABC.
⋅ La symétrie axiale conserve :
⋅ les angles consécutifs mais change le signe des angles orientés.
⋅ les distances
⋅ le parallélisme
La symétrie axiale est un antidéplacement ou isométrie négative. L'image d'une figure quelconque est une figure de même nature et de même dimension.
II.3.3 Expression analytique et complexe
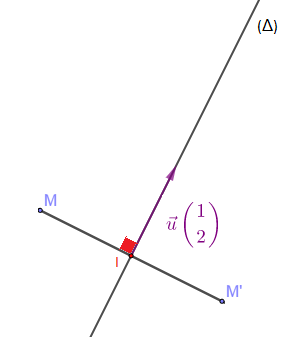
Soit (Δ) la droite d'équation : 2x−y+3=0. On se donne M(xy) un point du plan d'image M′(x′y′) par la réflexion d'axe (Δ). Soient →u(12) vecteur directeur de (Δ) et I(x+x′2y+y′2) milieu de [MM′], donc I∈Δ. On a :
SΔ(M)=M′⇔→MM′⋅→u=→0 et I∈Δ⇔{1(x′−x)+2(y′−y)=02(x+x′2)−(y+y′2)+3=0⇔{x′+2y′−x−2y=02x′−y′+2x−y+6=0⇔{x′=15(−3x+4y−12)y′=15(4x+3y+6)
Ce dernier système définit une expression analytique de la réflexion SΔ.
En écriture complexe on a :
M(xy) d'affixe zM=z=x+iy et M′(x′y′) d'affixe zM′=z′=x′+iy′.
Donc,
z′=15(−3x+4y−12)+i5(4x+2y+6)=(x−iy)(−35+45i)−125+65i⇒ z′=(−35+45i)¯z−125+65i
Cette dernière écriture traduit l'expression complexe de S(Δ) avec ¯z=x−iy.
II.3.4 Composée et réciproque
Soit SΔ une réflexion d'axe Δ. On a :
(SΔ)−1=SΔ
Supposons (Δ1)//(Δ2) et SΔ1, SΔ2 deux réflexions d'axes Δ1 et Δ2 respectivement. Alors, pour tout M, l'image M′, par leur composée est donnée par :
SΔ2∘SΔ1(M)=SΔ2(SΔ1(M))=SΔ2(M1)=M′
Nous avons :
→MM′=→MM1+→M1M′=2→IM1+2→M1J=2(→IM1+→M1J)=2→IJ⇒ M′=t2→IJ(M)
I et J sont respectivement les points d'intersection de la droite perpendiculaire à Δ1 et à Δ2 passant par M
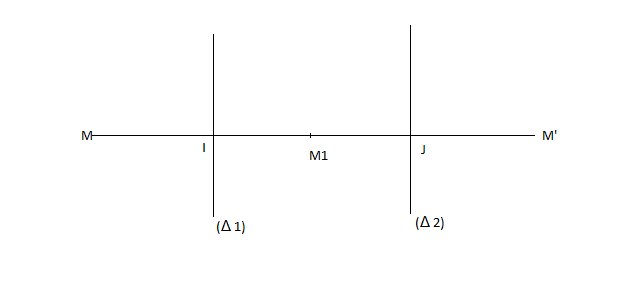
SΔ2∘SΔ1=t2→IJ
II.4 Rotation
II.4.1 Définition
Soient θ∈R et Ω un point du plan. On appelle rotation d'angle θ et de centre Ω la transformation du plan notée r(Ω, θ) qui laisse Ω invariant et qui associe à tout point M de P le point M′ tel que
{ΩM=ΩM′(→ΩM, →ΩM′)=θ (2π),θ≠0(2π)
{ΩM=ΩM′(→ΩM, →ΩM′)=θ (2π),θ≠0(2π)
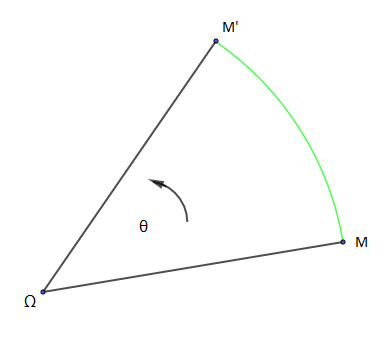
⋅ si θ=0(2π)⟹r(Ω, θ)=Id
⋅ si θ=π(2π)⟹r(Ω, θ)=SΩ
II.4.2 Points invariants
⋅ InVr(Ω, θ)={Ω}. Ω est le seul point invariant.
⋅ ABCD carré direct alors r(O, π2) , r(O, π) et r(O, −π2) laissent globalement invariant le carré.

⋅ ABC triangle alors r(G, 2π3) et r(G, −2π3) laissent globalement invariant le triangle ABC.
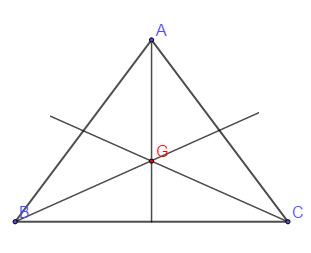
II.4.3 Propriétés
⋅ r(Ω, θ) conserve le barycentre, les distances et les angles orientés. Donc, une rotation est un déplacement.
⋅ r(Ω, θ) conserve le parallélisme, la perpendiculaire et l'alignement.

On a :
M′N′2=ΩM′2+ΩN′2−2ΩN′.ΩM′.cosα=ΩM2+ΩN2−2ΩN.ΩM.cosαDonc M′N′2=MN2⇒ M′N′=MN
M′N′2=ΩM′2+ΩN′2−2ΩN′.ΩM′.cosα=ΩM2+ΩN2−2ΩN.ΩM.cosαDonc M′N′2=MN2⇒ M′N′=MN
II.4.4 Expression complexe et analytique
Soient Ω(ab) d'affixe zΩ=a+ib=α , M(xy) d'affixe zM=z=x+iy et M′(x′y′) d'affixe zM′=z′=x′+iy′.
On a :
r(Ω, θ)(M)=M′⇔{ΩM=ΩM′(→ΩM, →ΩM′)=θ(2π)⇔{ΩM′ΩM=1(→ΩM, →ΩM′)=θ(2π)⇔{|z′−zΩz−zΩ|=1arg(z′−zΩz−zΩ)=θ(2π)
Donc z′−zΩz−zΩ=eiθz′−zΩ=eiθ(z−zΩ) ou z′=eiθz+β
Ainsi, z′=eiθz+β est l'expression complexe de la rotation d'angle θ et de centre Ω tels que zΩ=eiθzΩ+β , zΩ=β1−eiθ
Autrement, soit z′=eiθz+β avec β=β1+β2
On a :
x′+iy′=(cosθ+isinθ)(x+iy)+β1+β2⇒{x′=xcosθ−ysinθ+β1y′=ycosθ+xsinθ+β2
qui est l'expression analytique
II.4.5 Composée et réciproque
On a :
r(Ω, θ)(M)=M′⇔{ΩM=ΩM′(→ΩM, →ΩM′)=θ(2π)⇔{ΩM′=ΩM(→ΩM′, →ΩM)=−θ(2π)⇔M=r(Ω, −θ)(M′)=(r(Ω, θ))−1(M′)
D'où, (r(Ω, θ))−1=r(Ω, −θ). La réciproque de la rotation de centre Ω et d'angle θ est la rotation de même centre Ω et d'angle −θ.
− même centre :
Soient r1 et r2 deux rotations de même centre Ω invariant.
On a :
r1=r(Ω, θ1) : z′=eiθ1z+α
r2=r(Ω, θ2) : z′=eiθ2z+β
Soit r=r1∘r2 la composée de r1 et de r2. On a :
r=r1∘r2 : z′=eiθ1(eiθ2z+β)+α ⇒ z′=ei(θ1+θ2)z+eiθ2β+α
− si θ1+θ2≠0(2π), alors r1∘r2 est une rotation de centre Ω et d'angle θ=(θ1+θ2).
− si θ1+θ2=0(2π), alors θ1=−θ2 et donc r2=r(Ω, θ1)=(r(Ω, θ2))−1.
D'où, r1∘r2=Id
− centres différents :
r1 et r2 sont maintenant de centres différents. On a:
r1=r(Ω1, θ1) : z′−zΩ1=eiθ1(z−zΩ1) ou z′=eiθ1z+α
r2=r(Ω2, θ2) : z′−zΩ2=eiθ2(z−zΩ2) ou z′=eiθ2z+β
Soit r=r1∘r2 la composée de r1 et de r2. On a :
r=r1∘r2 : z′=eiθ1(eiθ2z+β)+α
Donc, M′ est d'affixe z′=ei(θ1+θ2z+δ
− si θ1+θ2≠0(2π) ⇒ r1∘r2 est une rotation de centre Ω tel que zΩ=δ1−ei(θ1+θ2) et d'angle θ=(θ1+θ2).
− si θ1+θ2=0(2π) ⇒ r1∘r2 est une translation de vecteur →u(δ)
− composée d'une rotation r(Ω, θ) et d'une translation de vecteur →u(δ) :
Soient r1 une rotation et t une translation de vecteur, définies respectivement par : r1=r(Ω, θ) ; z′=eiθz+β et t→u ; z′=z+α
Considérons la composée r=r1∘t définie par :
r=r(Ω, θ)∘t→u; z′=eiθ(z+α)+β ou encore z′=eiθz+δ
z′=eiθz+δ est l'expression complexe d'une rotation. Donc la composée d'une rotation et d'une translation est une rotation d'angle ; l'angle de la rotation et de centre ; le point invariant.
II.4.6 Décomposition d'une rotation
⋅ composée de deux symétries axiales d'axes sécants :
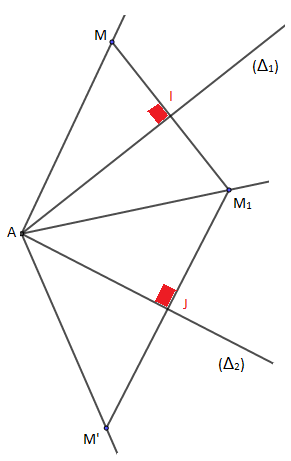
Soit A le point d'intersection entre Δ1 et Δ2 ; on a :
SΔ2∘SΔ1(M)=SΔ2(SΔ1(M))=SΔ2(M1)=M′
De plus AM=AM1=AM′
et
(→AM, →AM′)=(→AM, →AM1)+(→AM1, →AM′)=2(→AI, →AM1)+2(→AM1, →AJ)⇒ (→AM, →AM′)=2(→AI, →AJ)
Donc SΔ2∘SΔ1(M)=M′ ⇔ {AM=AM′(→AM, →AM′)=2(→AI, →AJ)(2π)
Ce qui se traduit par : M′=r[A, 2(→AI, →AJ)](M)
D'où, la composée SΔ2∘SΔ1 de deux réflexions d'axes sécants en A est une rotation de centre A et d'angle 2(^Δ1, Δ2)(2π)
Toute rotation r de centre Ω et d'angle θ peut se décomposer en une composée de symétries axiales d'axes (Δ1) et (Δ2) sécants en Ω ; le centre de la rotation est tel que 2(^Δ1, Δ2)=θ; (r=SΔ2∘SΔ1).
III Isométrie
III.1 Définition
Une isométrie du plan est une transformation qui conserve les distances, ∀M et N tels que f(M)=M′ et f(N)=N′ alors M′N′=MN.
III.2 Classification des isométries
⋅ une isométrie f qui fixe trois points A , B et C non alignés est l'identité.
⋅ une isométrie f qui fixe deux points A et B, si elle est différente de l'identité, est une symétrie axiale d'axe (AB).
⋅ une isométrie f qui a un seul point invariant Ω est une rotation de centre Ω
Exercice d'application
Soit f l'application définie par {x′=12(x+y√3+1)y′=12(−x√3+y+√3) qui, à tout M(xy), associe le point M′(x′y′)
a) Montrer que f est une isométrie
b) Déterminer InVf, en déduire que f est une rotation dont on précisera le centre et l'angle.
Résolution
a) Soient M1(x1y1) et M2(x2y2) deux points du plan d'images respectives M′1(x′1y′1) et M′2(x′2y′2), alors
||→M′1M′2||2=(x′2−x′1)2+(y′2−y′1)2=14(x2+y2√3+1−x1−y1√3−1)2+14(−x2√3+y2+√3+x1√3−y1−√3)2=14((x2−x1)+√3(y2−y1))2+14(−√3(x2−x1)+(y2−y1))2=14((x2−x1)2+3(y2−y1)2+3(x2−x1)2+(y2−y1)2)=14(4(x2−x1)2+4(y2−y1)2)=(x2−x1)2+4(y2−y1)2=||→M1M2||2
Donc, ||→M′1M′2||2=||→M1M2||2 ⇒ M′1M′2=M1M2, ce qui montre bien que f est une isométrie.
b)
M∈InVf⇔f(M)=M⇔{x′=xy′=y⇔{x=12(x+y√3+1)y=12(−x√3+y+√3)⇔{12(−x+y√3+1)=012(−x√3−y+√3)=0⇔{−x+y√3+1=0−x√3−y+√3=0⇔{x=1y=0
Donc, InVf=Ω(10)
f est une isométrie qui a un seul point invariant Ω, donc, f est une rotation de centre Ω d'affixe 1.
Soit z=x+iy l'affixe de M et z′=x′+iy′ celui de M′, alors
z′=x′+iy′=12(x+y√3+1)+i2(−x√3+y+√3)=x(12−i√32)+y(√32+i2)+12+i√32=(x+iy)(12−i√32)+12+i√32=(12−i√32)z+12+i√32
Donc, z′=e−iπ3z+b avec b=12+i√32. Ainsi, f est une rotation de centre Ω et d'angle θ=−π3.
III.3 Propriétés
⋅ La réciproque d'une isométrie est une isométrie.
⋅ La composée d'une isométrie est une isométrie.
⋅ Une isométrie conserve le barycentre, le produit scalaire, l'alignement, le parallélisme, la perpendiculaire. L'image d'une figure quelconque est une figure de même nature et de même dimension.
⋅ Une isométrie qui conserve la mesure des angles orientés est appelée déplacement et celle qui change les signes des angles orientés est appelée antidéplacement.
III.4 Décomposition d'une isométrie
Soit f une isométrie telle que f(A)=A′. Posons t la translation de vecteur →A′A ; t=t→A′A et f1=t∘f est une isométrie.
Nous avons f1(A)=t∘f(A)=t[f(A)]=t(A′)=A. Donc f1 est une isométrie qui fixe A.
f1=t∘f⇒t−1∘f1=f où t−1=t→AA′.
Ainsi, toute isométrie f peut se décomposer en une composition d'une translation et d'une isométrie qui fixe un point.
Théorème :
Etant donnés quatre points A, B, C et D tels que AB=CD, il existe une isométrie et une seule qui transforme A en C et B en D.
Si →AB=→CD alors l'isométrie est la translation de vecteur →AC.
Si →AB≠→CD alors l'isométrie est une rotation dont le centre est le point de rencontre des médiatrices de [BC] et de [BA] et d'angle θ=(^→AB, →CD) car
{r(Ω, θ)(M)=M′r(Ω, θ)(N)=N′⇔{MN=M′N′(→MN, →M′N′)=θ(2π)
{r(Ω, θ)(M)=M′r(Ω, θ)(N)=N′⇔{MN=M′N′(→MN, →M′N′)=θ(2π)
Auteur:
Seyni Ndiaye & Diny Faye

Commentaires
Anonyme (non vérifié)
dim, 03/20/2022 - 15:45
Permalien
Bien fait.. mais est-ce que
Anonyme (non vérifié)
dim, 03/20/2022 - 15:46
Permalien
Bien fait
Kirikou (non vérifié)
dim, 07/21/2024 - 17:02
Permalien
suggestion
Ajouter un commentaire