Vous êtes ici

Limites et Continuité - 1er S

Classe:

Première

I Limites

I.1 Limites à l'infinie

I.1.1 Limites infinies à l'infinie

Considérons la fonction

$f$ définie par

$f(x)=x^{2}$ ; son ensemble de définition est

$D_{f}=\mathbb{R}.$

Examinons le tableau suivant :

$\begin{array}{|c|c|c|c|c|c|c|c|c|c|c|c|c|}\hline x&-10^{18}&-10^{9}&-10^{4}&-100&-10&-1&0&1&10&10^{2}&10^{4}&10^{6} \\ \hline f(x)&10^{36}&10^{18}&10^{8}&10^{4}&10^{2}&1&0&1&10^{2}&10^{4}&10^{8}&10^{12} \\ \hline\end{array}$

Nous constatons que lorsque

$x$ prend des valeurs de plus en plus grandes, les valeurs de

$f(x)$ deviennent de plus en plus grandes. On dira que

$f(x)$ tend vers

$+\infty$ quand

$x$ tend vers

$+\infty.$

De même lorsque

$x$ prend des valeurs de plus en plus grandes en valeurs absolues mais en étant négatives,

$f(x)$ prend des valeurs de plus en plus grandes. On dira que

$f(x)$ tend vers

$+\infty$ quand

$x$ tend vers

$-\infty.$

Définitions intuitives

Soit

$f$ une fonction définie sur un domaine

$D_{f}$ .

$\centerdot\ \$ Lorsque

$x$ tend vers

$+\infty$ , si les

$f(x)$ tendent vers

$+\infty$ alors on dira que la limite de

$f(x)$ quand

$x$ tend vers

$+\infty$ est égale à

$+\infty$ et on note :

$\lim_{x\rightarrow +\infty}f(x)=+\infty$

$\centerdot\ \$ Lorsque

$x$ tend vers

$-\infty$ , si les

$f(x)$ tendent vers

$+\infty$ alors on dira que la limite de

$f(x)$ quand

$x$ tend vers

$-\infty$ est égale à

$+\infty$ et on note :

$\lim_{x\rightarrow -\infty}f(x)=+\infty$

$\centerdot\ \$ Lorsque

$x$ tend vers

$+\infty$ , si les

$f(x)$ tendent vers

$-\infty$ alors on dira que la limite de

$f(x)$ quand

$x$ tend vers

$+\infty$ est égale à

$-\infty$ et on note :

$\lim_{x\rightarrow +\infty}f(x)=-\infty$

$\centerdot\ \$ Lorsque

$x$ tend vers

$-\infty$ , si les

$f(x)$ tendent vers

$-\infty$ alors on dira que la limite de

$f(x)$ quand

$x$ tend vers

$-\infty$ est égale à

$-\infty$ et on note :

$\lim_{x\rightarrow -\infty}f(x)=-\infty$

I.1.2 Limites finies à l'infinie

Considérons la fonction

$f$ définie sur

$D_{f}=\mathbb{R}^{*}$ par

$f(x)=\dfrac{1}{x^{3}}.$

Soit le tableau de valeurs suivant :

$\begin{array}{|c|c|c|c|c|c|c|c|}\hline x&-10^{4}&-10^{2}&-10&10&10^{2}&10^{4}&10^{6} \\ \hline f(x)&-10^{-12}&-0.000001&-0.001&0.001&0.000001&10^{-12}&10^{-18} \\ \hline\end{array}$ En examinant ce tableau, nous constatons que lorsque

$x$ prend des valeurs de plus en plus grandes, les valeurs de

$f(x)$ restent très proches de 0. On dira que

$f(x)$ tend vers 0 quand

$x$ tend vers

$+\infty.$

De même, lorsque

$x$ prend des valeurs de plus en plus grandes en valeurs absolues, tout en étant négatives, les valeurs de

$f(x)$ deviennent de plus en plus proches de la valeur 0. On dira que

$f(x)$ tend vers 0 quand

$x$ tend vers

$-\infty.$

Définitions intuitives

Soit

$f$ une fonction définie sur un domaine

$D_{f}$ et

$\ell\in\mathbb{R}.$

$\centerdot\ \$ Lorsque

$x$ tend vers

$+\infty$ , si les

$f(x)$ tendent vers un réel

$\ell$ alors on dira que la limite de

$f(x)$ quand

$x$ tend vers

$+\infty$ est égale à

$\ell$ et on note :

$\lim_{x\rightarrow +\infty}f(x)=\ell$

$\centerdot\ \$ Lorsque

$x$ tend vers

$-\infty$ , si les

$f(x)$ tendent vers un réel

$\ell$ alors on dira que la limite de

$f(x)$ quand

$x$ tend vers

$-\infty$ est égale à

$\ell$ et on note :

$\lim_{x\rightarrow -\infty}f(x)=\ell$

Remarque

La limite lorsqu'elle existe, elle est unique.

I.2 Limites en un point $x_{0}$

Activité

Soit

$f$ une fonction dont la courbe représentative

$C_{f}$ est illustrée sur la figure ci-dessous.

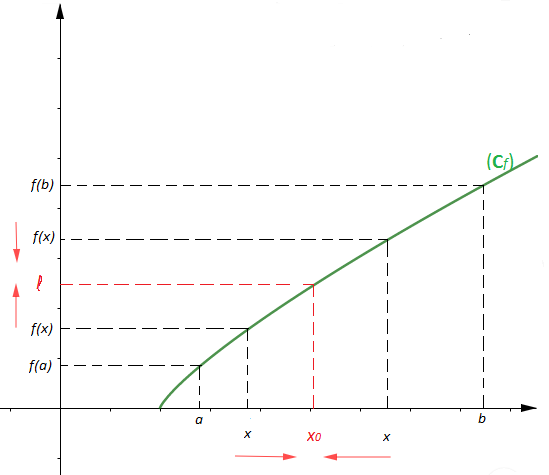
En examinant ce graphique nous pouvons constater que les valeurs de

$f(x)$ tendent vers

$L$ lorsque

$x$ tend vers

$x_{0}$ de part et d'autre.

Cet fait peut être exprimer en disant que

$f(x)$ a pour limite

$\ell$ lorsque

$x$ tend vers

$x_{0}$ et on note :

$\lim_{x\rightarrow x_{0}}f(x)=\ell$

I.2.1 Limite à gauche - limite à droite de $x_{0}$

Définitions

Soit

$f$ une fonction d'ensemble de définition

$D_{f}$ et

$x_{0}$ un réel.

$\centerdot\ \$ La limite éventuelle de la restriction de

$f$ à

$D_{f}\cap]-\infty\;;\ x_{0}[$ est appelée limite de

$f$ à gauche au point

$x_{0}$ ou encore la limite de

$f$ lorsque

$x$ tend vers

$x_{0}$ en étant inférieur à

$x_{0}$ , et on note :

$\lim_{\substack{x \to x_{0} \\ x<x_{0}}}f(x)\quad\text{ou plus simplement}\quad\lim_{x\rightarrow x_{0}^{-}}f(x)$

$\centerdot\ \$ La limite éventuelle de la restriction de

$f$ à

$D_{f}\cap]x_{0}\;;\ +\infty[$ est appelée limite de

$f$ à droite au point

$x_{0}$ ou encore la limite de

$f$ lorsque

$x$ tend vers

$x_{0}$ en étant supérieur à

$x_{0}$ , et on note :

$\lim_{\substack{x \to x_{0} \\ x>x_{0}}}f(x)\quad\text{ou plus simplement}\quad\lim_{x\rightarrow x_{0}^{+}}f(x)$

Théorème

On dit que

$f$ admet une limite fini

$\ell$ en

$x_{0}$ si et seulement si

$\lim_{x\rightarrow x_{0}^{-}}f(x)=\lim_{x\rightarrow x_{0}^{+}}f(x)=\ell$

I.2.2 Limites infinies en $x_{0}$

Soit la fonction

$f$ définie par

$f(x)=\dfrac{1}{x}\;;\quad D_{f}=\mathbb{R}^{*}.$

Examinons le tableau suivant :

$\begin{array}{|c|c|c|c|c|c|c|c|c|c|c|c|}\hline x&-0.1&-0.01&-10^{-4}&-10^{-8}&\cdots&0&\cdots&10^{-6}&10^{-3}&0.01&0.1 \\ \hline f(x)&-10&-100&-10^{4}&-10^{8}&\cdots&\times&\cdots&10^{6}&10^{3}&100&10 \\ \hline\end{array}$

Nous constatons que lorsque

$x$ prend des valeurs de plus en plus proches de 0 en étant supérieures à 0, les valeurs de

$f(x)$ deviennent de plus en plus grandes. On dira que

$f(x)$ tend vers

$+\infty$ quand

$x$ tend vers

$0^{+}.$ On note :

$\lim_{x\rightarrow 0^{+}}f(x)=+\infty$

De même lorsque

$x$ prend des valeurs de plus en plus proches de

$0$ en étant inférieures à

$0\;,\ f(x)$ prend des valeurs de plus en plus grandes en valeurs absolues mais en étant négatives. On dira que

$f(x)$ tend vers

$-\infty$ quand

$x$ tend vers

$0^{-}.$ On note :

$\lim\limits_{x\rightarrow 0^{-}}f(x)=-\infty$

Définitions intuitives

Soit

$f$ une fonction définie sur un domaine

$D_{f}$ et

$x_{0}$ appartenant à l'intérieur de ce domaine :

$\centerdot\ \$ Lorsque

$x$ tend vers

$x_{0}$ , si les

$f(x)$ tendent vers

$+\infty$ alors on dira que la limite de

$f(x)$ quand

$x$ tend vers

$x_{0}$ est égale à

$+\infty$ et on note :

$\lim_{x\rightarrow x_{0}}f(x)=+\infty$

$\centerdot\ \$ Lorsque

$x$ tend vers

$x_{0}$ , si les

$f(x)$ tendent vers

$-\infty$ alors on dira que la limite de

$f(x)$ quand

$x$ tend vers

$x_{0}$ est égale à

$-\infty$ et on note :

$\lim_{x\rightarrow x_{0}}f(x)=-\infty$

I.2.3 Limites finies en $x_{0}$

Soit

$f$ la fonction définie par

$f(x)=\dfrac{x^{2}-4}{x-2}$ . On a

$D_{f}=\mathbb{R}\setminus\{2\}.$

Considérons le tableau suivant :

$\begin{array}{|c|c|c|c|c|c|c|c|c|c|}\hline x&1.8&1.9&1.99&1.999&2.00001&2.0001&2.001&2.01&2.1 \\ \hline f(x)&3.8&3.9&3.99&3.999&4.00001&4.0001&4.001&4.01&4.1 \\ \hline\end{array}$

Nous constatons que lorsque

$x$ prend des valeurs de plus en plus proches de 2 en restant dans

$D_{f}$ , les valeurs de

$f(x)$ semblent s'approcher de la valeur 4. On exprime ce fait en disant que

$f(x)$ tend vers

$4$ quand

$x$ tend vers

$2.$ On note :

$\lim\limits_{x\rightarrow 2}f(x)=4$

Définition

Soit

$f$ une fonction définie sur un domaine

$D\;,\ x_{0}$ appartenant à l'intérieur de ce domaine et

$\ell$ un réel. Lorsque

$x$ prend des valeurs proches de

$x_{0}$ , si les valeurs de

$f(x)$ deviennent de plus en plus proches du réel

$\ell$ alors on dira que

$f(x)$ a pour limite

$\ell$ lorsque

$x$ tend vers

$x_{0}$ et on note :

$\lim_{x\rightarrow x_{0}}f(x)=\ell$

Travaux pratiques

Déterminer la limite des fonctions suivantes au point

$x_{0}=1$

$f(x)=\left\lbrace\begin{array}{rcl} 2x+1&\text{si}&x<1 \\ 3x^{2}-x-1&\text{si}&x>1\end{array}\right.$

$g(x)=\left\lbrace\begin{array}{rcl} x^{2}-1&\text{si}&x<1 \\ \\ \dfrac{3x}{x^{2}+1}&\text{si}&x>1\end{array}\right.$

$h(x)=\left\lbrace\begin{array}{rcl} \dfrac{2x}{x-1}&\text{si}&x>1 \\ \\ \dfrac{-x^{2}}{(x-1)^{2}}&\text{si}&x<1\end{array}\right.$

Résolution

$\lim\limits_{x\rightarrow 1^{-}}f(x)=\lim\limits_{x\rightarrow 1^{-}}2x+1=2\times 1+1=3$

$\lim\limits_{x\rightarrow 1^{+}}f(x)=\lim\limits_{x\rightarrow 1^{+}}3x^{2}-x-1=3\times(1)^{2}-1-1=1$

$\lim\limits_{x\rightarrow 1^{-}}g(x)=\lim\limits_{x\rightarrow 1^{-}}x^{2}-1=1-1=0$

$\lim\limits_{x\rightarrow 1^{+}}g(x)=\lim\limits_{x\rightarrow 1^{+}}\dfrac{3x}{x^{2}+1}=\dfrac{3\times 1}{1^{2}+1}=\dfrac{3}{2}$

$\lim\limits_{x\rightarrow 1^{+}}h(x)=\lim\limits_{x\rightarrow 1^{+}}\dfrac{2x}{x-1}$ or lorsque

$x$ tend vers

$1^{+}\;,\ (x-1)$ tend vers

$0^{+}.$

Donc,

$\lim\limits_{x\rightarrow 1^{+}}\dfrac{2x}{x-1}=\dfrac{2}{(x-1)\rightarrow 0^{+}}=+\infty.$

D'où,

$\lim\limits_{x\rightarrow 1^{+}}h(x)=+\infty$

$\lim\limits_{x\rightarrow 1^{-}}h(x)=\lim\limits_{x\rightarrow 1^{-}}\dfrac{-x^{2}}{(x-1)^{2}}$ or lorsque

$x$ tend vers

$1^{-}\;,\ (x-1)$ tend vers

$0^{-}$ et donc

$(x-1)^{2}$ va tendre vers

$0^{+}.$

Ainsi,

$\lim\limits_{x\rightarrow 1^{-}}\dfrac{-x^{2}}{(x-1)^{2}}=\dfrac{-1}{(x-1)^{2}\rightarrow 0^{+}}=-\infty.$

D'où,

$\lim\limits_{x\rightarrow 1^{-}}h(x)=-\infty$

I.3 Opération sur les limites

Soient

$f$ et

$g$ deux fonctions de limites finies ou non. Les limites de

$f+g\;,\ f\times g$ et de

$\dfrac{f}{g}$ sont récapitulées dans les tableaux ci-dessous.

N.B : Dans les cas où on ne peut conclure, on dira qu'on est en face d'une indétermination ou simplement une "forme indéterminée" notée

$(F.I)$

I.3.1 Limites d'une somme de deux fonctions

$\begin{array}{|c|c|c|c|c|c|c|}\hline \lim f&\ell&\ell&\ell&+\infty&-\infty&+\infty \\ \hline \lim g&\ell'&+\infty&-\infty&+\infty&-\infty&-\infty \\ \hline \lim (f+g)&\ell+\ell'&+\infty&-\infty&+\infty&-\infty&F.I \\ \hline\end{array}$

Exemple

$\lim\limits_{x\rightarrow +\infty}\left(x+1-\dfrac{1}{x^{3}}\right)=+\infty$ car en posant

$f(x)=x+1\$ et

$\ g(x)=-\dfrac{1}{x^{3}}$ on a

$\lim\limits_{x\rightarrow +\infty}f(x)=+\infty\$ et

$\ \lim\limits_{x\rightarrow +\infty}g(x)=0.$

Donc,

$\lim\limits_{x\rightarrow +\infty}(f(x)+g(x))=+\infty$

$\lim\limits_{x\rightarrow 0^{-}}\left(x+1-\dfrac{1}{x^{3}}\right)=+\infty$ car

$\ \lim\limits_{x\rightarrow 0^{-}}(x+1)=1\$ et

$\ \lim\limits_{x\rightarrow 0^{-}}-\dfrac{1}{x^{3}}=+\infty$

$\lim\limits_{x\rightarrow +\infty}(x^{3}-x^{2})\;,\ (F.I)$ car

$\ \lim\limits_{x\rightarrow +\infty}x^{3}=+\infty\$ et

$\ \lim\limits_{x\rightarrow +\infty}-x^{2}=-\infty$

I.3.2 Limites d'un produit

$\begin{array}{|c|c|c|c|c|c|c|c|c|c|}\hline \lim f&\ell&\ell>0&\ell<0&\ell>0&\ell<0&+\infty&-\infty&+\infty&0 \\ \hline \lim g&\ell'&+\infty&+\infty&-\infty&-\infty&+\infty&-\infty&-\infty&\infty \\ \hline \lim (f\times g)&\ell\times\ell'&+\infty&-\infty&-\infty&+\infty&+\infty&+\infty&-\infty&F.I \\ \hline\end{array}$

Exemple

$\lim\limits_{x\rightarrow +\infty}(x^{2}+2x-3)=\lim\limits_{x\rightarrow +\infty}x^{2}\left(1+\dfrac{2}{x}-\dfrac{3}{x^{2}}\right)=+\infty$

car

$\ \lim\limits_{x\rightarrow +\infty}x^{2}=+\infty\$ et

$\ \lim\limits_{x\rightarrow +\infty}\left(1+\dfrac{2}{x}-\dfrac{1}{x^{2}}\right)=1$ du fait que

$\lim\limits_{x\rightarrow +\infty}\left(\dfrac{2}{x}-\dfrac{1}{x^{2}}\right)=0$

$\lim\limits_{x\rightarrow 0}(x^{2}+1)(x^{3}+2x+1)=1$ car

$\ \lim\limits_{x\rightarrow 0}(x^{2}+1)=1\$ et

$\ \lim\limits_{x\rightarrow 0}(x^{3}+2x+1)=1$

$\lim\limits_{x\rightarrow 1^{+}}(x^{2}-1)\sqrt{\dfrac{1}{x-1}}\;,\ (F.I)$ car

$\ \lim\limits_{x\rightarrow 1^{+}}(x^{2}-1)=0\$ et

$\ \lim\limits_{x\rightarrow 1^{+}}\sqrt{\dfrac{1}{x-1}}=+\infty$

I.3.3 Limites d'un quotient

$\begin{array}{|c|c|c|c|c|c|c|c|}\hline \lim f&\infty&\ell&\ell&+\infty&+\infty&-\infty&-\infty \\ \hline \lim g&\infty&\ell'\neq 0&\infty&\ell'<0&\ell'>0&\ell'<0&\ell'>0 \\ \hline \lim\left(\dfrac{f}{g}\right)&F.I&\dfrac{\ell}{\ell'}&0&-\infty&+\infty&+\infty&-\infty \\ \hline\end{array}$

$\begin{array}{|c|c|c|c|c|c|}\hline \lim f&\ell>0&\ell>0&\ell<0&\ell<0&0 \\ \hline \lim g&0^{+}&0^{-}&0^{+}&0^{-}&0 \\ \hline \lim\left(\dfrac{f}{g}\right)&+\infty&-\infty&-\infty&+\infty& F.I \\ \hline\end{array}$

Exemple

$\lim\limits_{x\rightarrow +\infty}\dfrac{3}{x-2}=0^{+}$ car

$\ \lim\limits_{x\rightarrow +\infty}3=3\$ et

$\ \lim\limits_{x\rightarrow +\infty}(x-2)=+\infty$

$\lim\limits_{x\rightarrow 0^{-}}\dfrac{x-1}{x^{3}}=+\infty$ car

$\ \lim\limits_{x\rightarrow 0^{-}}(x-1)=-1\$ et

$\ \lim\limits_{x\rightarrow 0^{-}}x^{3}=0^{-}$

$\lim\limits_{x\rightarrow +\infty}\dfrac{x-1}{x}\;,\ (F.I)$ car

$\ \lim\limits_{x\rightarrow +\infty}(x-1)=+\infty\$ et

$\ \lim\limits_{x\rightarrow +\infty}x=+\infty$

I.4 Méthodes de calcul des limites - levée d'une forme indéterminée

Pour calculer des limites ou pour lever une indétermination on peut procéder selon les méthodes suivantes :

Méthode 1 : utiliser l'un des théorème suivants

Théorème 1

La limite en

$\pm\infty$ d'un polynôme est la limite en

$\pm\infty$ de son monôme de plus haut degré.

Théorème 2

La limite en

$\pm\infty$ d'une fraction rationnelle est la limite en

$\pm\infty$ du quotient de monôme de plus haut degré.

Exemple

$\lim\limits_{x\rightarrow +\infty}x^{2}-2x+1=\lim\limits_{x\rightarrow +\infty}x^{2}\left(1-\dfrac{2}{x}+\dfrac{1}{x^{2}}\right)=\lim\limits_{x\rightarrow +\infty}x^{2}$ car

$\ \lim\limits_{x\rightarrow +\infty}\left(1-\dfrac{2}{x}+\dfrac{1}{x^{2}}\right)=1$

donc $\lim\limits_{x\rightarrow +\infty}x^{2}-2x+1=\lim\limits_{x\rightarrow +\infty}x^{2}=+\infty$

$\lim\limits_{x\rightarrow +\infty}\dfrac{x^{3}-2x^{2}+1}{x^{2}-4x+3}=\lim\limits_{x\rightarrow +\infty}\dfrac{x^{3}}{x^{2}}=\lim\limits_{x\rightarrow +\infty}x=+\infty$

Méthode 2 : factoriser par $(x-x_{0})$

Si, pour une fonction rationnelle,

$x_{0}$ annule aussi bien le numérateur que le dénominateur, alors pour chercher la limite de cette fonction en

$x_{0}$ on factorise par

$(x-x_{0})$ au numérateur comme au dénominateur et on simplifie.

Exemple

$\begin{array}{rcl} \lim\limits_{x\rightarrow 2}\dfrac{x^{2}-4}{x^{2}-6x+8}&=&\lim\limits_{x\rightarrow 2}\dfrac{(x-2)(x+2)}{(x-2)(x-4)}\\ \\ &=&\lim\limits_{x\rightarrow 2}\dfrac{(x+2)}{(x-4)}\\ \\ &=&\lim\limits_{x\rightarrow 2}\dfrac{4}{-2}\\ \\&=&-2\end{array}$

donc

$\lim_{x\rightarrow 2}\dfrac{x^{2}-4}{x^{2}-6x+8}=-2$

Méthode 3 : multiplier par l'expression conjuguée

Pour calculer la limite d'une fraction irrationnelle en un point

$x_{0}$ annulant le numérateur et le dénominateur, on multiplie par l'expression conjuguée et on simplifie.

Exemple

$\begin{array}{rcl} \lim\limits_{x\rightarrow 2}\dfrac{\sqrt{2x+5}-3}{x-2}&=&\lim\limits_{x\rightarrow 2}\dfrac{(\sqrt{2x+5}-3)(\sqrt{2x+5}+3)}{(x-2)(\sqrt{2x+5}+3)}\\ \\ &=&\lim\limits_{x\rightarrow 2}\dfrac{2x+5-9}{(x-2)(\sqrt{2x+5}+3)}\\ \\ &=&\lim\limits_{x\rightarrow 2}\dfrac{2x-4}{(x-2)(\sqrt{2x+5}+3)}\\ \\ &=&\lim\limits_{x\rightarrow 2}\dfrac{2(x-2)}{(x-2)(\sqrt{2x+5}+3)}\\ \\ &=&\lim\limits_{x\rightarrow 2}\dfrac{2}{\sqrt{2x+5}+3}\\ \\ &=&\lim\limits_{x\rightarrow 2}\dfrac{2}{\sqrt{4+5}+3}\\ \\ &=&\lim\limits_{x\rightarrow 2}\dfrac{2}{6}\\ \\&=&\dfrac{1}{3} \end{array}$

donc

$\lim_{x\rightarrow 2}\dfrac{\sqrt{2x+5}-3}{x-2}=\dfrac{1}{3}$

Méthode 4 : utiliser les théorèmes de comparaison

Soit

$f\;,\ g\ :\ I\longrightarrow \mathbb{R}$ et soit

$x_{0}\in I$ ou

$x_{0}=\pm\infty$

Théorème 1

Si au voisinage de

$x_{0}$ on a

$f(x)\leq g(x)$ alors on a :

$\begin{array}{rcl} \text{si }\lim f(x)=+\infty&\text{alors}&\lim g(x)=+\infty\\ \\ \text{si }\lim g(x)=-\infty&\text{alors}&\lim f(x)=-\infty\end{array}$

Exemple

Soit la fonction

$f$ définie sur

$\mathbb{R}^{+}$ par

$f(x)=x-\sqrt{x}+4$

1) Montrer que

$\forall\;x\in\;[0\;;\ +\infty[\;,\ f(x)\geq 3\sqrt{x}$

2) en déduire

$\lim_{x\rightarrow +\infty}f(x)$

Résolution

$\begin{array}{rcl} f(x)-3\sqrt{x}&=&x-\sqrt{x}+4-3\sqrt{x}\\ \\ &=&x-4\sqrt{x}+4\\ \\ &=&(\sqrt{x}-2)^{2}\geq 0\end{array}$

Donc,

$f(x)-3\sqrt{x}\geq 0\ \Rightarrow\ f(x)\geq 3\sqrt{x}$

2) On a :

$\lim\limits_{x\rightarrow +\infty}3\sqrt{x}=+\infty\$ et

$\ f(x)\geq 3\sqrt{x}$ donc,

$\ \lim\limits_{x\rightarrow +\infty}f(x)=+\infty$

Théorème 2 : (Théorème des gendarmes)

Si au voisinage de

$x_{0}$ on a

$g(x)\leq f(x)\leq h(x)$ et que

$\lim\limits_{x\rightarrow x_{0}}g(x)=\lim\limits_{x\rightarrow x_{0}}h(x)=\ell$ (finie ou infinie) alors

$\lim\limits_{x\rightarrow x_{0}}f(x)=\ell$

Exemple

Calculer

$\lim\limits_{x\rightarrow 0^{+}}x^{2}\cos\dfrac{1}{x}$

Nous avons ;

$-1\leq\cos\dfrac{1}{x}\leq 1\;,\ \forall\;x\ \Rightarrow\ -x^{2}\leq x^{2}\cos\dfrac{1}{x}\leq x^{2}$

Or,

$\ \left.\begin{array}{lcl}\lim\limits_{x\rightarrow 0^{+}}-x^{2}&=&0 \\ \lim\limits_{x\rightarrow 0^{+}}x^{2}&=&0\end{array}\right\rbrace$ donc,

$\ \lim\limits_{x\rightarrow 0^{+}}x^{2}\cos\dfrac{1}{x}=0$

Corollaire du théorème des gendarmes

Si au voisinage de

$x_{0}$ on a :

$|f(x)-\ell|\leq g(x)$ et que

$\lim\limits_{x\rightarrow x_{0}}g(x)=0$ alors,

$\ \lim\limits_{x\rightarrow x_{0}}f(x)=\ell$

Méthode 5 : utiliser le théorème de composée de fonctions

Théorème

Soit deux fonctions

$f\$ et

$\ g$ telles que :

$f\ :\ I\longrightarrow\mathbb{R}\;,\ g\ :\ J\longrightarrow\mathbb{R}$

$I\$ et

$\ J$ sont deux intervalles de

$\mathbb{R}$ tels que

$f(I)\subset J$ et soit

$x_{0}\;,\ \ell\;,\ \ell'$ finis ou infinis, on a :

$\lim\limits_{x\rightarrow x_{0}}f(x)=\ell$ et si

$\lim\limits_{x\rightarrow \ell}g(x)=\ell'$ alors,

$\ \lim\limits_{x\rightarrow x_{0}}g\circ f(x)=\ell'$

Exemple

a) Soit

$h(x)=\sqrt{\dfrac{4x+5}{x-2}}$ , calculer

$\lim\limits_{x\rightarrow +\infty}h(x)$

Posons

$f(x)=\dfrac{4x+5}{x-2}\;,\ g(x)=\sqrt{x}$ et

$h(x)=g\circ f(x)$

alors,

$g\circ f(x)=g[f(x)]=\sqrt{f(x)}=\sqrt{\dfrac{4x+5}{x-2}}$

On a :

$\lim\limits_{x\rightarrow +\infty}\dfrac{4x+5}{x-2}=\lim\limits_{x\rightarrow +\infty}\dfrac{4x}{4}=4$

donc,

$\begin{array}{rcl} \lim\limits_{x\rightarrow +\infty}\sqrt{\dfrac{4x+5}{x-2}}&=&\lim\limits_{x\rightarrow 4}g(x)\\\\&=&\lim\limits_{x\rightarrow 4}\sqrt{x}\\ \\&=&\lim\limits_{x\rightarrow 4}\sqrt{4}\\ \\&=&2\end{array}$

donc,

$\lim_{x\rightarrow +\infty}\sqrt{\dfrac{4x+5}{x-2}}=2$

b) On donne

$h(x)=\sqrt{\dfrac{x+9}{x-2}}$ , calculer

$\lim\limits_{x\rightarrow 2^{+}}h(x)$

Soit

$f(x)=\dfrac{x+9}{x-2}\;,\ g(x)=\sqrt{x}$ et

$h(x)=g\circ f(x)$

On a :

$\lim\limits_{x\rightarrow 2^{+}}\dfrac{x+9}{x-2}=\lim\limits_{x\rightarrow 2^{+}}\dfrac{11}{0^{+}}=+\infty$

donc,

$\begin{array}{rcl} \lim\limits_{x\rightarrow 2^{+}}\sqrt{\dfrac{x+9}{x-2}}&=&\lim\limits_{x\rightarrow +\infty}g(x)\\ \\ &=&\lim\limits_{x\rightarrow +\infty}\sqrt{x}\\ \\ &=&+\infty\end{array}$

D'où,

$\lim_{x\rightarrow 2^{+}}\sqrt{\dfrac{x+9}{x-2}}=+\infty$

I.5 Notion d'asymptotes

Soit

$f$ une fonction de courbe représentative

$(C_{f})$ .

I.5.1 Asymptote horizontale

$\lim\limits_{x\rightarrow -\infty}f(x)=\ell$ ou

$\ \lim\limits_{x\rightarrow +\infty}f(x)=\ell$ alors on dira que

$(C_{f})$ admet une asymptote horizontale

$\Delta$ d'équation

$y=\ell$

$(C_{f})$ s'approche de plus en plus de la droite

$\Delta$ d'équation

$y=\ell$ lorsque

$x$ devient infini.

I.5.2 Asymptote verticale

$\lim\limits_{x\rightarrow x_{0}^{-}}f(x)=-\infty\text{ ou }(+\infty)$ ou

$\ \lim\limits_{x\rightarrow x_{0}^{+}}f(x)=-\infty\text{ ou }(+\infty)$ alors on dit que

$(C_{f})$ admet une asymptote verticale

$\Delta'$ d'équation

$x=x_{0}$

$\centerdot\ \ \lim\limits_{x\rightarrow x_{0}^{+}}f(x)=-\infty$

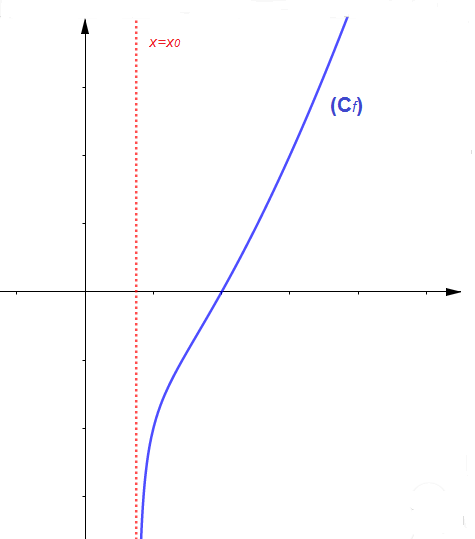
$\centerdot\ \ \lim\limits_{x\rightarrow x_{0}^{-}}f(x)=-\infty$

Exemple

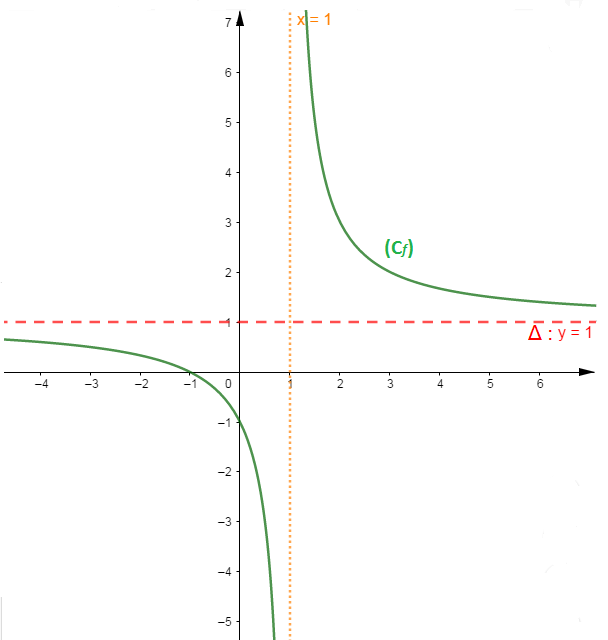
Soit

$f(x)=\dfrac{x+1}{x-1}$ , on a

$D_{f}=\mathbb{R}\setminus\{1\}=]-\infty\;;\ 1[\cup]1\;;\ +\infty[$

$\lim\limits_{x\rightarrow -\infty}f(x)=\lim\limits_{x\rightarrow +\infty}f(x)=1$ , donc la droite

$\Delta$ d'équation

$y=1$ est asymptote horizontale à

$(C_{f})$

$\lim\limits_{x\rightarrow 1^{-}}f(x)=\lim\limits_{x\rightarrow 1^{-}}\dfrac{x+1}{x-1}=\lim\limits_{x\rightarrow 1^{-}}\dfrac{2}{0^{-}}=-\infty$

$\lim\limits_{x\rightarrow 1^{+}}f(x)=\lim\limits_{x\rightarrow 1^{+}}\dfrac{x+1}{x-1}=\lim\limits_{x\rightarrow 1^{+}}\dfrac{2}{0^{+}}=+\infty$ donc la droite

$\Delta'$ d'équation

$x=1$ est asymptote verticale à

$(C_{f})$

illustration graphique

I.5.3 Asymptote oblique

S'il existe une fonction affine

$x\mapsto ax+b\;;\ (a\neq 0)$ telle que

$\lim\limits_{x\rightarrow \infty}[f(x)-(ax+b)]=0$ , on dit que

$(C_{f})$ admet une asymptote oblique d'équation

$y=ax+b$

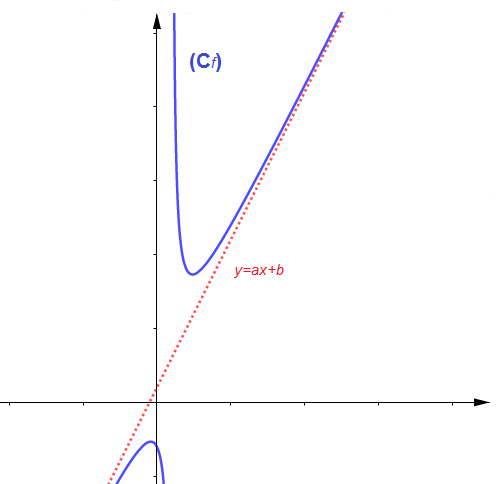
Exemple

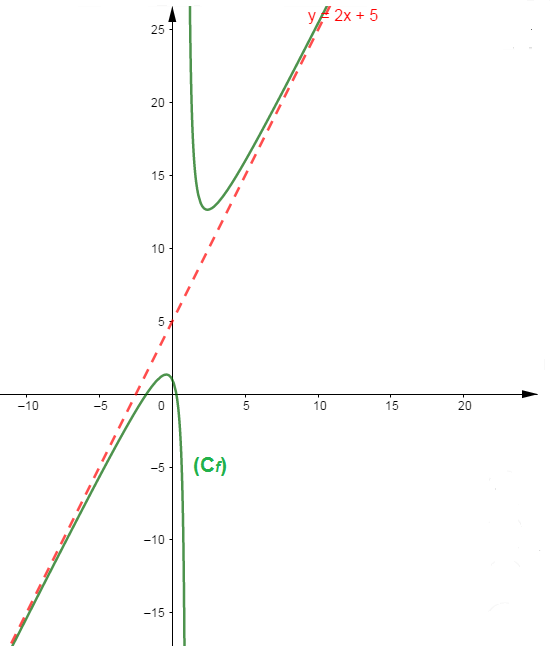
Soit

$f$ la fonction définie par

$f(x)=\dfrac{2x^{2}+3x-1}{x-1}$

$D_{f}=\mathbb{R}\setminus\{1\}=]-\infty\;;\ 1[\cup]1\;;\ +\infty[\$ et

$\ \forall\;x\in D_{f}\;,\ f(x)=2x+5+\dfrac{4}{x-1}$

Montrons que la droite d'équation

$y=2x+5$ est asymptote oblique à

$(C_{f})$ .

On a :

$f(x)-y=2x+5+\dfrac{4}{x-1}-(2x+5)=\dfrac{4}{x-1}$

$\lim\limits_{x\rightarrow -\infty}[f(x)-y]=\lim\limits_{x\rightarrow -\infty}\dfrac{4}{x-1}=0^{-}$ et

$\lim\limits_{x\rightarrow +\infty}[f(x)-y]=\lim\limits_{x\rightarrow +\infty}\dfrac{4}{x-1}=0^{+}$ donc la droite d'équation

$y=2x+5$ est asymptote oblique à

$(C_{f})$ au voisinage de

$-\infty$ et de

$+\infty.$

illustration graphique

Détermination pratique de $a$ et $b$

Dans l'équation

$y=ax+b$ , pour déterminer les coefficients

$a\$ et

$\ b$ on procède comme suit :

calculer :

$\lim\limits_{x\rightarrow \infty}\dfrac{f(x)}{x}=a\$ et

$\ \lim\limits_{x\rightarrow \infty}f(x)-ax=b$

Exemple

$f(x)=\dfrac{2x^{2}+3x-1}{x-1}$

$\begin{array}{rcl} \lim_{x\rightarrow \infty}\dfrac{f(x)}{x}&=&\lim\limits_{x\rightarrow \infty}\dfrac{\dfrac{2x^{2}+3x-1}{x-1}}{x}\\ \\&=&\lim\limits_{x\rightarrow \infty}\dfrac{2x^{2}+3x-1}{(x-1)}\times\dfrac{1}{x}\\ \\&=&\lim\limits_{x\rightarrow \infty}\dfrac{2x^{2}+3x-1}{x^{2}-x}\\ \\&=&\lim\limits_{x\rightarrow \infty}\dfrac{2x^{2}}{x^{2}}\\ \\ &=&2\end{array}$

Donc,

$\lim\limits_{x\rightarrow \infty}\dfrac{f(x)}{x}=2\ \Rightarrow\ a=2$

Ainsi,

$\begin{array}{rcl} \lim\limits_{x\rightarrow \infty}f(x)-2x&=&\lim\limits_{x\rightarrow \infty}\dfrac{2x^{2}+3x-1}{x-1}-2x\\ \\ &=&\lim\limits_{x\rightarrow \infty}\dfrac{2x^{2}+3x-1-2x^{2}+2x}{x-1}\\ \\ &=&\lim\limits_{x\rightarrow \infty}\dfrac{5x-1}{x-1}\\ \\ &=&\lim\limits_{x\rightarrow \infty}\dfrac{5x}{x}\\ \\ &=&5\end{array}$

Donc

$\lim\limits_{x\rightarrow \infty}f(x)-2x=5\ \Rightarrow\ b=5$ , d'où la droite d'équation

$y=2x+5$ est asymptote oblique à

$(C_{f})$ au voisinage de

$\pm\infty.$

II Continuité

II.1 Définition

Soit

$f\ :\ I\longrightarrow\mathbb{R}$ et

$x_{0}\in I$

$\centerdot\ \$ On dit que

$f$ est continue en

$x_{0}$ si, et seulement si,

$\lim_{x\rightarrow x_{0}}f(x)=f(x_{0})$

$\centerdot\ \$ On dit que

$f$ est continue à gauche de

$x_{0}$ si, et seulement si,

$\lim_{x\rightarrow x_{0}^{-}}f(x)=f(x_{0})$

$\centerdot\ \$ On dit que

$f$ est continue à droite de

$x_{0}$ si, et seulement si,

$\lim_{x\rightarrow x_{0}^{+}}f(x)=f(x_{0})$

Théorème 1

Soit

$f\ :\ I\longrightarrow\mathbb{R}\$ et

$\ x_{0}\in I$

On dit que

$f$ est continue en

$x_{0}$ si, et seulement si,

$f$ est continue à gauche et à droite de

$x_{0}$ , c'est-à-dire

$\left\lbrace\begin{array}{lcl}\lim\limits_{x\rightarrow x_{0}^{-}}f(x)&=&f(x_{0}) \\ \lim\limits_{x\rightarrow x_{0}^{+}}f(x)&=&f(x_{0})\end{array}\right.$

Exemple

Soit

$f$ la fonction définie par

$f(x)=\left\lbrace\begin{array}{lcl}\dfrac{\sqrt{1+x^{2}}-1}{2x}&\text{si}&x>0 \\ \\ 2x-1&\text{si}&x\leq 0\end{array}\right.$

Étudier la continuité de

$f$ en

$0.$

Résolution

$\begin{array}{rcl} \lim\limits_{x\rightarrow 0^{+}}f(x)&=&\lim\limits_{x\rightarrow 0^{+}}\dfrac{\sqrt{1+x^{2}}-1}{2x}\\ \\ &=&\lim\limits_{x\rightarrow 0^{+}}\dfrac{(\sqrt{1+x^{2}}-1)(\sqrt{1+x^{2}}+1)}{2x(\sqrt{1+x^{2}}+1)}\\ \\ &=&\lim\limits_{x\rightarrow 0^{+}}\dfrac{1+x^{2}-1}{2x(\sqrt{1+x^{2}}+1)}\\ \\ &=&\lim\limits_{x\rightarrow 0^{+}}\dfrac{x^{2}}{2x(\sqrt{1+x^{2}}+1)}\nonumber \\ &=&\lim\limits_{x\rightarrow 0^{+}}\dfrac{x}{2(\sqrt{1+x^{2}}+1)}\\ \\ &=&\lim\limits_{x\rightarrow 0^{+}}\dfrac{0}{4}=0\end{array}$

Or,

$\ f(0)=-1=\lim\limits_{x\rightarrow 0^{-}}f(x)$ donc,

$\ \lim\limits_{x\rightarrow 0^{-}}f(x)\neq\lim\limits_{x\rightarrow 0^{+}}f(x)$

ainsi,

$f$ n'est pas continue en

$0.$

Théorème 2

Soient

$f\$ et

$\ g$ deux fonctions continues en un point

$x_{0}\$ et

$\ \alpha$ un réel ; alors les fonctions

$\alpha f\;,\ (f+g)$ et

$(f\times g)$ sont continues en

$x_{0}.$

Si de plus

$g(x_{0}\neq 0$ alors les fonctions

$\dfrac{1}{g}\$ et

$\ \dfrac{f}{g}$ sont aussi continues en

$x_{0}.$

II.2 Fonction continue sur un intervalle

Définition

Une fonction

$f$ est continue sur un intervalle

$I$ de

$\mathbb{R}$ si elle est continue en tout point de

$I.$

Théorème 1

Toute fonction polynôme est continue sur

$\mathbb{R}$

Toute fonction rationnelle est continue sur son domaine de définition.

La fonction

$\sqrt{U}$ est continue sur son domaine de définition.

La fonction

$|U|$ est continue sur son domaine de définition.

Théorème 2

Soient

$f\$ et

$\ g$ deux fonctions telles que

$f$ soit continue sur un intervalle

$I\$ et

$\ g$ continue sur un intervalle

$J$ contenant

$f(I)$ alors

$g\circ f$ est continue sur

$I.$

Prolongement par continuité d'une fonction

Soit

$\begin{eqnarray} f\ :\ \mathbb{R}&\longrightarrow&\mathbb{R}\nonumber \\ x&\longrightarrow&\dfrac{x^{2}-1}{x-1}\nonumber \end{eqnarray}$

$D_{f}=\mathbb{R}\setminus\{1\}\;,\ 1\notin D_{f}$ ;

$f$ n'est pas continue au point 1.

$\lim\limits_{x\rightarrow 1}f(x)=F.I$

On a :

$\forall\;x\in\mathbb{R}\setminus\{1\}\;,\ f(x)=\dfrac{(x-1)(x+1)}{x-1}=x+1$

donc,

$\lim\limits_{x\rightarrow 1}f(x)=\lim\limits_{x\rightarrow 1}x+1=2$

Soit alors

$g$ la fonction définie par

$\left\lbrace\begin{array}{rcl} g(x)&=&x+1\quad\text{si }x\neq 1 \\ g(1)&=&2 \end{array}\right.$

$\lim\limits_{x\rightarrow 1}g(x)=\lim\limits_{x\rightarrow 1}x+1=2$ donc,

$\ \lim\limits_{x\rightarrow 1}g(x)=g(1)$

ainsi,

$g$ est continue au point 1. D'où

$g$ est appelé le prolongement par continuité de

$f$ au point

$1.$

Auteur:

Diny Faye & Seyni Ndiaye

Commentaires

M Pouye (non vérifié)

lun, 01/11/2021 - 07:13

Permalien

C'est vraiment un très bon

C'est vraiment un très bon cours

répondre

Anonyme (non vérifié)

ven, 02/05/2021 - 13:24

Permalien

Cours très intéressants.

Cours très intéressants. Merci

répondre

Anonyme (non vérifié)

ven, 02/05/2021 - 13:24

Permalien

Cours très intéressants.

Cours très intéressants. Merci

répondre

Nafiu (non vérifié)

ven, 05/07/2021 - 01:28

Permalien

Les cours ne se terminent pas

Les cours ne se terminent pas et sa me pose des problemes

répondre

Anonyme (non vérifié)

jeu, 07/22/2021 - 13:34

Permalien

Cours tres complet

répondre

Baye Kebe (non vérifié)

lun, 05/23/2022 - 01:08

Permalien

Besoin d'aide sur svt,pc et maths

Bonjour je me nomme baye kebe Je fais la 1er S2 au lycée de Tendouck Je cheche quelqu'un(e) qui m'explique les cours et exercices Car parfois je n'arrive pas à suivre le rytheme des professeurs Voici mon numero 772388565 J'ai vraiment besoin d'aide.

répondre

Ajouter un commentaire

form.antibot { display: none !important; } You must have JavaScript enabled to use this form.

Vous êtes ici

Limites et Continuité - 1er S

Formulaire de recherche

I Limites

I.1 Limites à l'infinie

I.1.1 Limites infinies à l'infinie

Définitions intuitives

I.1.2 Limites finies à l'infinie

Définitions intuitives

Remarque

I.2 Limites en un point x_{0}x_{0}

Activité

I.2.1 Limite à gauche - limite à droite de x_{0}x_{0}

Définitions

Théorème

I.2.2 Limites infinies en x_{0}x_{0}

Définitions intuitives

I.2.3 Limites finies en x_{0}x_{0}

Définition

Travaux pratiques

Résolution

I.3 Opération sur les limites

I.3.1 Limites d'une somme de deux fonctions

Exemple

I.3.2 Limites d'un produit

Exemple

I.3.3 Limites d'un quotient

Exemple

I.4 Méthodes de calcul des limites - levée d'une forme indéterminée

Méthode 1 : utiliser l'un des théorème suivants

Théorème 1

Théorème 2

Exemple

Méthode 2 : factoriser par (x-x_{0})(x-x_{0})

Exemple

Méthode 3 : multiplier par l'expression conjuguée

Exemple

Méthode 4 : utiliser les théorèmes de comparaison

Théorème 1

Exemple

Résolution

Théorème 2 : (Théorème des gendarmes)

Exemple

Corollaire du théorème des gendarmes

Méthode 5 : utiliser le théorème de composée de fonctions

Théorème

Exemple

I.5 Notion d'asymptotes

I.5.1 Asymptote horizontale

I.5.2 Asymptote verticale

Exemple

illustration graphique

I.5.3 Asymptote oblique

Exemple

illustration graphique

Détermination pratique de aa et bb

Exemple

II Continuité

II.1 Définition

Théorème 1

Exemple

Résolution

Théorème 2

II.2 Fonction continue sur un intervalle

Définition

Théorème 1

Théorème 2

Prolongement par continuité d'une fonction

Commentaires

C'est vraiment un très bon

Cours très intéressants.

Cours très intéressants.

Les cours ne se terminent pas

Cours tres complet

Besoin d'aide sur svt,pc et maths

Ajouter un commentaire

Plain text

I.2 Limites en un point $x_{0}$

I.2.1 Limite à gauche - limite à droite de $x_{0}$

I.2.2 Limites infinies en $x_{0}$

I.2.3 Limites finies en $x_{0}$

Méthode 2 : factoriser par $(x-x_{0})$

Détermination pratique de $a$ et $b$