Limites et Continuité - 1er S
Classe:
Première
I Limites
I.1 Limites à l'infinie
I.1.1 Limites infinies à l'infinie
Considérons la fonction f définie par f(x)=x2 ; son ensemble de définition est Df=R.
Examinons le tableau suivant : x−1018−109−104−100−10−10110102104106f(x)103610181081041021011021041081012
Nous constatons que lorsque x prend des valeurs de plus en plus grandes, les valeurs de f(x) deviennent de plus en plus grandes. On dira que f(x) tend vers +∞ quand x tend vers +∞.
De même lorsque x prend des valeurs de plus en plus grandes en valeurs absolues mais en étant négatives, f(x) prend des valeurs de plus en plus grandes. On dira que f(x) tend vers +∞ quand x tend vers −∞.
Définitions intuitives
Soit f une fonction définie sur un domaine Df.
⋅ Lorsque x tend vers +∞, si les f(x) tendent vers +∞ alors on dira que la limite de f(x) quand x tend vers +∞ est égale à +∞ et on note : limx→+∞f(x)=+∞
⋅ Lorsque x tend vers −∞, si les f(x) tendent vers +∞ alors on dira que la limite de f(x) quand x tend vers −∞ est égale à +∞ et on note : limx→−∞f(x)=+∞
⋅ Lorsque x tend vers +∞, si les f(x) tendent vers −∞ alors on dira que la limite de f(x) quand x tend vers +∞ est égale à −∞ et on note : limx→+∞f(x)=−∞
⋅ Lorsque x tend vers −∞, si les f(x) tendent vers −∞ alors on dira que la limite de f(x) quand x tend vers −∞ est égale à −∞ et on note : limx→−∞f(x)=−∞
I.1.2 Limites finies à l'infinie
Considérons la fonction f définie sur Df=R∗ par f(x)=1x3.
Soit le tableau de valeurs suivant : x−104−102−1010102104106f(x)−10−12−0.000001−0.0010.0010.00000110−1210−18 En examinant ce tableau, nous constatons que lorsque x prend des valeurs de plus en plus grandes, les valeurs de f(x) restent très proches de 0. On dira que f(x) tend vers 0 quand x tend vers +∞.
De même, lorsque x prend des valeurs de plus en plus grandes en valeurs absolues, tout en étant négatives, les valeurs de f(x) deviennent de plus en plus proches de la valeur 0. On dira que f(x) tend vers 0 quand x tend vers −∞.
Définitions intuitives
Soit f une fonction définie sur un domaine Df et ℓ∈R.
⋅ Lorsque x tend vers +∞, si les f(x) tendent vers un réel ℓ alors on dira que la limite de f(x) quand x tend vers +∞ est égale à ℓ et on note : limx→+∞f(x)=ℓ
⋅ Lorsque x tend vers −∞, si les f(x) tendent vers un réel ℓ alors on dira que la limite de f(x) quand x tend vers −∞ est égale à ℓ et on note : limx→−∞f(x)=ℓ
Remarque
La limite lorsqu'elle existe, elle est unique.
I.2 Limites en un point x0
Activité
Soit f une fonction dont la courbe représentative Cf est illustrée sur la figure ci-dessous.
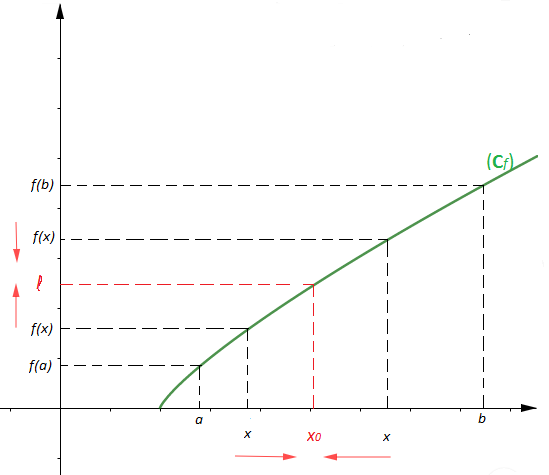
En examinant ce graphique nous pouvons constater que les valeurs de f(x) tendent vers L lorsque x tend vers x0 de part et d'autre.
Cet fait peut être exprimer en disant que f(x) a pour limite ℓ lorsque x tend vers x0 et on note : limx→x0f(x)=ℓ
I.2.1 Limite à gauche - limite à droite de x0
Définitions
Soit f une fonction d'ensemble de définition Df et x0 un réel.
⋅ La limite éventuelle de la restriction de f à Df∩]−∞; x0[ est appelée limite de f à gauche au point x0 ou encore la limite de f lorsque x tend vers x0 en étant inférieur à x0, et on note : limx→x0x<x0f(x)ou plus simplementlimx→x−0f(x)
⋅ La limite éventuelle de la restriction de f à Df∩]x0; +∞[ est appelée limite de f à droite au point x0 ou encore la limite de f lorsque x tend vers x0 en étant supérieur à x0, et on note : limx→x0x>x0f(x)ou plus simplementlimx→x+0f(x)
Théorème
On dit que f admet une limite fini ℓ en x0 si et seulement si limx→x−0f(x)=limx→x+0f(x)=ℓ
I.2.2 Limites infinies en x0
Soit la fonction f définie par f(x)=1x;Df=R∗.
Examinons le tableau suivant : x−0.1−0.01−10−4−10−8⋯0⋯10−610−30.010.1f(x)−10−100−104−108⋯×⋯10610310010
Nous constatons que lorsque x prend des valeurs de plus en plus proches de 0 en étant supérieures à 0, les valeurs de f(x) deviennent de plus en plus grandes. On dira que f(x) tend vers +∞ quand x tend vers 0+. On note : limx→0+f(x)=+∞
De même lorsque x prend des valeurs de plus en plus proches de 0 en étant inférieures à 0, f(x) prend des valeurs de plus en plus grandes en valeurs absolues mais en étant négatives. On dira que f(x) tend vers −∞ quand x tend vers 0−. On note : limx→0−f(x)=−∞
Définitions intuitives
Soit f une fonction définie sur un domaine Df et x0 appartenant à l'intérieur de ce domaine :
⋅ Lorsque x tend vers x0, si les f(x) tendent vers +∞ alors on dira que la limite de f(x) quand x tend vers x0 est égale à +∞ et on note : limx→x0f(x)=+∞
⋅ Lorsque x tend vers x0, si les f(x) tendent vers −∞ alors on dira que la limite de f(x) quand x tend vers x0 est égale à −∞ et on note : limx→x0f(x)=−∞
I.2.3 Limites finies en x0
Soit f la fonction définie par f(x)=x2−4x−2. On a Df=R∖{2}.
Considérons le tableau suivant : x1.81.91.991.9992.000012.00012.0012.012.1f(x)3.83.93.993.9994.000014.00014.0014.014.1
Nous constatons que lorsque x prend des valeurs de plus en plus proches de 2 en restant dans Df, les valeurs de f(x) semblent s'approcher de la valeur 4. On exprime ce fait en disant que f(x) tend vers 4 quand x tend vers 2. On note : limx→2f(x)=4
Définition
Soit f une fonction définie sur un domaine D, x0 appartenant à l'intérieur de ce domaine et ℓ un réel. Lorsque x prend des valeurs proches de x0, si les valeurs de f(x) deviennent de plus en plus proches du réel ℓ alors on dira que f(x) a pour limite ℓ lorsque x tend vers x0 et on note : limx→x0f(x)=ℓ
Travaux pratiques
Déterminer la limite des fonctions suivantes au point x0=1
a) f(x)={2x+1six<13x2−x−1six>1
b) g(x)={x2−1six<13xx2+1six>1
c) h(x)={2xx−1six>1−x2(x−1)2six<1
Résolution
a) limx→1−f(x)=limx→1−2x+1=2×1+1=3
limx→1+f(x)=limx→1+3x2−x−1=3×(1)2−1−1=1
b) limx→1−g(x)=limx→1−x2−1=1−1=0
limx→1+g(x)=limx→1+3xx2+1=3×112+1=32
c) limx→1+h(x)=limx→1+2xx−1 or lorsque x tend vers 1+, (x−1) tend vers 0+.
Donc, limx→1+2xx−1=2(x−1)→0+=+∞.
D'où, limx→1+h(x)=+∞
limx→1−h(x)=limx→1−−x2(x−1)2 or lorsque x tend vers 1−, (x−1) tend vers 0− et donc (x−1)2 va tendre vers 0+.
Ainsi, limx→1−−x2(x−1)2=−1(x−1)2→0+=−∞.
D'où, limx→1−h(x)=−∞
I.3 Opération sur les limites
Soient f et g deux fonctions de limites finies ou non. Les limites de f+g, f×g et de fg sont récapitulées dans les tableaux ci-dessous.
N.B : Dans les cas où on ne peut conclure, on dira qu'on est en face d'une indétermination ou simplement une "forme indéterminée" notée (F.I)
I.3.1 Limites d'une somme de deux fonctions
limfℓℓℓ+∞−∞+∞limgℓ′+∞−∞+∞−∞−∞lim(f+g)ℓ+ℓ′+∞−∞+∞−∞F.I
Exemple
a) limx→+∞(x+1−1x3)=+∞ car en posant f(x)=x+1 et g(x)=−1x3 on a limx→+∞f(x)=+∞ et limx→+∞g(x)=0.
Donc, limx→+∞(f(x)+g(x))=+∞
b) limx→0−(x+1−1x3)=+∞ car limx→0−(x+1)=1 et limx→0−−1x3=+∞
c) limx→+∞(x3−x2), (F.I) car limx→+∞x3=+∞ et limx→+∞−x2=−∞
I.3.2 Limites d'un produit
limfℓℓ>0ℓ<0ℓ>0ℓ<0+∞−∞+∞0limgℓ′+∞+∞−∞−∞+∞−∞−∞∞lim(f×g)ℓ×ℓ′+∞−∞−∞+∞+∞+∞−∞F.I
Exemple
a) limx→+∞(x2+2x−3)=limx→+∞x2(1+2x−3x2)=+∞
car limx→+∞x2=+∞ et limx→+∞(1+2x−1x2)=1 du fait que limx→+∞(2x−1x2)=0
b) limx→0(x2+1)(x3+2x+1)=1 car limx→0(x2+1)=1 et limx→0(x3+2x+1)=1
c) limx→1+(x2−1)√1x−1, (F.I) car limx→1+(x2−1)=0 et limx→1+√1x−1=+∞
I.3.3 Limites d'un quotient
limf∞ℓℓ+∞+∞−∞−∞limg∞ℓ′≠0∞ℓ′<0ℓ′>0ℓ′<0ℓ′>0lim(fg)F.Iℓℓ′0−∞+∞+∞−∞
limfℓ>0ℓ>0ℓ<0ℓ<00limg0+0−0+0−0lim(fg)+∞−∞−∞+∞F.I
Exemple
a) limx→+∞3x−2=0+ car limx→+∞3=3 et limx→+∞(x−2)=+∞
b) limx→0−x−1x3=+∞ car limx→0−(x−1)=−1 et limx→0−x3=0−
c) limx→+∞x−1x, (F.I) car limx→+∞(x−1)=+∞ et limx→+∞x=+∞
I.4 Méthodes de calcul des limites - levée d'une forme indéterminée
Pour calculer des limites ou pour lever une indétermination on peut procéder selon les méthodes suivantes :
Méthode 1 : utiliser l'un des théorème suivants
Théorème 1
La limite en ±∞ d'un polynôme est la limite en ±∞ de son monôme de plus haut degré.
Théorème 2
La limite en ±∞ d'une fraction rationnelle est la limite en ±∞ du quotient de monôme de plus haut degré.
Exemple
a) limx→+∞x2−2x+1=limx→+∞x2(1−2x+1x2)=limx→+∞x2 car limx→+∞(1−2x+1x2)=1
donc limx→+∞x2−2x+1=limx→+∞x2=+∞
b) limx→+∞x3−2x2+1x2−4x+3=limx→+∞x3x2=limx→+∞x=+∞
Méthode 2 : factoriser par (x−x0)
Si, pour une fonction rationnelle, x0 annule aussi bien le numérateur que le dénominateur, alors pour chercher la limite de cette fonction en x0 on factorise par (x−x0) au numérateur comme au dénominateur et on simplifie.
Exemple
limx→2x2−4x2−6x+8=limx→2(x−2)(x+2)(x−2)(x−4)=limx→2(x+2)(x−4)=limx→24−2=−2
donc limx→2x2−4x2−6x+8=−2
Méthode 3 : multiplier par l'expression conjuguée
Pour calculer la limite d'une fraction irrationnelle en un point x0 annulant le numérateur et le dénominateur, on multiplie par l'expression conjuguée et on simplifie.
Exemple
limx→2√2x+5−3x−2=limx→2(√2x+5−3)(√2x+5+3)(x−2)(√2x+5+3)=limx→22x+5−9(x−2)(√2x+5+3)=limx→22x−4(x−2)(√2x+5+3)=limx→22(x−2)(x−2)(√2x+5+3)=limx→22√2x+5+3=limx→22√4+5+3=limx→226=13
donc limx→2√2x+5−3x−2=13
Méthode 4 : utiliser les théorèmes de comparaison
Soit f, g : I⟶R et soit x0∈I ou x0=±∞
Théorème 1
Si au voisinage de x0 on a f(x)≤g(x) alors on a :
si limf(x)=+∞alorslimg(x)=+∞si limg(x)=−∞alorslimf(x)=−∞
Exemple
Soit la fonction f définie sur R+ par f(x)=x−√x+4
1) Montrer que ∀x∈[0; +∞[, f(x)≥3√x
2) en déduire limx→+∞f(x)
Résolution
1)
f(x)−3√x=x−√x+4−3√x=x−4√x+4=(√x−2)2≥0
Donc, f(x)−3√x≥0 ⇒ f(x)≥3√x
2) On a : limx→+∞3√x=+∞ et f(x)≥3√x donc, limx→+∞f(x)=+∞
Théorème 2 : (Théorème des gendarmes)
Si au voisinage de x0 on a g(x)≤f(x)≤h(x) et que limx→x0g(x)=limx→x0h(x)=ℓ (finie ou infinie) alors limx→x0f(x)=ℓ
Exemple
Calculer limx→0+x2cos1x
Nous avons ; −1≤cos1x≤1, ∀x ⇒ −x2≤x2cos1x≤x2
Or, limx→0+−x2=0limx→0+x2=0} donc, limx→0+x2cos1x=0
Corollaire du théorème des gendarmes
Si au voisinage de x0 on a : |f(x)−ℓ|≤g(x) et que limx→x0g(x)=0 alors, limx→x0f(x)=ℓ
Méthode 5 : utiliser le théorème de composée de fonctions
Théorème
Soit deux fonctions f et g telles que : f : I⟶R, g : J⟶R
I et J sont deux intervalles de R tels que f(I)⊂J et soit x0, ℓ, ℓ′ finis ou infinis, on a :
Si limx→x0f(x)=ℓ et si limx→ℓg(x)=ℓ′ alors, limx→x0g∘f(x)=ℓ′
Exemple
a) Soit h(x)=√4x+5x−2, calculer limx→+∞h(x)
Posons f(x)=4x+5x−2, g(x)=√x et h(x)=g∘f(x)
alors, g∘f(x)=g[f(x)]=√f(x)=√4x+5x−2
On a : limx→+∞4x+5x−2=limx→+∞4x4=4
donc,
limx→+∞√4x+5x−2=limx→4g(x)=limx→4√x=limx→4√4=2
donc, limx→+∞√4x+5x−2=2
b) On donne h(x)=√x+9x−2, calculer limx→2+h(x)
Soit f(x)=x+9x−2, g(x)=√x et h(x)=g∘f(x)
On a : limx→2+x+9x−2=limx→2+110+=+∞
donc,
limx→2+√x+9x−2=limx→+∞g(x)=limx→+∞√x=+∞
D'où, limx→2+√x+9x−2=+∞
I.5 Notion d'asymptotes
Soit f une fonction de courbe représentative (Cf).
I.5.1 Asymptote horizontale
Si limx→−∞f(x)=ℓ ou limx→+∞f(x)=ℓ alors on dira que (Cf) admet une asymptote horizontale Δ d'équation y=ℓ

(Cf) s'approche de plus en plus de la droite Δ d'équation y=ℓ lorsque x devient infini.
I.5.2 Asymptote verticale
Si limx→x−0f(x)=−∞ ou (+∞) ou limx→x+0f(x)=−∞ ou (+∞) alors on dit que (Cf) admet une asymptote verticale Δ′ d'équation x=x0
⋅ limx→x+0f(x)=−∞
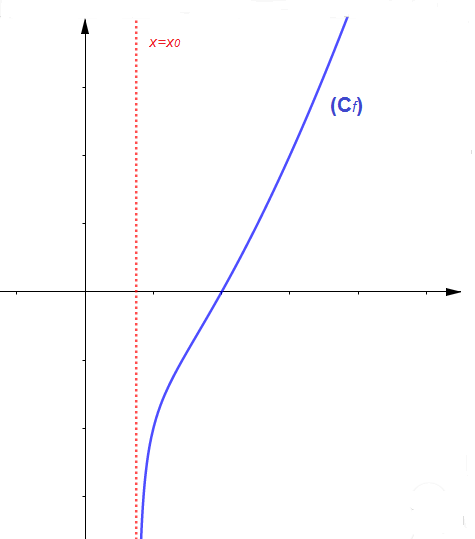
⋅ limx→x−0f(x)=−∞

Exemple
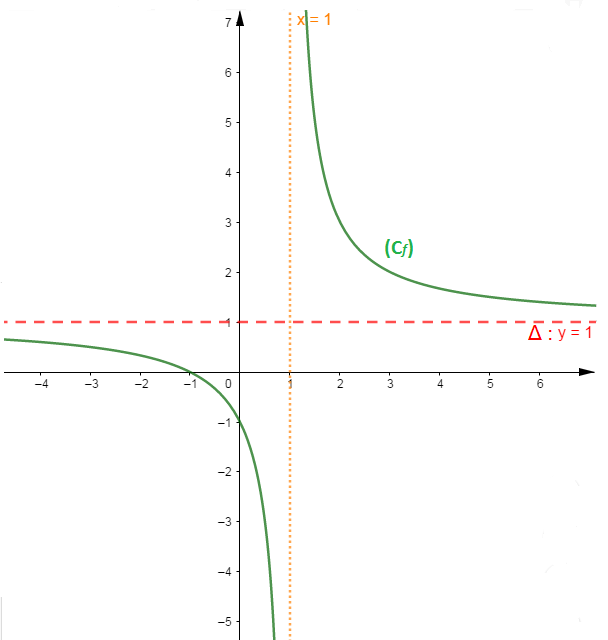
Soit f(x)=x+1x−1, on a Df=R∖{1}=]−∞; 1[∪]1; +∞[
limx→−∞f(x)=limx→+∞f(x)=1, donc la droite Δ d'équation y=1 est asymptote horizontale à (Cf)
limx→1−f(x)=limx→1−x+1x−1=limx→1−20−=−∞
limx→1+f(x)=limx→1+x+1x−1=limx→1+20+=+∞ donc la droite Δ′ d'équation x=1 est asymptote verticale à (Cf)
illustration graphique
I.5.3 Asymptote oblique
S'il existe une fonction affine x↦ax+b; (a≠0) telle que limx→∞[f(x)−(ax+b)]=0, on dit que (Cf) admet une asymptote oblique d'équation y=ax+b
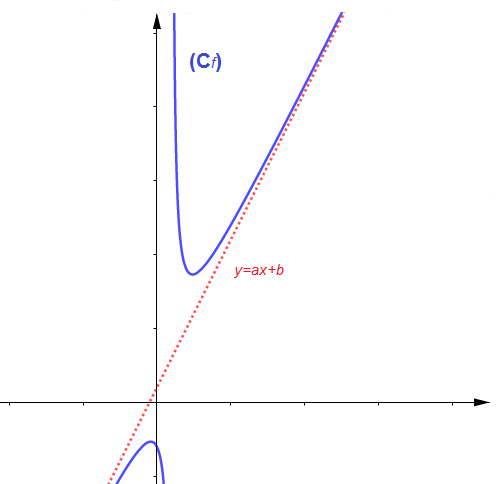
Exemple
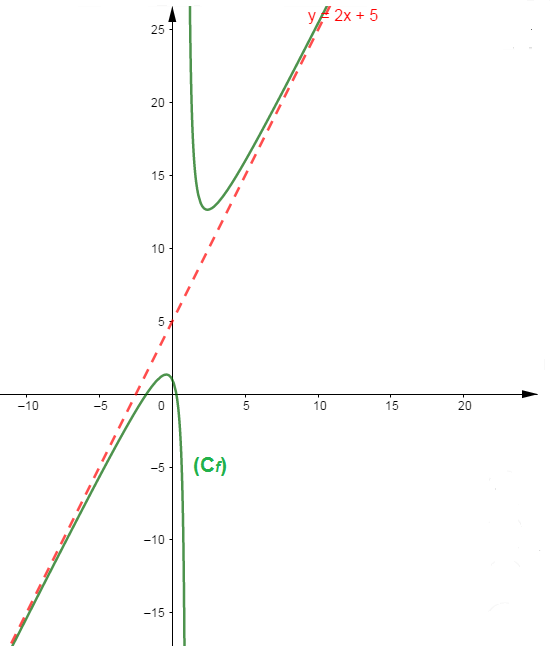
Soit f la fonction définie par f(x)=2x2+3x−1x−1
Df=R∖{1}=]−∞; 1[∪]1; +∞[ et ∀x∈Df, f(x)=2x+5+4x−1
Montrons que la droite d'équation y=2x+5 est asymptote oblique à (Cf).
On a : f(x)−y=2x+5+4x−1−(2x+5)=4x−1
limx→−∞[f(x)−y]=limx→−∞4x−1=0− et
limx→+∞[f(x)−y]=limx→+∞4x−1=0+ donc la droite d'équation y=2x+5 est asymptote oblique à (Cf) au voisinage de −∞ et de +∞.
illustration graphique
Détermination pratique de a et b
Dans l'équation y=ax+b, pour déterminer les coefficients a et b on procède comme suit :
calculer : limx→∞f(x)x=a et limx→∞f(x)−ax=b
Exemple
f(x)=2x2+3x−1x−1
limx→∞f(x)x=limx→∞2x2+3x−1x−1x=limx→∞2x2+3x−1(x−1)×1x=limx→∞2x2+3x−1x2−x=limx→∞2x2x2=2
Donc, limx→∞f(x)x=2 ⇒ a=2
Ainsi,
limx→∞f(x)−2x=limx→∞2x2+3x−1x−1−2x=limx→∞2x2+3x−1−2x2+2xx−1=limx→∞5x−1x−1=limx→∞5xx=5
Donc limx→∞f(x)−2x=5 ⇒ b=5, d'où la droite d'équation y=2x+5 est asymptote oblique à (Cf) au voisinage de ±∞.
II Continuité
II.1 Définition
Soit f : I⟶R et x0∈I
⋅ On dit que f est continue en x0 si, et seulement si, limx→x0f(x)=f(x0)
⋅ On dit que f est continue à gauche de x0 si, et seulement si, limx→x−0f(x)=f(x0)
⋅ On dit que f est continue à droite de x0 si, et seulement si, limx→x+0f(x)=f(x0)
Théorème 1
Soit f : I⟶R et x0∈I
On dit que f est continue en x0 si, et seulement si, f est continue à gauche et à droite de x0, c'est-à-dire {limx→x−0f(x)=f(x0)limx→x+0f(x)=f(x0)
Exemple
Soit f la fonction définie par f(x)={√1+x2−12xsix>02x−1six≤0
Étudier la continuité de f en 0.
Résolution
limx→0+f(x)=limx→0+√1+x2−12x=limx→0+(√1+x2−1)(√1+x2+1)2x(√1+x2+1)=limx→0+1+x2−12x(√1+x2+1)=limx→0+x22x(√1+x2+1)=limx→0+x2(√1+x2+1)=limx→0+04=0
Or, f(0)=−1=limx→0−f(x) donc, limx→0−f(x)≠limx→0+f(x)
ainsi, f n'est pas continue en 0.
Théorème 2
Soient f et g deux fonctions continues en un point x0 et α un réel ; alors les fonctions αf, (f+g) et (f×g) sont continues en x0.
Si de plus g(x0≠0 alors les fonctions 1g et fg sont aussi continues en x0.
II.2 Fonction continue sur un intervalle
Définition
Une fonction f est continue sur un intervalle I de R si elle est continue en tout point de I.
Théorème 1
Toute fonction polynôme est continue sur R
Toute fonction rationnelle est continue sur son domaine de définition.
La fonction √U est continue sur son domaine de définition.
La fonction |U| est continue sur son domaine de définition.
Théorème 2
Soient f et g deux fonctions telles que f soit continue sur un intervalle I et g continue sur un intervalle J contenant f(I) alors g∘f est continue sur I.
Prolongement par continuité d'une fonction
Soit f : R⟶Rx⟶x2−1x−1
Df=R∖{1}, 1∉Df ;
f n'est pas continue au point 1. limx→1f(x)=F.I
On a : ∀x∈R∖{1}, f(x)=(x−1)(x+1)x−1=x+1
donc, limx→1f(x)=limx→1x+1=2
Soit alors g la fonction définie par {g(x)=x+1si x≠1g(1)=2
limx→1g(x)=limx→1x+1=2 donc, limx→1g(x)=g(1)
ainsi, g est continue au point 1. D'où g est appelé le prolongement par continuité de f au point 1.
Auteur:
Diny Faye & Seyni Ndiaye

Commentaires
M Pouye (non vérifié)
lun, 01/11/2021 - 07:13
Permalien
C'est vraiment un très bon
Anonyme (non vérifié)
ven, 02/05/2021 - 13:24
Permalien
Cours très intéressants.
Anonyme (non vérifié)
ven, 02/05/2021 - 13:24
Permalien
Cours très intéressants.
Nafiu (non vérifié)
ven, 05/07/2021 - 01:28
Permalien
Les cours ne se terminent pas
Anonyme (non vérifié)
jeu, 07/22/2021 - 13:34
Permalien
Cours tres complet
Baye Kebe (non vérifié)
lun, 05/23/2022 - 01:08
Permalien
Besoin d'aide sur svt,pc et maths
Ajouter un commentaire