Solution des exercices : La cinétique chimique - Ts
Classe:
Terminale
Exercice 1
Montrons que la concentration molaire restante de l'eau oxygénée est par :
[H2O2]=C=C0−2V0VM
Équation de la réaction s'écrit :
2H2O2 → O2+2H2O
D'après l'équation de la réaction :
nH2O22=nO21⇒nH2O2=2nO2=2VO2VM
Or nH2O2restant=nH2O2initial−nH2O2dissocié⇒[H2O2]V=C0V−2VO2VM
2 Tableau
t(min)0510152030VO2(10−3L)01.562.763.654.445.26C=[H2O2]64.73.73.02.31.6(10−2mol/L)
3) Tracé de la courbe C=f(t)
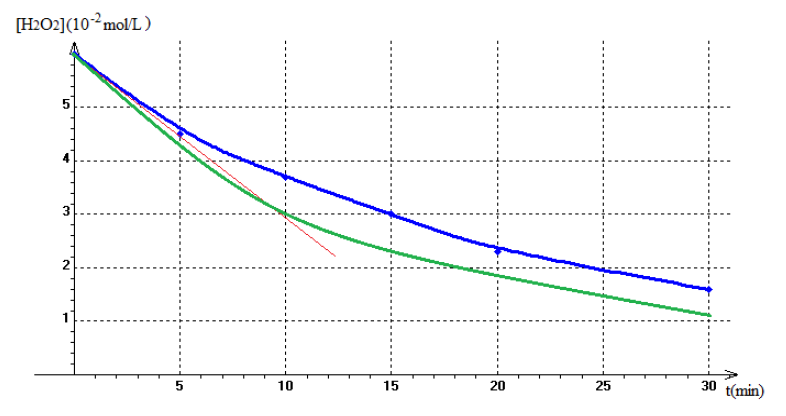
4) La vitesse instantanée de H2O2 est égale à l'opposé du coefficient directeur de la tangente à la courbe au temps considéré.
5) Tracé de la courbe pour une température supérieure à celle de la première expérience
Exercice 2
Exercice 3
1) Équation de la réaction d'oxydoréduction
Demi-équation : 2I− → I2 + 2e
électronique : S2O2−8 + 2e → 2SO2−4
Bilan : 2I− + S2O2−8 → I2 + 2SO2−4
2) a) la vitesse de la réaction à date t est égale à l'opposé du coefficient directeur de la tangente à la courbe à cette date.
b) Montrons que l'expression de la vitesse s'écrit sous la forme :
v=−V2d[I−]dt
D'après l'équation-bilan de la réaction :
nI−2=nS2O2−81−dnI−2dt=−dnS2O2−8dt=v⇒v=−dnI−2dt
Or nI−=[I−]V⇒v=−d[I−]V2dt
comme V est constant ⇒v=−V2d[I−]dt
c) Cette vitesse diminue au cours du temps car la concentration des ions iodures diminue.
d) Détermination de la valeur maximale de la vitesse
v=−[I−]6−[I−]0t6−t0=−(0−10⋅10−3)6−0⇒v=1.67⋅10−3mol⋅L−1min−1
3) a) La vitesse moyenne d'une réaction correspond à la variation de la quantité de matière (nombre de môles ou concentration) pendant de temps donnée
Expression de la vitesse moyenne :
vmoy=−Δ[I−]Δt
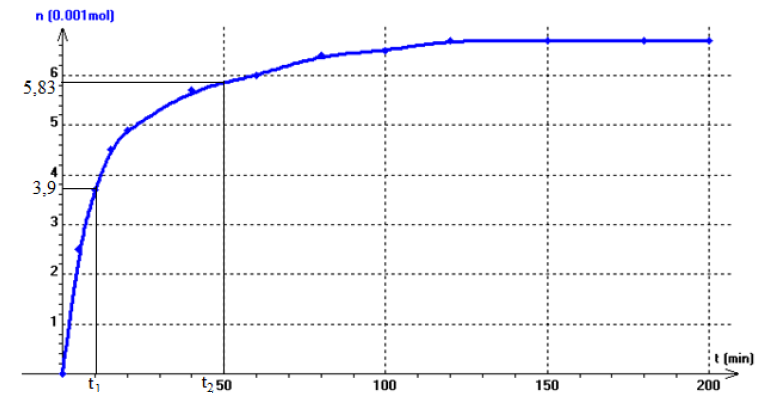
b) Calcul de la valeur de la vitesse moyenne entre les instants t1 et t2
vmoy=−(10⋅10−3−16⋅10−3)4−0⇒vmoy=1.5⋅10−3mol⋅L−1min−1
4) Tableau descriptif d'évolution du système chimique
SystèmeAvancement2I− + S2O2−8 → I2 + 2SO2−4initialx=0C1V1|C2V2|0|0intermédiairexC1V1−2x|C2V2−x|x|2xfinalxmaxC1V1−2xmax|C2V2−xmax|xmax|2xmax
b) Détermination de la quantité de matière initiale n0(I−)
n0(I−)=16⋅10−3mol
Valeur de C1
n0(I−)=C1V1⇒C1=n0(I−)V1=16⋅10−3200⋅10−3⇒C1=8⋅10−3mol⋅L−1
c)Le temps de demi-réaction est le temps au bout duquel la moitié de réactif limitant est consommée par la réaction.
Détermination de l'avancement final (maximale) de la réaction
A t1/2=4min[I−]=10⋅10−3mol⋅L−1⇒xmax=2[I−]V=2×10⋅10−3×(200+300)10−3
d'où xmax=10−2mol
d) Détermination du réactif limitant
Soient x1 et x2 les avancements maximums respectifs des réactifs
I− et S2O2−8
C1V1−2x1=0⇒x1=C1V2⇒x1=8⋅10−2×200⋅10−32⇒x1=8⋅10−3mol
C2V2−x2=0⇒x2=C2V2⇒x2=10−2×300⋅10−3⇒x2=3⋅10−3mol
x2<x1 le peroxodisulfate de potassium est le réactif limitant
Exercice 4
1) Expression de la quantité de matière de dioxygène à l'instant t en fonction de V(O2)t et de Vm
n(O2)t=V(O2)tVm
2) Tableau d'avancement de la réaction
SystèmeAvancement2H2O2(l) → 2H2O(g) + O2(g)initialx=08⋅10−2|0|0intermédiairex8⋅10−2−2x|2x|xfinalxmax8⋅10−2−xmax|2xmax|xmax
Valeur de l'avancement maximale
L'avancement est maximal lorsque 8⋅10−2−2xmax=0
⇒xmax=4⋅10−2mol
3) Complétons le tableau de mesure
x=V(O2)tVm
Pour V(O2)t=0.2L⇒x=0.224⇒x=0.83⋅10−3mol
d'où le tableau
t(min)0510152025304060V(O2)t00.20.310.400.480.540.580.650.72x(10−2mol)00.831.31.722.32.42.73
4) Tracé de la courbe x=f(t)
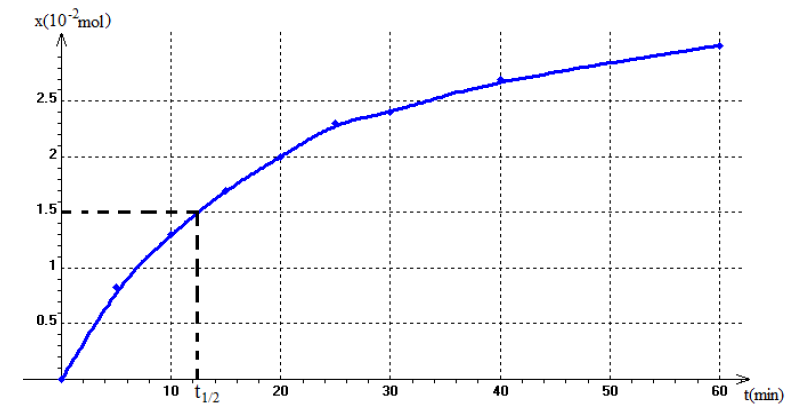
5) Vitesse moyenne de la réaction entre les t1 et t2
6) La vitesse instantanée de la réaction est égale ou coefficient directeur de la tangente à la courbe au temps considéré.
Cette vitesse de formation diminue au cours du temps (car la concentration du réactif diminue).
7) Temps de demi-réaction
t1/2=12.4min
Taux d'avancement à cette date
τ=x(t1/2)xmax=1.5⋅10−24⋅10−2⇒τ=0.375⇒τ=37.5%
Exercice 5
1) a) calcule de la concentration initiale du mélange en ions peroxodisulfate
[S2O2−8]=C1V1V1+V2=0.05×3030+30⇒[S2O2−8]=2.5⋅10−2mol⋅L−1
b) Tableau d'avancement
SystèmeAvancement2I− +S2O2−8 → I2 + 2SO2−4initialx=0C1V1|C2V2|0|0intermédiairexC1V1−2x|C2V2−x|x|2xfinalxmaxC1V1−2xmax|C2V2−xmax|xmax|2xmax
Montrons que I− est le réactif limitant
D'après l'équation de la réactif :
nI−=2nI2=2[I2]V
avec V=V1+V2
nI−2=[I2]V=15⋅10−3×(30+30)10−3=0.9⋅10−3mol
nS2O2−81=C2V2=0.05×30⋅10−3=1.5⋅10−3mol
nI−2<nS2O2−21 I− est donc le réactif limitant
c) Détermination de la concentration initiale de
nI−=2nI2=2[I2]V⇒[I−]0=nI−V=2[I2]=2×15⋅10−3⇒[I−]0=3⋅10−2mol⋅L−1
d) Détermination de la concentration C1
C1=nI−V1=1.8⋅10−330⋅10−3⇒C1=6⋅10−3mol⋅L−1
2) Détermination en mole⋅L−1, de la composition du mélange à l'instant t1=1000s
[I−]=C1V1−2xV=C1V1−2[I2]VV=6⋅10−2×30−2×2⋅10⋅10−3×6060⇒[I−]=10−2mol⋅L−1
[S2O2−8]=C2V2−xV=C2V2−[I2]VV=0.5×30−10⋅10−3×6060⇒[S2O2−8]=1.5⋅10−2mol⋅L−1
[I2]=10⋅10−3mol⋅L−1
[SO2−4]=2⋅10⋅10−3mol⋅L−1
3) a Détermination de la vitesse moyenne de la réaction
Vmoy=Δ[I2]Δt=[I2]t1−[I2]t2t2−t1=10⋅10−3−01000−0⇒Vmoy=10−5mol⋅L−1⋅s−1
4) a) La vitesse instantanée de la réaction est égale au coefficient directeur de la tangente à la courbe au temps considéré.
b) La vitesse instantanée diminue au cours du temps car la concentration ne varie pas.
c) Détermination de la concentration initiale de I−
v=(d[I2]dt)t1=[I2]t1−[I2]t2t2−t1=10⋅10−3−5⋅10−31000−0⇒v=5⋅10−6mol⋅L−1⋅s−1
5) a) Équation de la réaction :
Demi-équations : 2S2O2−3 → S4O2−6 + 2e
électronique : I2 + 2e → 2I−
bilan : 2S2O2−3 + I2 → S4O2−6 + 2I−
b) calcul de la concentration C
D'après l'équation de la réaction ;
nS2O2−3=2nI2⇒CV=2[I2]VI2⇒C=2[I2]VI2V=2×10⋅10−3×52⇒C=5⋅10−2mol⋅L−1
Exercice 6
1) Tableau d'avancement
Systèmeavancement5H2O2+2MnO−4+6H+→ 5O2+2Mn2++8H2Oinitialex=04⋅10−2|CV||0|0|excèsIntermédiairex4⋅10−2−5x|CV−2x||5x|2x|excèsfinalxmax4⋅10−2−5xmax|CV−2xmax||5xmax|2xmax|excès
L'avancement maximale correspond à 4⋅10−2−5xmax=0
⇒xmax=4⋅10−25
⇒xmax=8⋅10−3
2) a) la vitesse instantanée de disparition est à l'opposé du coefficient directeur de la tangente à la courbe à l'instant considéré
b) Détermination de la vitesse instantanée à la date t=20min
v=−dnH2O2dt=(0−3.35⋅10−2)49−0⇒v=6.8⋅10−4mol⋅L−1⋅min−1
c) La vitesse diminue au cours du temps, car la quantité de matière de l'eau oxygénée diminue
3) La vitesse moyenne est égale à la variation du nombre de moles ou de la concentration par la variation de temps
Vmoy=ΔnH2O2Δt=2⋅10−2−020−0⇒Vmoy=10−3mol⋅L−1⋅min−1
4) a) Le volume nécessaire pour le dosage
A la date t=20min, nH2O2=2⋅10−2mol
D'après l'équation bilan :
nMnO−42=nH2O25⇒CV2=nH2O25⇒V=2nH2O25C=2×2⋅10−25×0.5⇒V=16mL
b) Date à laquelle disparait 75% de la quantité initiale de H2O2
H2O2nH2O2=75%nH2O2=75×4⋅10−2100=3⋅10−2mol
Cette date correspond l'abscisse d'ordonnée nH2O2=2⋅10−2mol
⇒t=10min
Exercice 7
1) Équation-bilan de la réaction
Demi-équations : S2O2−8 + 2e → 2SO2−4
Électroniques : 2I− → I2 + 2e
Bilan : S2O2−8 + 2I− → 2SO2−4 + I2
2) a) Description de l'expérience (à faire)
On peut reconnaitre expérimentalement le point d'équivalence par la disparition de couleur bleue due à l'action de l'amidon sur le diode
b) Calcule de la concentration molaire initiale des ions iodures [I−]0 et des ions peroxodisulfate [S2O2−8]0 dans le mélange réactionnel.
[I−]0=C1V1V1+V2=16⋅10−2×5050+50⇒[I−]0=8⋅10−2mol⋅L−1
[S2O2−8]0=C2V2V1+V2=5⋅10−2×5050+50⇒[S2O2−8]0=2.5⋅10−2mol⋅L−1
c) Tableau d'avancement
SystèmeAvancement2I−+S2O2−8→ I2 + 2SO2−4initialex=0C2V2|C1V1|0|0IntermédiairexC2V2−2x|C1V1−x|x|2xfinalxmaxC2V2−2xmax|C1V1−xmax|xmax|2xmax
3) Montrons qu'à la date t[I−]t=[I−]0−2y
D'après le tableau d'avancement,
n1=C2V2−2x⇒[I−]t(V1+V2)=C2V2−2x⇒[I−]t=C2V2(V1+V2)−2x(V1+V2)
Or [I−]0=C2V2(V1+V2)
et y=x(V1+V2)
d'où [I−]t=[I−]0−2y
4) a) Précisons le réactif limitant
Soient x1 et x2 respectivement les avancements finaux des réactif S2O2−8 et I−
C1V1−x1=0⇒x1=C1V1=5⋅10−2×50⋅5⋅10−3⇒x1=2.5⋅10−3mol
C2V2−2x2=0⇒x2=C2V22=16⋅10−2×50⋅10−32⇒x2=4⋅10−3mol
x2>x1.
L'ion peroxodisulfate est le réactif limitant.
b) Déterminations de la concentration finale en ions iodures xmax=x1
[I−]f=C2V2−2x1V1+V2=C2V2−2C1V1V1+V2
Or V1=V2⇒[I−]f=C22C12=16⋅10−2−2×5⋅10−22⇒[I−]f=3⋅10−2mol⋅L−1
c) La vitesse d'une réaction chimique est à l'opposé du coefficient directeur de la tangente à la courbe au temps considéré
Montrons que s'écrit sous la forme Vvol=−12d[I−]dt
D'après l'équation de la réaction : nS2O4−81=nI−2.
En passant aux petites variation, on obtient
dnS2O4−8dt=−dnI−2dt ; puis en disant par V, il vient : dnS2O4−8Vdt=−dnI−2Vdt=vvol
Or nI−=[I−]V⇒vvol=−d[I−]V2Vdt comme V=constant⇒vvol=Vd[I−]2Vdt
⇒vvol=−12d[I−]dt
La vitesse instantanée à la date t=20min
A t=30min [I−]=[I−]f=3⋅10−2mol⋅L−1
et à t0=0min[I−]=5.5⋅10−2mol⋅L−1
v=[I−]f−[I−]t−t0=−3⋅10−2−5.5⋅10−230−0
⇒v=8.3⋅10−4mol⋅L−1⋅s−1
Exercice 8
1) Équation de la réaction d'estérification
CH3−COOH+CH3−(CH2)2−CH2OH
CH3−COO−(CH2)3−CH3+H2O
2) Tableau d'avancement
SystèmeAvancementCH3COOH+ C4H9OH→CH3COOC4H9+H2OInitialx=0a|a|0|0Intermédiairexa−x|a−x|x|xFinalxfa−xf|a−xf|xf|xf
3) a) Expression de l'avancement x en fonction de a, CB et VBE
− Équation de la réaction de dosage
CH3COH + OH− → CH3COO− + H2O
A l'équivalence :
nA=nB⇒x−a=CBVBE⇒x=a−CBVBE
b) Le taux d'avancement final
τf=xa=a−CBVBEa⇒τf=1−CBVBEa
4) a) L'état d'équilibre du système est atteint à partir de la date t=30min
b) La réaction est :
− limitée
− réalisée entre au acide carboxylique et un alcool primaire (pourcentage 67%)
c) Expression de la constant d'équilibre K en fonction de τf
k=[ester][eau][acide][alcool]=x×x(a−x)(a−x)
Or τf=xa⇒x=aτf⇒
k=aτf×aτf(a−aτf)(a−aτf)⇒k=a2τ2fa2(1−τf)(1τf)⇒k=τ2f(1−τf)2
d) Détermination, en nombre de moles, de la composition du mélange à la date t=30min
R=xa⇒x=Ra⇒nC4H9OH=a−Ra=a(1−R)=1.33⋅10−3(1−0.67)
nC4H9OH=4.4⋅10−3mol ;
nCH3COOH=nC4H9OH=4.4⋅10−3mol
nCH3COOC4H9=nH2O=x=Ra=0.67×1.33⋅10−3⇒nCH3COOC4H9=nH2O=8.9⋅10−3mol
Le volume VBE versé à la date
τf=a−CBVBEa⇒a−CBVBE=aτf⇒CBVBE=a−aτf=a(1−τf)⇒VBE=a(1−τf)CB=1.33⋅10−3(1−067)1⇒VBE=4.4mL

Commentaires
S babacar s’y diouf (non vérifié)
ven, 03/12/2021 - 00:15
Permalien
Intéressant vraiment
Anonyme (non vérifié)
mer, 01/05/2022 - 06:37
Permalien
ces exercices de cinétique
Hrizi Chakib (non vérifié)
mer, 01/05/2022 - 06:38
Permalien
ces exercices de cinétique
Anonyme (non vérifié)
lun, 02/14/2022 - 00:43
Permalien
Pour mieux comprendre le
Thierno Ibrahim... (non vérifié)
lun, 02/14/2022 - 00:47
Permalien
Demande de compréhension
Désiré (non vérifié)
sam, 12/30/2023 - 21:40
Permalien
Élève
Lamine (non vérifié)
mar, 01/23/2024 - 21:43
Permalien
Correction de l'exercice 9
Ajouter un commentaire